Question and Answers Forum

All Questions      Topic List

Arithmetic Questions

Previous in All Question      Next in All Question      

Previous in Arithmetic      Next in Arithmetic      

Question Number 128462 by Ari last updated on 07/Jan/21

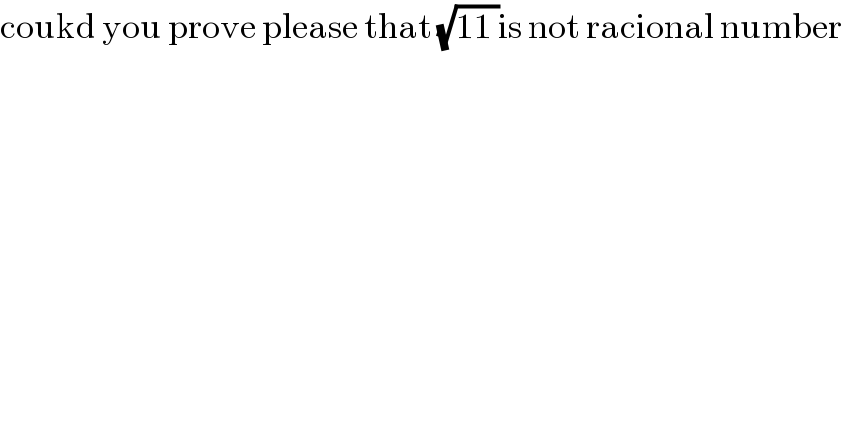
coukd you prove please that (√(11 ))is not racional number

$$\mathrm{coukd}\:\mathrm{you}\:\mathrm{prove}\:\mathrm{please}\:\mathrm{that}\:\sqrt{\mathrm{11}\:}\mathrm{is}\:\mathrm{not}\:\mathrm{racional}\:\mathrm{number} \\ $$

Answered by Dwaipayan Shikari last updated on 07/Jan/21

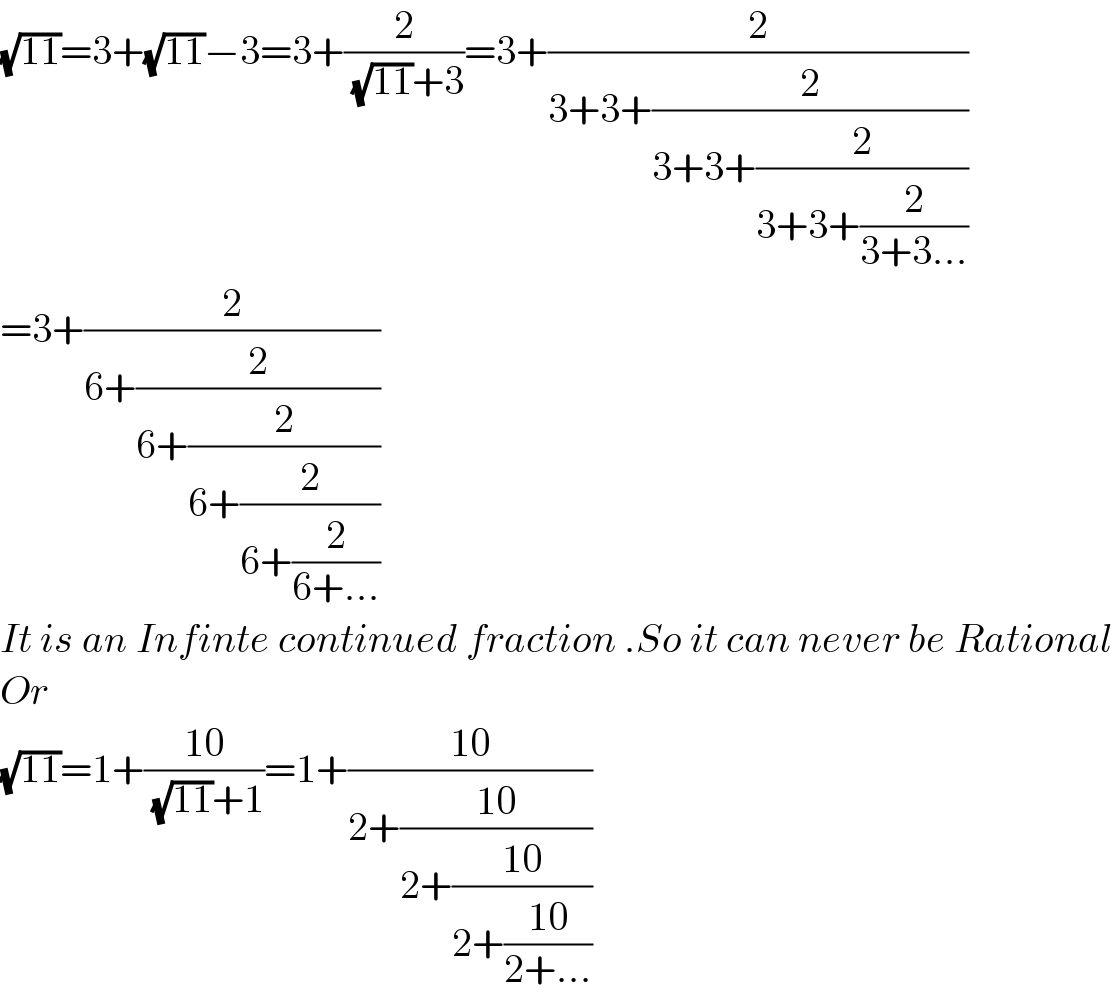
(√(11))=3+(√(11))−3=3+(2/( (√(11))+3))=3+(2/(3+3+(2/(3+3+(2/(3+3+(2/(3+3...)))))))) =3+(2/(6+(2/(6+(2/(6+(2/(6+(2/(6+...)))))))))) It is an Infinte continued fraction .So it can never be Rational Or (√(11))=1+((10)/( (√(11))+1))=1+((10)/(2+((10)/(2+((10)/(2+((10)/(2+...))))))))

$$\sqrt{\mathrm{11}}=\mathrm{3}+\sqrt{\mathrm{11}}−\mathrm{3}=\mathrm{3}+\frac{\mathrm{2}}{\:\sqrt{\mathrm{11}}+\mathrm{3}}=\mathrm{3}+\frac{\mathrm{2}}{\mathrm{3}+\mathrm{3}+\frac{\mathrm{2}}{\mathrm{3}+\mathrm{3}+\frac{\mathrm{2}}{\mathrm{3}+\mathrm{3}+\frac{\mathrm{2}}{\mathrm{3}+\mathrm{3}...}}}} \\ $$$$=\mathrm{3}+\frac{\mathrm{2}}{\mathrm{6}+\frac{\mathrm{2}}{\mathrm{6}+\frac{\mathrm{2}}{\mathrm{6}+\frac{\mathrm{2}}{\mathrm{6}+\frac{\mathrm{2}}{\mathrm{6}+...}}}}}\:\:\:\:\: \\ $$$${It}\:{is}\:{an}\:{Infinte}\:{continued}\:{fraction}\:.{So}\:{it}\:{can}\:{never}\:{be}\:{Rational} \\ $$$${Or} \\ $$$$\sqrt{\mathrm{11}}=\mathrm{1}+\frac{\mathrm{10}}{\:\sqrt{\mathrm{11}}+\mathrm{1}}=\mathrm{1}+\frac{\mathrm{10}}{\mathrm{2}+\frac{\mathrm{10}}{\mathrm{2}+\frac{\mathrm{10}}{\mathrm{2}+\frac{\mathrm{10}}{\mathrm{2}+...}}}} \\ $$

Commented by Ari last updated on 07/Jan/21

Yes,one way !

Answered by physicstutes last updated on 07/Jan/21

let′s do it by contradiction. let′s assume that (√(11)) was rational. then (√(11)) = (a/b) where a and b are in thier simpliest term. ⇒ 11 = ((a/b))^2 ⇒ a^2 = 11b^2 so we can say a = 11k (an assumption) ⇒ a^2 = 11^2 k^2 ⇒ 11b^2 = 11^2 k^2 hence b^2 = 11 k^2 so b = 11m thus gcd(a,b) = 11 which contradicts that fact that gcd(a,b) = 1 hence (√(11)) is not rational.

$$\mathrm{let}'\mathrm{s}\:\mathrm{do}\:\mathrm{it}\:\mathrm{by}\:\mathrm{contradiction}. \\ $$$$\:\mathrm{let}'\mathrm{s}\:\mathrm{assume}\:\mathrm{that}\:\sqrt{\mathrm{11}}\:\mathrm{was}\:\mathrm{rational}. \\ $$$$\mathrm{then}\:\:\sqrt{\mathrm{11}}\:=\:\frac{{a}}{{b}}\:\mathrm{where}\:{a}\:\mathrm{and}\:{b}\:\mathrm{are}\:\mathrm{in}\:\mathrm{thier}\:\mathrm{simpliest}\:\mathrm{term}. \\ $$$$\Rightarrow\:\mathrm{11}\:=\:\left(\frac{{a}}{{b}}\right)^{\mathrm{2}} \\ $$$$\Rightarrow\:{a}^{\mathrm{2}} \:=\:\mathrm{11}{b}^{\mathrm{2}} \:\:\mathrm{so}\:\mathrm{we}\:\mathrm{can}\:\mathrm{say}\:{a}\:=\:\mathrm{11}{k}\:\left(\mathrm{an}\:\mathrm{assumption}\right) \\ $$$$\Rightarrow\:{a}^{\mathrm{2}} \:=\:\mathrm{11}^{\mathrm{2}} {k}^{\mathrm{2}} \\ $$$$\Rightarrow\:\:\:\mathrm{11}{b}^{\mathrm{2}} \:=\:\mathrm{11}^{\mathrm{2}} {k}^{\mathrm{2}} \:\mathrm{hence}\:{b}^{\mathrm{2}} \:=\:\mathrm{11}\:{k}^{\mathrm{2}} \:\mathrm{so}\:{b}\:=\:\mathrm{11}{m} \\ $$$$\mathrm{thus}\:\mathrm{gcd}\left({a},{b}\right)\:=\:\mathrm{11}\:\mathrm{which}\:\mathrm{contradicts}\:\mathrm{that}\:\mathrm{fact}\:\mathrm{that}\:\mathrm{gcd}\left({a},{b}\right)\:=\:\mathrm{1} \\ $$$$\mathrm{hence}\:\sqrt{\mathrm{11}}\:\mathrm{is}\:\mathrm{not}\:\mathrm{rational}. \\ $$

Commented by Ari last updated on 07/Jan/21

Yes,you are right!

Terms of Service

Privacy Policy

Contact: info@tinkutara.com