
Question Number 128610 by john_santu last updated on 08/Jan/21
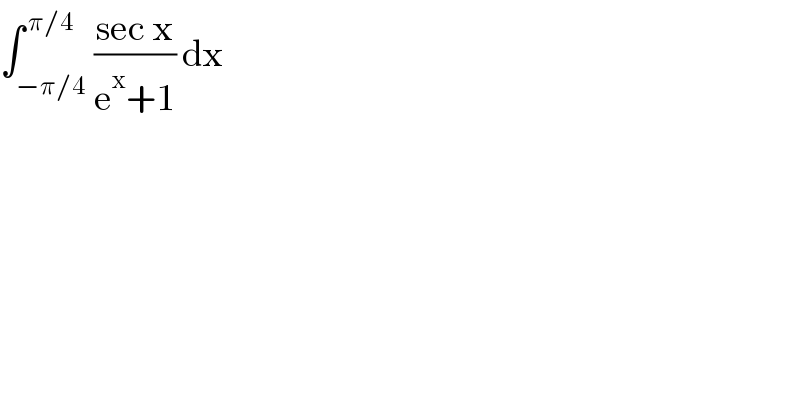
$$\int_{−\pi/\mathrm{4}} ^{\:\pi/\mathrm{4}} \frac{\mathrm{sec}\:\mathrm{x}}{\mathrm{e}^{\mathrm{x}} +\mathrm{1}}\:\mathrm{dx}\: \\ $$
Commented by liberty last updated on 09/Jan/21
![I=−∫_(−π/4) ^( π/4) ((sec (−x))/(e^(−x) +1)) d(−x) I=∫_(−π/4) ^( π/4) ((sec x)/(e^(−x) +1))dx =∫ _(−π/4)^(π/4) (((sec x)/(e^(−x) (1+e^x ))))dx I=∫_(−π/4) ^( π/4) ((e^x sec x)/(e^x +1)) dx adding together two equation 2I=∫_(−π/4) ^( π/4) ((sec x+e^x sec x)/(e^x +1)) dx =∫_(−π/4) ^( π/4) sec x dx 2I = 2∫_0 ^( π/4) sec x dx I= ln ∣sec x+tan x∣ ]_( 0) ^(π/4) = ln (1+(√2) )](Q128635.png)
$$\mathrm{I}=−\int_{−\pi/\mathrm{4}} ^{\:\pi/\mathrm{4}} \frac{\mathrm{sec}\:\left(−\mathrm{x}\right)}{\mathrm{e}^{−\mathrm{x}} +\mathrm{1}}\:\mathrm{d}\left(−\mathrm{x}\right) \\ $$$$\mathrm{I}=\int_{−\pi/\mathrm{4}} ^{\:\pi/\mathrm{4}} \frac{\mathrm{sec}\:\mathrm{x}}{\mathrm{e}^{−\mathrm{x}} +\mathrm{1}}\mathrm{dx}\:=\int\:_{−\pi/\mathrm{4}} ^{\pi/\mathrm{4}} \:\left(\frac{\mathrm{sec}\:\mathrm{x}}{\mathrm{e}^{−\mathrm{x}} \left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)}\right)\mathrm{dx} \\ $$$$\mathrm{I}=\int_{−\pi/\mathrm{4}} ^{\:\:\pi/\mathrm{4}} \:\frac{\mathrm{e}^{\mathrm{x}} \mathrm{sec}\:\mathrm{x}}{\mathrm{e}^{\mathrm{x}} +\mathrm{1}}\:\mathrm{dx}\: \\ $$$$\mathrm{adding}\:\mathrm{together}\:\mathrm{two}\:\mathrm{equation} \\ $$$$\mathrm{2I}=\int_{−\pi/\mathrm{4}} ^{\:\pi/\mathrm{4}} \frac{\mathrm{sec}\:\mathrm{x}+\mathrm{e}^{\mathrm{x}} \mathrm{sec}\:\mathrm{x}}{\mathrm{e}^{\mathrm{x}} +\mathrm{1}}\:\mathrm{dx}\:=\int_{−\pi/\mathrm{4}} ^{\:\pi/\mathrm{4}} \mathrm{sec}\:\mathrm{x}\:\mathrm{dx} \\ $$$$\mathrm{2I}\:=\:\mathrm{2}\int_{\mathrm{0}} ^{\:\pi/\mathrm{4}} \mathrm{sec}\:\mathrm{x}\:\mathrm{dx}\: \\ $$$$\left.\mathrm{I}=\:\mathrm{ln}\:\mid\mathrm{sec}\:\mathrm{x}+\mathrm{tan}\:\mathrm{x}\mid\:\right]_{\:\mathrm{0}} ^{\pi/\mathrm{4}} \:=\:\mathrm{ln}\:\left(\mathrm{1}+\sqrt{\mathrm{2}}\:\right) \\ $$$$ \\ $$
Answered by mathmax by abdo last updated on 09/Jan/21
![I=∫_(−(π/4)) ^(π/4) (1/(cosx(1+e^x )))dx =_(x=−t) ∫_(−(π/4)) ^(π/4) (1/(cost(1+e^(−t) )))dt ⇒ 2I =∫_(−(π/4)) ^(π/4) (1/(cosx))((1/(1+e^x ))+(1/(1+e^(−x) )))dx =∫_(−(π/4)) ^(π/4) (1/(cosx))(((1+e^(−x) +1+e^x )/(1+e^(−x) +e^x +1)))dx =∫_(−(π/4)) ^(π/4) (dx/(cosx)) =2∫_0 ^(π/4) (dx/(cosx)) ⇒ I =∫_0 ^(π/4) (dx/(cosx))=_(tan((x/2))=t) ∫_0 ^((√2)−1) ((2dt)/((1+t^2 ).((1−t^2 )/(1+t^2 )))) =∫_0 ^((√2)−1) ((1/(1−t))+(1/(1+t)))dt =[ln∣((1+t)/(1−t))∣]_0 ^((√2)−1) =ln∣((√2)/(2−(√2)))∣](Q128628.png)
$$\mathrm{I}=\int_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{1}}{\mathrm{cosx}\left(\mathrm{1}+\mathrm{e}^{\mathrm{x}} \right)}\mathrm{dx}\:=_{\mathrm{x}=−\mathrm{t}} \:\:\:\int_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{1}}{\mathrm{cost}\left(\mathrm{1}+\mathrm{e}^{−\mathrm{t}} \right)}\mathrm{dt}\:\Rightarrow \\ $$$$\mathrm{2I}\:=\int_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{1}}{\mathrm{cosx}}\left(\frac{\mathrm{1}}{\mathrm{1}+\mathrm{e}^{\mathrm{x}} }+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{e}^{−\mathrm{x}} }\right)\mathrm{dx}\:=\int_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{4}}} \frac{\mathrm{1}}{\mathrm{cosx}}\left(\frac{\mathrm{1}+\mathrm{e}^{−\mathrm{x}} \:+\mathrm{1}+\mathrm{e}^{\mathrm{x}} }{\mathrm{1}+\mathrm{e}^{−\mathrm{x}} \:+\mathrm{e}^{\mathrm{x}} \:+\mathrm{1}}\right)\mathrm{dx} \\ $$$$=\int_{−\frac{\pi}{\mathrm{4}}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{cosx}}\:=\mathrm{2}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{cosx}}\:\Rightarrow\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \:\frac{\mathrm{dx}}{\mathrm{cosx}}=_{\mathrm{tan}\left(\frac{\mathrm{x}}{\mathrm{2}}\right)=\mathrm{t}} \\ $$$$\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \:\frac{\mathrm{2dt}}{\left(\mathrm{1}+\mathrm{t}^{\mathrm{2}} \right).\frac{\mathrm{1}−\mathrm{t}^{\mathrm{2}} }{\mathrm{1}+\mathrm{t}^{\mathrm{2}} }}\:=\int_{\mathrm{0}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{1}−\mathrm{t}}+\frac{\mathrm{1}}{\mathrm{1}+\mathrm{t}}\right)\mathrm{dt}\:=\left[\mathrm{ln}\mid\frac{\mathrm{1}+\mathrm{t}}{\mathrm{1}−\mathrm{t}}\mid\right]_{\mathrm{0}} ^{\sqrt{\mathrm{2}}−\mathrm{1}} \\ $$$$=\mathrm{ln}\mid\frac{\sqrt{\mathrm{2}}}{\mathrm{2}−\sqrt{\mathrm{2}}}\mid \\ $$
