Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 128698 by I want to learn more last updated on 09/Jan/21

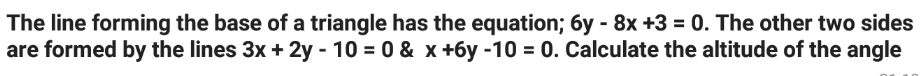
Commented by I want to learn more last updated on 09/Jan/21

$$\mathrm{Altitude}\mathrm{of}\mathrm{the}\mathrm{triangle}.$$

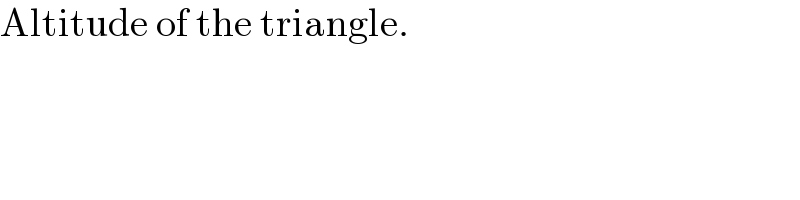
Commented by liberty last updated on 09/Jan/21

$$\mathrm{distance}\mathrm{point}(\frac{5}{2},\frac{5}{4})\mathrm{to}\mathrm{line}6\mathrm{y}-8\mathrm{x}+3=0$$$$\mathrm{the}\mathrm{altitude}=\frac{\mid \frac{30}{4}-\frac{40}{2}+3\mid }{\sqrt{36+64}}=\frac{\mid -\frac{25}{2}+\frac{6}{2}\mid }{10}=\frac{19}{20}$$

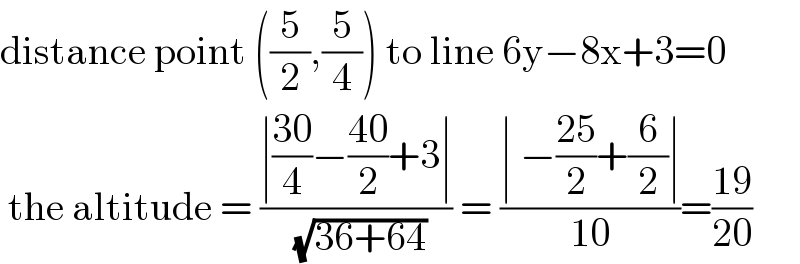
Commented by I want to learn more last updated on 10/Jan/21

$$\mathrm{Thanks}\mathrm{sir}.$$

Answered by mr W last updated on 09/Jan/21

$$intersectionof$$$$3x+2y-10=0and$$$$x+6y-10=0$$$$\Rightarrow y=\frac{5}{4}$$$$\Rightarrow x=\frac{5}{2}$$$$distancefrom(\frac{5}{2},\frac{5}{4})tobase$$$$6y-8x+3=0$$$$is$$$$h=\frac{\mid -8\times \frac{5}{2}+6\times \frac{5}{4}+3\mid }{\sqrt{{(-8)}^2+6^2}}=\frac{19}{20}$$$$whichisalsothealtitude.$$

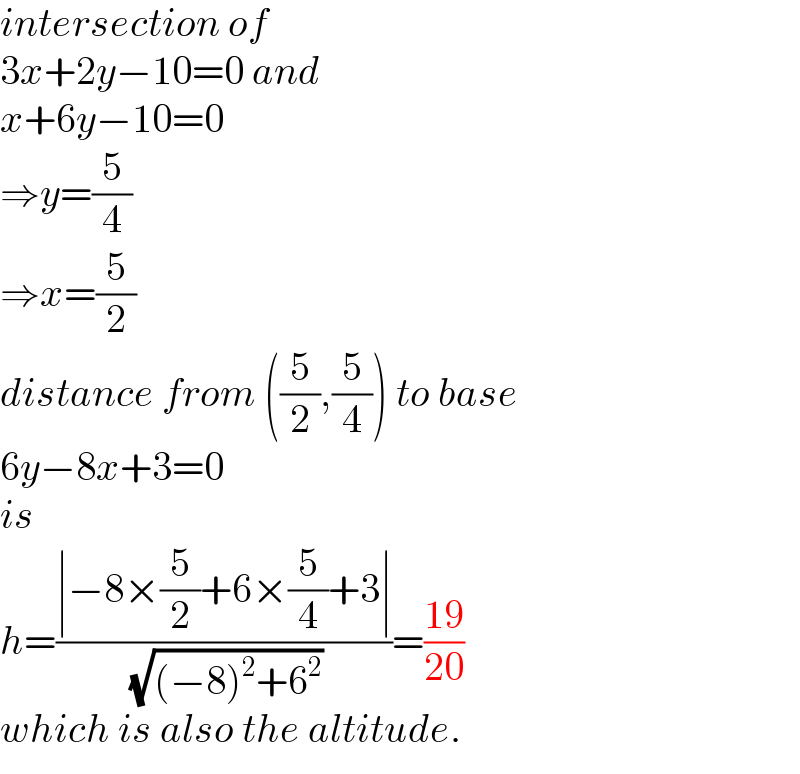
Commented by I want to learn more last updated on 10/Jan/21

$$\mathrm{Thanks}\mathrm{sir}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com