
Question Number 129446 by Eric002 last updated on 15/Jan/21
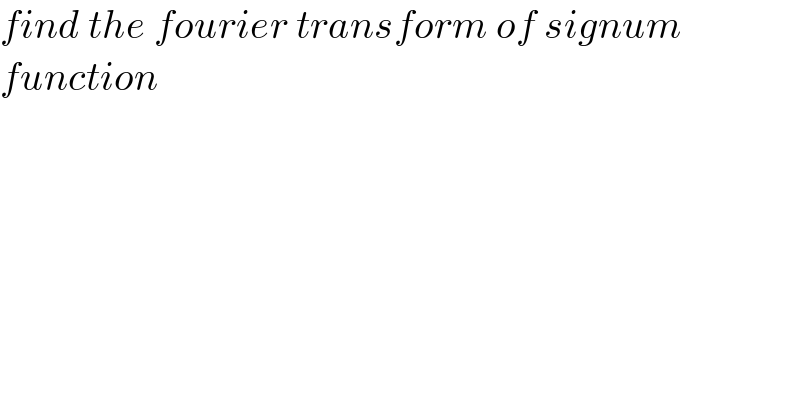
$${find}\:{the}\:{fourier}\:{transform}\:{of}\:{signum} \\ $$$${function} \\ $$
Answered by Olaf last updated on 16/Jan/21
![sgn(x) = { ((−1, x < 1)),((0, x = 0)),((+1, x > 1)) :} sgn^(�) (f) = lim_(T→∞,α→0) {∫_(−(T/2)) ^(+(T/2)) sgn_α (t)e^(−j2πft) dt} with sgn_α (t) = { ((−e^(αt) , t < 0)),((+e^(αt) , t > 0)) :} sgn^(�) (f) = lim_(T→∞,α→0) {−∫_(−(T/2)) ^(+0) e^(−jt(2πf+jα)) dt +∫_0 ^(+(T/2)) e^(−jt(2πf−jα)) dt} sgn^(�) (f) = lim_(T→∞,α→0) {(1/(−α+j2πf))+(1/(α+j2πf)) +((e^(−α(T/2)) e^(jπft) )/(α−j2πf))+((e^(−α(T/2)) e^(−j2πf) )/(α+j2πf))} Finally, by imposing αT→∞ : sgn^(�) (f) = lim_(α→0) {((j4πf)/(−α^2 −4π^2 f^2 ))} = (1/(jπf)) sgn^(�) (f) = = (1/(jπf)), f is the frequency [Hertz] or sgn^(�) (ω) = (1/( (√(2π)))).(1/(jπ(ω/(2π)))) = (√(2/π)).(1/(jω)) (ω is the pulsation [rad.s^(−1) ])](Q129600.png)
$$\mathrm{sgn}\left({x}\right)\:=\:\begin{cases}{−\mathrm{1},\:{x}\:<\:\mathrm{1}}\\{\mathrm{0},\:{x}\:=\:\mathrm{0}}\\{+\mathrm{1},\:{x}\:>\:\mathrm{1}}\end{cases}\: \\ $$$$\widehat {\mathrm{sgn}}\left({f}\right)\:=\:\underset{{T}\rightarrow\infty,\alpha\rightarrow\mathrm{0}} {\mathrm{lim}}\left\{\int_{−\frac{{T}}{\mathrm{2}}} ^{+\frac{{T}}{\mathrm{2}}} \mathrm{sgn}_{\alpha} \left({t}\right){e}^{−{j}\mathrm{2}\pi{ft}} {dt}\right\} \\ $$$$\mathrm{with}\:\mathrm{sgn}_{\alpha} \left({t}\right)\:=\:\begin{cases}{−{e}^{\alpha{t}} ,\:{t}\:<\:\mathrm{0}}\\{+{e}^{\alpha{t}} ,\:{t}\:>\:\mathrm{0}}\end{cases} \\ $$$$\widehat {\mathrm{sgn}}\left({f}\right)\:=\:\underset{{T}\rightarrow\infty,\alpha\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left\{−\int_{−\frac{{T}}{\mathrm{2}}} ^{+\mathrm{0}} {e}^{−{jt}\left(\mathrm{2}\pi{f}+{j}\alpha\right)} {dt}\right. \\ $$$$\left.+\int_{\mathrm{0}} ^{+\frac{{T}}{\mathrm{2}}} {e}^{−{jt}\left(\mathrm{2}\pi{f}−{j}\alpha\right)} {dt}\right\} \\ $$$$\widehat {\mathrm{sgn}}\left({f}\right)\:=\:\underset{{T}\rightarrow\infty,\alpha\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left\{\frac{\mathrm{1}}{−\alpha+{j}\mathrm{2}\pi{f}}+\frac{\mathrm{1}}{\alpha+{j}\mathrm{2}\pi{f}}\right. \\ $$$$\left.+\frac{{e}^{−\alpha\frac{{T}}{\mathrm{2}}} {e}^{{j}\pi{ft}} }{\alpha−{j}\mathrm{2}\pi{f}}+\frac{{e}^{−\alpha\frac{{T}}{\mathrm{2}}} {e}^{−{j}\mathrm{2}\pi{f}} }{\alpha+{j}\mathrm{2}\pi{f}}\right\} \\ $$$$\mathrm{Finally},\:\mathrm{by}\:\mathrm{imposing}\:\alpha{T}\rightarrow\infty\:: \\ $$$$\widehat {\mathrm{sgn}}\left({f}\right)\:=\:\underset{\alpha\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left\{\frac{{j}\mathrm{4}\pi{f}}{−\alpha^{\mathrm{2}} −\mathrm{4}\pi^{\mathrm{2}} {f}^{\mathrm{2}} }\right\}\:=\:\frac{\mathrm{1}}{{j}\pi{f}} \\ $$$$\widehat {\mathrm{sgn}}\left({f}\right)\:=\:=\:\frac{\mathrm{1}}{{j}\pi{f}},\:{f}\:\mathrm{is}\:\mathrm{the}\:\mathrm{frequency}\:\left[\mathrm{Hertz}\right] \\ $$$$\mathrm{or}\:\widehat {\mathrm{sgn}}\left(\omega\right)\:=\:\frac{\mathrm{1}}{\:\sqrt{\mathrm{2}\pi}}.\frac{\mathrm{1}}{{j}\pi\frac{\omega}{\mathrm{2}\pi}}\:=\:\sqrt{\frac{\mathrm{2}}{\pi}}.\frac{\mathrm{1}}{{j}\omega} \\ $$$$\left(\omega\:\mathrm{is}\:\mathrm{the}\:{pulsation}\:\left[\mathrm{rad}.\mathrm{s}^{−\mathrm{1}} \right]\right) \\ $$
Commented by Eric002 last updated on 16/Jan/21

$${well}\:{done}\:{sir} \\ $$
