
Question and Answers Forum
Question Number 129825 by 0731619177 last updated on 19/Jan/21
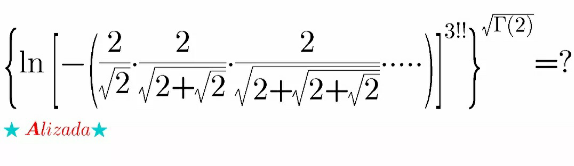
Answered by Olaf last updated on 20/Jan/21
![Let u_0 = 0 and u_n = (√(2+(√(2+(√(2...)))))), n ≥ 1 u_(n+1) = (√(2+u_n )) By induction : 0 ≤ u_n ≤ 2 ⇒ 0 ≤ u_(n+1) ≤ 2 So let u_n = 2cosθ_n (u_0 = 0 ⇒ θ_0 = (π/2)) u_(n+1) = (√(2+2cosθ_n )) = 2(√((1+cosθ_n )/2)) u_(n+1) = 2(√(cos^2 (θ_n /2))) = 2cos(θ_n /2) = 2cosθ_(n+1) ⇒ θ_(n+1) = (θ_n /2) Finally, θ_n = (θ_0 /2^n ) = (π/2^(n+1) ), u_n = 2cos(π/2^(n+1) ) sin2α = 2sinαcosα cosα = ((sin2α)/(2sinα)) ⇒ u_n = 2cosθ_n = ((sin2θ_n )/(sinθ_n )) = ((sin(π/2^n ))/(sin(π/2^(n+1) ))) Π_(n=1) ^N (2/u_n ) = 2^N Π_(n=1) ^N ((sin(π/2^(n+1) ))/(sin(π/2^n ))) = 2^N ((sin(π/2^(N+1) ))/(sin(π/2))) (telescopic product) Π_(n=1) ^N (2/u_n ) = 2^N sin(π/2^(N+1) ) ∼_∞ 2^N (π/2^(N+1) ) = (π/2) (1) 3!! = 6! = 720 (2) (√(Γ(2))) = (√(1!)) = 1 (3) With (1), (2) and (3) the result is : {ln[−((π/2))]^(720) }^1 = 720.ln(π/2)](Q129842.png)
Commented by mr W last updated on 20/Jan/21

Commented by Olaf last updated on 20/Jan/21

Commented by Olaf last updated on 20/Jan/21
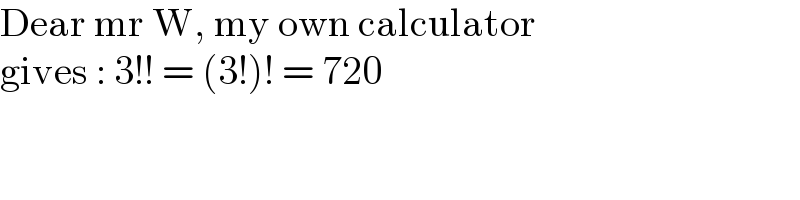
Commented by Olaf last updated on 20/Jan/21
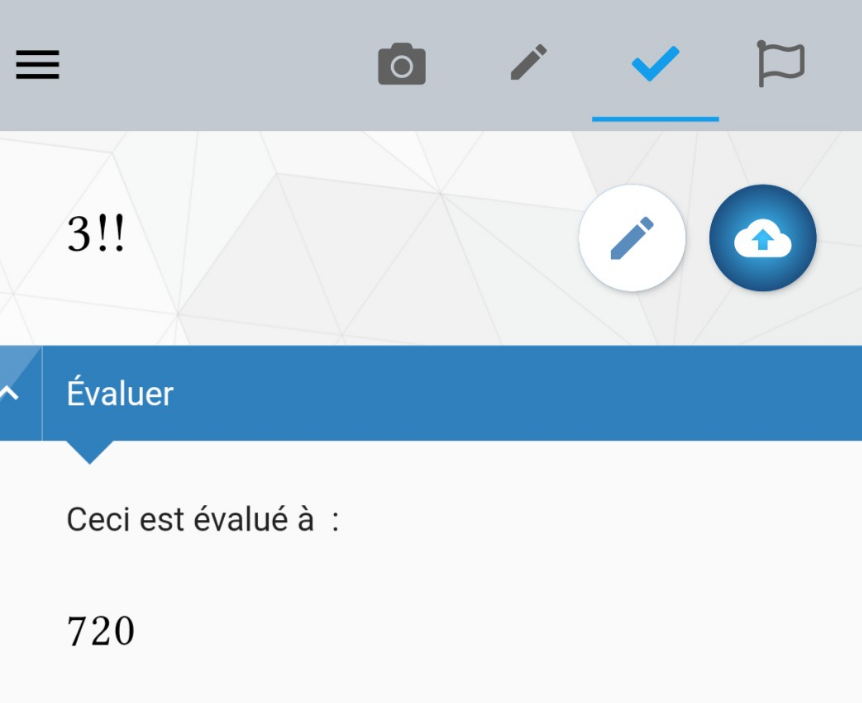
Commented by mr W last updated on 20/Jan/21

