Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 129839 by liberty last updated on 20/Jan/21

Answered by EDWIN88 last updated on 20/Jan/21

$$\mathrm{J}={\int }_0^{\pi /2}\frac{3\sqrt{\cos \mathrm{x}}}{{(\sqrt{\cos \mathrm{x}}+\sqrt{\sin \mathrm{x}})}^5}\mathrm{dx}$$$$\mathrm{let}\mathrm{x}=\frac{\pi }{2}-\mathrm{t}\Rightarrow \mathrm{J}={\int }_{\frac{\pi }{2}}^0\frac{3\sqrt{\sin \mathrm{t}}}{{(\sqrt{\sin \mathrm{t}}+\sqrt{\cos \mathrm{t}})}^5}(-\mathrm{dt})$$$$\mathrm{or}\mathrm{J}={\int }_0^{\pi /2}\frac{3\sqrt{\sin \mathrm{x}}}{{(\sqrt{\sin \mathrm{x}}+\sqrt{\cos \mathrm{x}})}^5}\mathrm{dx}$$$$\mathrm{we}\mathrm{get}2\mathrm{J}={\int }_0^{\pi /2}\frac{3(\sqrt{\sin \mathrm{x}}+\sqrt{\cos \mathrm{x}})}{{(\sqrt{\sin \mathrm{x}}+\sqrt{\cos \mathrm{x}})}^5}\mathrm{dx}$$$$2\mathrm{J}={\int }_0^{\pi /2}\frac{3\mathrm{dx}}{{\left(\sqrt{\cos \mathrm{x}}(1+\sqrt{\tan \mathrm{x}})\right)}^4}$$$$2\mathrm{J}=3{\int }_0^{\pi /2}\frac{\sec {}^2\mathrm{x}}{{(1+\sqrt{\tan \mathrm{x}})}^4}\mathrm{dx}$$$$2\mathrm{J}=3{\int }_0^{\mathrm{\infty }}\frac{\mathrm{dj}}{{(1+\sqrt{\mathrm{j}})}^4}$$$$2\mathrm{J}=3{\int }_1^{\mathrm{\infty }}({\mathrm{z}}^{-3}-{\mathrm{z}}^{-4})\mathrm{dz};\mathrm{with}\mathrm{z}=1+\sqrt{\mathrm{j}}$$$$\mathrm{J}=\frac{3}{2}\underset{\mathrm{q}\to \mathrm{\infty }}{\lim }{[-\frac{{\mathrm{z}}^{-2}}{2}+\frac{{\mathrm{z}}^{-3}}{3}]}_1^{\mathrm{q}}=\frac{1}{2}$$$$$$

Answered by Lordose last updated on 20/Jan/21

$$1.\mathrm{\Omega }={\int }_0^{\mathrm{\infty }}\frac{\cos \mathrm{x}}{\sqrt{\mathrm{x}}}\mathrm{dx}=\mathrm{Re}{\int }_0^{\mathrm{\infty }}{\mathrm{x}}^{-\frac{1}{2}}{\mathrm{e}}^{-\mathrm{ix}}\mathrm{dx}\stackrel{\mathrm{u}=\mathrm{ix}}{=}\mathrm{Re}\left(\frac{1}{\sqrt{\mathrm{i}}}{\int }_0^{\mathrm{\infty }}{\mathrm{u}}^{\frac{1}{2}-1}{\mathrm{e}}^{-\mathrm{u}}\mathrm{du}\right)$$$$\mathrm{\Omega }=\mathrm{Re}\left(\frac{1}{\sqrt{\mathrm{i}}}\mathbf{\Gamma }\left(\frac{1}{2}\right)\right)=\mathrm{Re}\left(\sqrt{\pi }\left(\frac{1-\mathrm{i}}{\sqrt{2}}\right)\right)=\sqrt{\frac{\pi }{2}}$$$$$$

Answered by Lordose last updated on 20/Jan/21

$$2.\mathrm{Im}{\int }_0^{\mathrm{\infty }}{\mathrm{x}}^{\frac{1}{2}-1}{\mathrm{e}}^{-\mathrm{ix}}\mathrm{dx}=\sqrt{\frac{\pi }{2}}$$

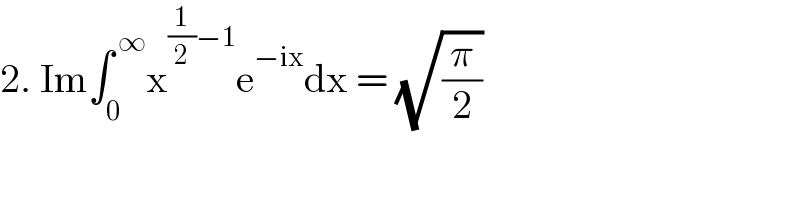
Answered by Olaf last updated on 20/Jan/21

$$\mathrm{I}={\int }_0^{\mathrm{\infty }}{e}^{-{it}^2}dt=\sqrt{\frac{\pi }{2}}.\frac{1-i}{2}$$$$\left(\mathrm{Complex}\mathrm{Fresnel}\mathrm{integral}\right)$$$$$$$$\mathrm{J}={\int }_0^{\mathrm{\infty }}\frac{\cos x}{\sqrt{x}}dx=2{\int }_0^{\mathrm{\infty }}\cos t^{2}dt(t=\sqrt{x})$$$$\mathrm{J}=2{\int }_0^{\mathrm{\infty }}\cos (-t^2)dt=2\mathrm{Re}\left(\mathrm{I}\right)=\sqrt{\frac{\pi }{2}}$$$$$$$$\mathrm{J}={\int }_0^{\mathrm{\infty }}\frac{\sin x}{\sqrt{x}}dx=2{\int }_0^{\mathrm{\infty }}\sin t^{2}dt(t=\sqrt{x})$$$$\mathrm{J}=-2{\int }_0^{\mathrm{\infty }}\sin (-t^2)dt=-2\mathrm{Im}\left(\mathrm{I}\right)=\sqrt{\frac{\pi }{2}}$$

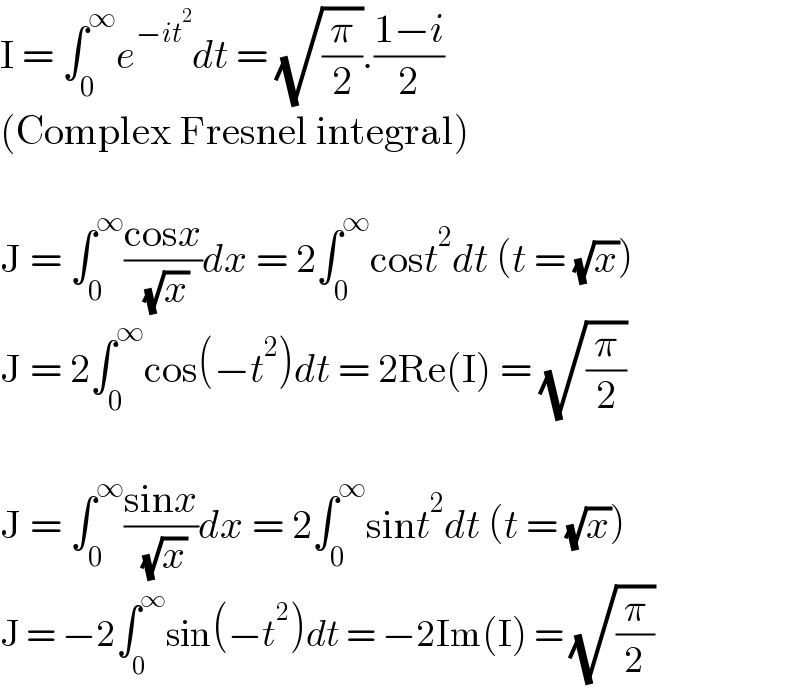
Answered by EDWIN88 last updated on 20/Jan/21

$$\mathrm{one}\mathrm{approach}\mathrm{to}\mathrm{solving}\mathrm{this}\mathrm{would}\mathrm{be}\mathrm{to}\mathrm{use}$$$$\mathrm{the}\mathrm{Gaussian}\mathrm{Integral}{\int }_0^{\mathrm{\infty }}{\mathrm{e}}^{-{\mathrm{t}}^2}\mathrm{dt}=\frac{\sqrt{\pi }}{2}$$$$\mathrm{let}\mathrm{t}=\mathrm{y}\sqrt{\mathrm{x}}\mathrm{where}\mathrm{x}\mathrm{is}\mathrm{constant}$$$$\frac{2}{\sqrt{\pi }}{\int }_0^{\mathrm{\infty }}{\mathrm{e}}^{-{\mathrm{xy}}^2}\mathrm{dy}=\frac{1}{\sqrt{\mathrm{x}}}$$$$\mathrm{I}={\int }_0^{\mathrm{\infty }}\cos \mathrm{x}\frac{2}{\sqrt{\pi }}{\int }_0^{\mathrm{\infty }}{\mathrm{e}}^{-{\mathrm{xy}}^2}\mathrm{dydx}$$$$=\frac{2}{\sqrt{\pi }}{\int }_0^{\mathrm{\infty }}{\int }_0^{\mathrm{\infty }}{\mathrm{e}}^{-{\mathrm{xy}}^2}\cos \mathrm{x}\mathrm{dx}\mathrm{dy}$$$$=\frac{2}{\sqrt{\pi }}{\int }_0^{\mathrm{\infty }}\mathcal{L}\left(\cos \mathrm{t}\right){\mid }_{\mathrm{s}={\mathrm{y}}^2}\mathrm{dy}$$$$=\frac{2}{\sqrt{\pi }}{\int }_0^{\mathrm{\infty }}\frac{{\mathrm{y}}^2}{{\mathrm{y}}^4+1}\mathrm{dy}$$$$\mathrm{similarly}\mathrm{to}\mathrm{J}={\int }_0^{\mathrm{\infty }}\frac{\sin \mathrm{x}}{\sqrt{\mathrm{x}}}\mathrm{dx}=\frac{2}{\sqrt{\pi }}{\int }_0^{\mathrm{\infty }}\frac{\mathrm{dy}}{{\mathrm{y}}^4+1}$$$$\mathrm{I}=\mathrm{J}\Rightarrow 2\mathrm{I}=\frac{2}{\sqrt{\pi }}{\int }_0^{\mathrm{\infty }}\frac{{\mathrm{y}}^2+1}{{\mathrm{y}}^4+1}\mathrm{dy}$$$$2\mathrm{I}=\frac{2}{\sqrt{\pi }}{\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}\frac{1}{{\mathrm{u}}^2+2}\mathrm{du}[\mathrm{with}\mathrm{u}=\mathrm{y}-\frac{1}{\mathrm{y}}]$$$$2\mathrm{I}=\frac{2}{\sqrt{\pi }}.\frac{1}{\sqrt{2}}\left(\arctan \left(\frac{\mathrm{u}}{\sqrt{2}}\right)\right){\mid }_{-\mathrm{\infty }}^{\mathrm{\infty }}$$$$\mathrm{I}=\sqrt{\frac{\pi }{2}}\to \{\begin{array}{l}{\int }_0^{\mathrm{\infty }}\frac{\cos \mathrm{x}}{\sqrt{\mathrm{x}}}\mathrm{dx}=\sqrt{\frac{\pi }{2}}\\ {\int }_0^{\mathrm{\infty }}\frac{\sin \mathrm{x}}{\sqrt{\mathrm{x}}}\mathrm{dx}=\sqrt{\frac{\pi }{2}}\end{array}$$

Answered by Bird last updated on 20/Jan/21

$$1an2)letI={\int }_0^{\mathrm{\infty }}\frac{cosx}{\sqrt{x}}dxand$$$$J={\int }_0^{\mathrm{\infty }}\frac{sinx}{\sqrt{x}}dx\Rightarrow I-iJ={\int }_0^{\mathrm{\infty }}\frac{e^{-ix}}{\sqrt{x}}dx$$$${=}_{\sqrt{x}=t}{\int }_0^{\mathrm{\infty }}\frac{e^{-{it}^2}}{t}\left(2t\right)dt$$$$=2{\int }_0^{\mathrm{\infty }}{e}^{-{it}^2}dt=2{\int }_0^{\mathrm{\infty }}{e}^{-{\left(\sqrt{i}t\right)}^2}dt$$$${=}_{\sqrt{i}t=z}2{\int }_0^{\mathrm{\infty }}{e}^{-z^2}\frac{dz}{\sqrt{i}}$$$$=\frac{2}{e^{\frac{i\pi }{4}}}{\int }_0^{\mathrm{\infty }}{e}^{-z^2}dz=2e^{-i\frac{\pi }{4}}\times \frac{\sqrt{\pi }}{2}$$$$=\sqrt{\pi }\{cos\left(\frac{\pi }{4}\right)-isin\left(\frac{\pi }{4}\right)\}$$$$\sqrt{\pi }\times \frac{\sqrt{2}}{2}-i\sqrt{\pi }.\frac{\sqrt{2}}{2}\Rightarrow I=\frac{\sqrt{2\pi }}{2}$$$$andJ=\frac{\sqrt{2\pi }}{2}$$

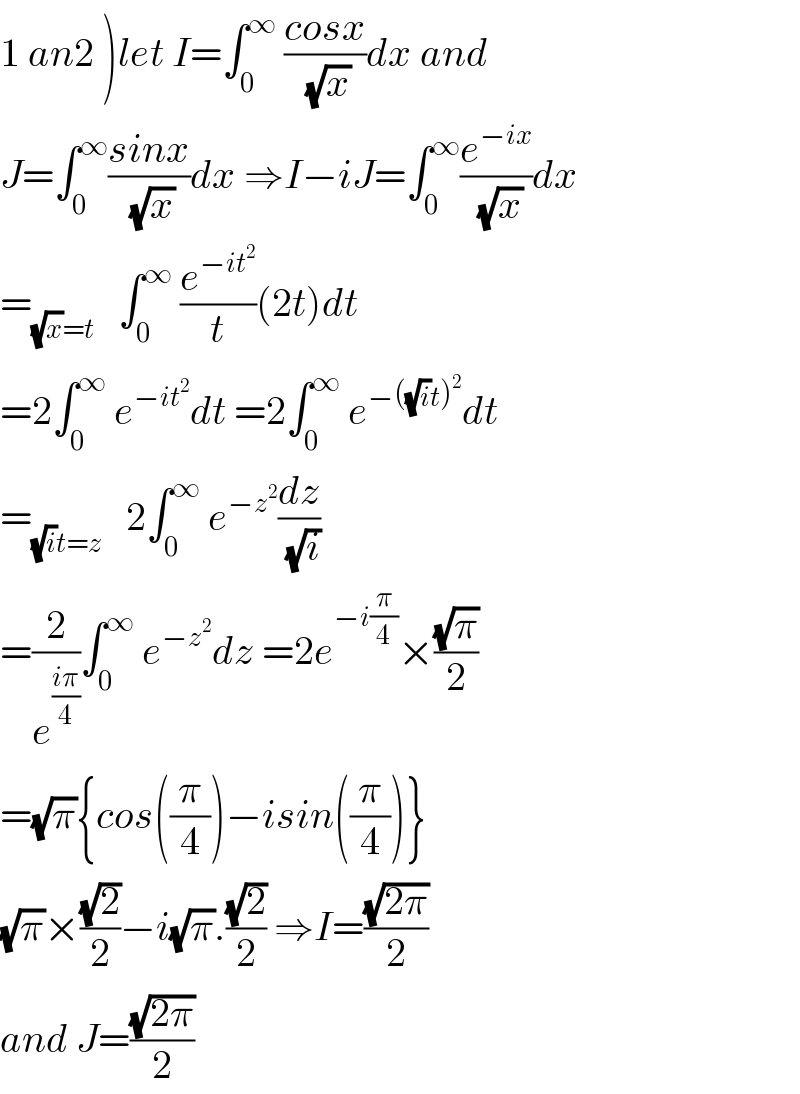
Answered by bemath last updated on 21/Jan/21

Terms of Service

Privacy Policy

Contact: info@tinkutara.com