Question and Answers Forum
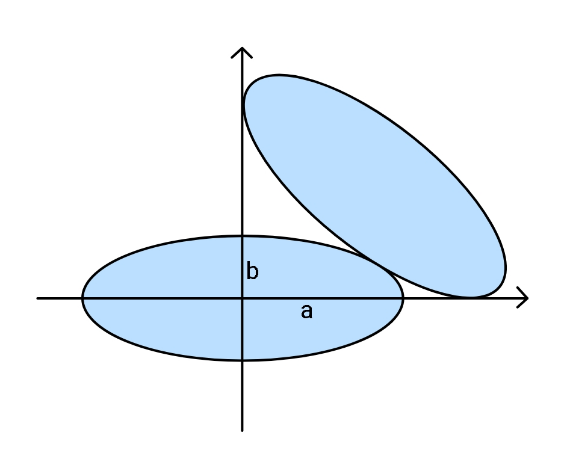
Question Number 130358 by ajfour last updated on 24/Jan/21
Commented by ajfour last updated on 24/Jan/21
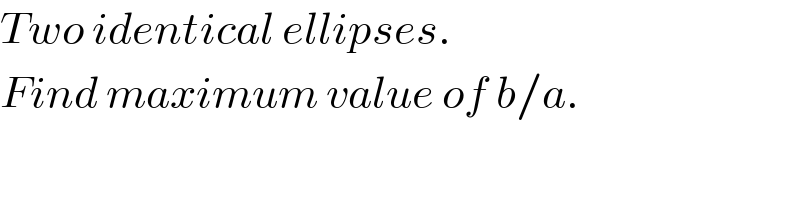
Commented by MJS_new last updated on 25/Jan/21
![once again thinking backwards (exchanging the ellipses) let a=1 (1) search 2 tangents to the 1^(st) ellipse with t_1 ⊥t_2 (2) shift and rotate the ellipse to get the 2^(nd) ellipse with center at t_1 ∩t_2 and axes t_1 and t_2 [the intersections of all possible rectangular pairs of tangents form a circle with radius (√(b^2 +1)) and center ((0),(0) ) ] I think we get the maximum b when t_1 and t_2 are symmetric to x=0 ⇔ t_1 ∩t_2 = ((0),((√(b^2 +1))) ) in this case the equation of the 2^(nd) ellipse is easy to get because the angle of rotation is (π/4). now we must intersect the ellipses and find b in order to get exactly one intersection. this leads to a 4^(th) degree polynome for x. I once posted the conditions for all possible kinds of solutions for this. it leads to a 12^(th) degree polynome for b^2 which has exactly one real solution we can only approximate. I get b≈.368 671 126 255 not sure if my idea is right... but it looks a lot to me we will get a smaller value for b when using a different pair of tangents...](Q130403.png)
Commented by MJS_new last updated on 25/Jan/21
![the equations for plotting are: ellipse 1: y=±b(√(1−x^2 )) tangents: y=±x+(√(b^2 +1)) ellipse 2: y=((1−b^2 )/(b^2 +1))x+(√(b^2 +1))±((b(√(2b^2 +2−4x^2 )))/(b^2 +1)) it seems obvious that (1) if b gets smaller the ellipses will lose contact ⇒ we have to rotate the tangents (2) if b gets larger we get 2 intersections ⇒ we have to rotate the tangents but then the space gets even smaller [this needs a proof...]](Q130404.png)
Commented by MJS_new last updated on 25/Jan/21
![above (1) obviously we rotate to the left ⇒ (2) we cannot rotate to the left with larger b but rotating to the right seems no good idea either [plot it!]](Q130405.png)
Commented by mr W last updated on 25/Jan/21

Commented by mr W last updated on 25/Jan/21

Commented by MJS_new last updated on 25/Jan/21
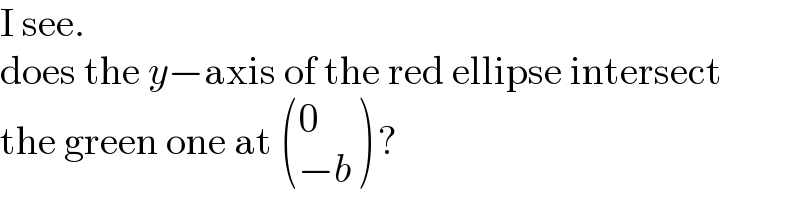
Commented by mr W last updated on 25/Jan/21