
Question and Answers Forum
Question Number 133109 by EDWIN88 last updated on 18/Feb/21
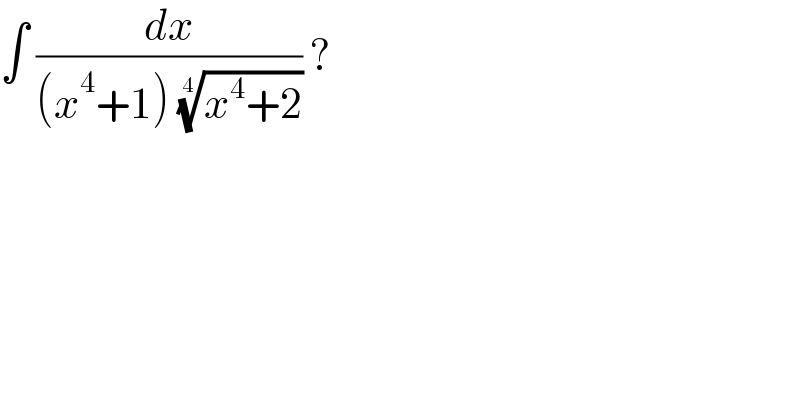
Commented by liberty last updated on 20/Feb/21
![I = ∫ (dx/((x^4 +1) ((x^4 +2))^(1/4) )) I=∫ (((1/x^5 ) dx)/((1+(1/x^4 )) ((1+(2/x^4 )))^(1/4) )) I=−(1/4)∫ ((d((1/x^4 )))/((1+(1/x^4 )) ((1+(2/x^4 )))^(1/4) )) let (1/x^4 ) = y I=−(1/4)∫ (dy/((1+y)((1+2y))^(1/4) )) let again z = ((1+2y))^(1/4) ; y=((z^4 −1)/2) I=−(1/4)∫ ((2z^3 dz)/((1+((z^4 −1)/2))z)) I=−∫ (z^2 /(z^4 +1)) dz =−∫ (1/(z^2 +(1/z^2 ))) dz I=−(1/2)∫ (((1+(1/z^2 ))+(1−(1/z^2 )))/(z^2 +(1/z^2 ))) dz I=−(1/2)[∫ ((d(z−(1/z)))/((z−(1/z))^2 +2))+ ∫ ((d(z+(1/z)))/((z+(1/z))^2 −2)) ] I=−(1/2)[ (1/( (√2))) arctan(((z−(1/z))/( (√2))))+(1/(2(√2))) ln ∣((z+(1/z)−(√2))/(z+(1/z)+(√2)))∣ + c I=−(1/(2(√2))) arctan (((z^2 −1)/(z(√2))))−(1/(4(√2)))ln ∣((z^2 −z(√2)+1)/(z^2 +z(√2)+1))∣+c I=−(1/(2(√2)))arctan ((((√(1+2y))−1)/( (√2) ((1+2y))^(1/4) )))−(1/(4(√2)))ln ∣(((√(1+2y))−(√2) ((1+2y))^(1/4) +1)/( (√(1+2y))+(√2) ((1+2y))^(1/4) +1))∣ + c I= −(1/(2(√2)))arctan (((x^2 (√(x^4 +2))−1)/(x(√2) ((x^4 +2))^(1/4) )))−(1/(4(√2)))ln ∣((x^2 (√(x^4 +2)) −x(√2) (√(x^4 +2)) +1)/(x^2 (√(x^4 +2)) +x(√2) ((x^4 +2))^(1/4) +1))∣+c](Q133235.png)
Answered by MJS_new last updated on 19/Feb/21
![∫(dx/((x^4 +1)((x^4 +2))^(1/4) ))= [t=(((x^4 +2))^(1/4) /x) ⇔ x=((2/(t^4 −1)))^(1/4) → dx=−((x^2 (((x^2 +2)^3 ))^(1/4) )/2)dt] =−∫(t^2 /(t^4 +1))dt and this should be “easy”](Q133174.png)
Answered by john_santu last updated on 19/Feb/21

| ||
Question and Answers Forum | ||
Question Number 133109 by EDWIN88 last updated on 18/Feb/21 | ||
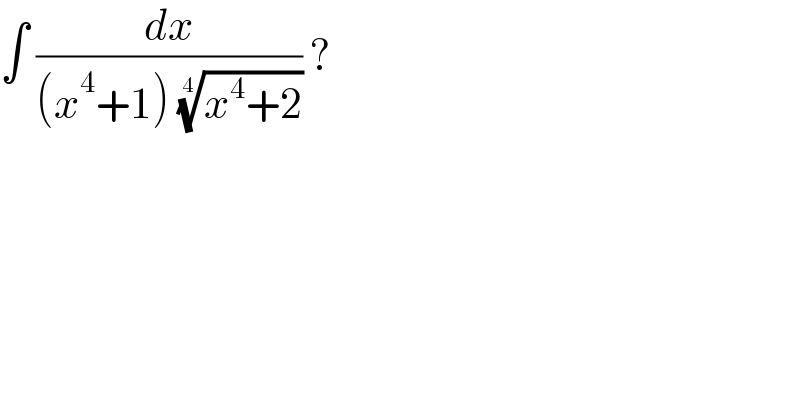 | ||
Commented by liberty last updated on 20/Feb/21 | ||
![I = ∫ (dx/((x^4 +1) ((x^4 +2))^(1/4) )) I=∫ (((1/x^5 ) dx)/((1+(1/x^4 )) ((1+(2/x^4 )))^(1/4) )) I=−(1/4)∫ ((d((1/x^4 )))/((1+(1/x^4 )) ((1+(2/x^4 )))^(1/4) )) let (1/x^4 ) = y I=−(1/4)∫ (dy/((1+y)((1+2y))^(1/4) )) let again z = ((1+2y))^(1/4) ; y=((z^4 −1)/2) I=−(1/4)∫ ((2z^3 dz)/((1+((z^4 −1)/2))z)) I=−∫ (z^2 /(z^4 +1)) dz =−∫ (1/(z^2 +(1/z^2 ))) dz I=−(1/2)∫ (((1+(1/z^2 ))+(1−(1/z^2 )))/(z^2 +(1/z^2 ))) dz I=−(1/2)[∫ ((d(z−(1/z)))/((z−(1/z))^2 +2))+ ∫ ((d(z+(1/z)))/((z+(1/z))^2 −2)) ] I=−(1/2)[ (1/( (√2))) arctan(((z−(1/z))/( (√2))))+(1/(2(√2))) ln ∣((z+(1/z)−(√2))/(z+(1/z)+(√2)))∣ + c I=−(1/(2(√2))) arctan (((z^2 −1)/(z(√2))))−(1/(4(√2)))ln ∣((z^2 −z(√2)+1)/(z^2 +z(√2)+1))∣+c I=−(1/(2(√2)))arctan ((((√(1+2y))−1)/( (√2) ((1+2y))^(1/4) )))−(1/(4(√2)))ln ∣(((√(1+2y))−(√2) ((1+2y))^(1/4) +1)/( (√(1+2y))+(√2) ((1+2y))^(1/4) +1))∣ + c I= −(1/(2(√2)))arctan (((x^2 (√(x^4 +2))−1)/(x(√2) ((x^4 +2))^(1/4) )))−(1/(4(√2)))ln ∣((x^2 (√(x^4 +2)) −x(√2) (√(x^4 +2)) +1)/(x^2 (√(x^4 +2)) +x(√2) ((x^4 +2))^(1/4) +1))∣+c](Q133235.png) | ||
Answered by MJS_new last updated on 19/Feb/21 | ||
![∫(dx/((x^4 +1)((x^4 +2))^(1/4) ))= [t=(((x^4 +2))^(1/4) /x) ⇔ x=((2/(t^4 −1)))^(1/4) → dx=−((x^2 (((x^2 +2)^3 ))^(1/4) )/2)dt] =−∫(t^2 /(t^4 +1))dt and this should be “easy”](Q133174.png) | ||
| ||
Answered by john_santu last updated on 19/Feb/21 | ||
 | ||