
Question Number 13388 by Tinkutara last updated on 19/May/17
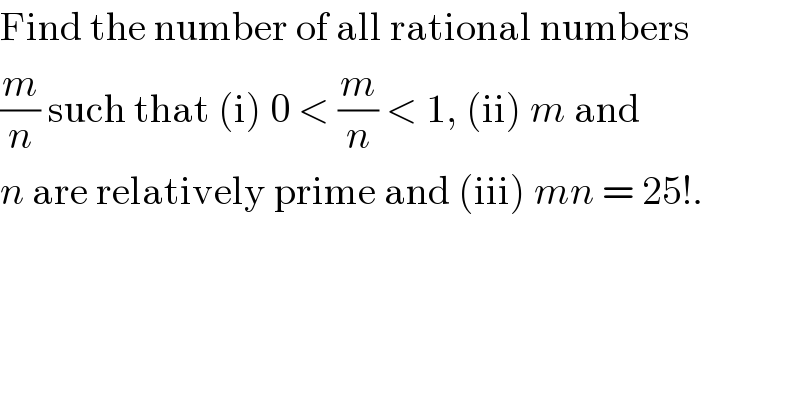
$$\mathrm{Find}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{all}\:\mathrm{rational}\:\mathrm{numbers} \\ $$ $$\frac{{m}}{{n}}\:\mathrm{such}\:\mathrm{that}\:\left(\mathrm{i}\right)\:\mathrm{0}\:<\:\frac{{m}}{{n}}\:<\:\mathrm{1},\:\left(\mathrm{ii}\right)\:{m}\:\mathrm{and} \\ $$ $${n}\:\mathrm{are}\:\mathrm{relatively}\:\mathrm{prime}\:\mathrm{and}\:\left(\mathrm{iii}\right)\:{mn}\:=\:\mathrm{25}!. \\ $$
Commented byRasheedSindhi last updated on 19/May/17
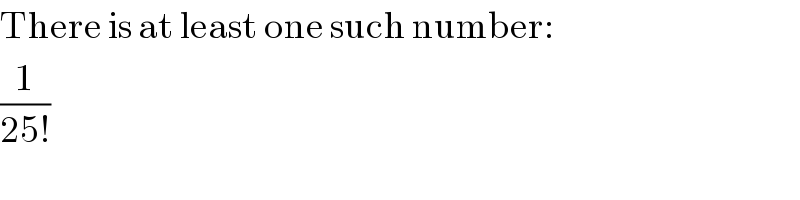
$$\mathrm{There}\:\mathrm{is}\:\mathrm{at}\:\mathrm{least}\:\mathrm{one}\:\mathrm{such}\:\mathrm{number}: \\ $$ $$\frac{\mathrm{1}}{\mathrm{25}!} \\ $$
Commented byprakash jain last updated on 19/May/17
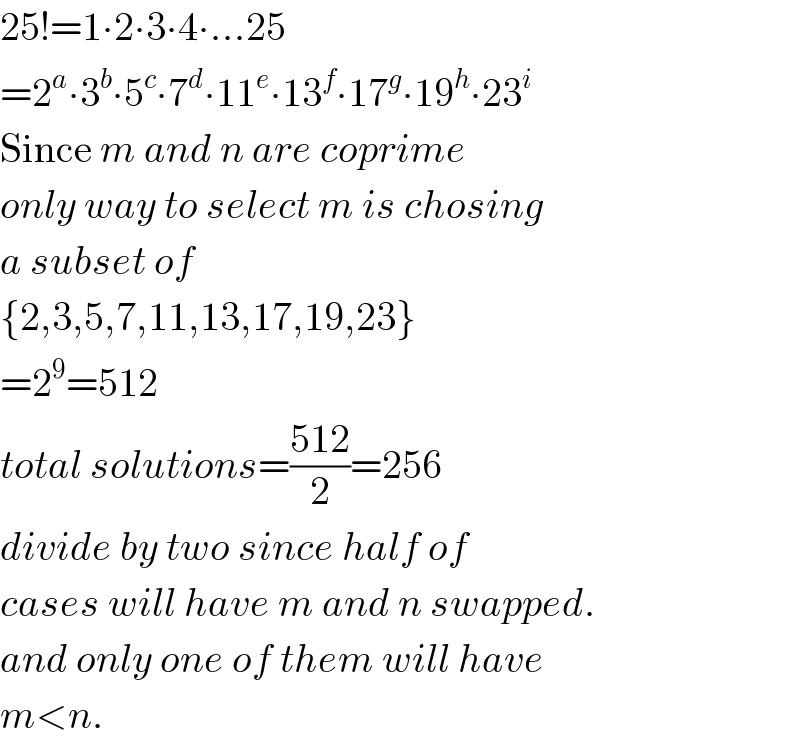
$$\mathrm{25}!=\mathrm{1}\centerdot\mathrm{2}\centerdot\mathrm{3}\centerdot\mathrm{4}\centerdot...\mathrm{25} \\ $$ $$=\mathrm{2}^{{a}} \centerdot\mathrm{3}^{{b}} \centerdot\mathrm{5}^{{c}} \centerdot\mathrm{7}^{{d}} \centerdot\mathrm{11}^{{e}} \centerdot\mathrm{13}^{{f}} \centerdot\mathrm{17}^{{g}} \centerdot\mathrm{19}^{{h}} \centerdot\mathrm{23}^{{i}} \\ $$ $$\mathrm{Since}\:{m}\:{and}\:{n}\:{are}\:{coprime} \\ $$ $${only}\:{way}\:{to}\:{select}\:{m}\:{is}\:{chosing} \\ $$ $${a}\:{subset}\:{of}\: \\ $$ $$\left\{\mathrm{2},\mathrm{3},\mathrm{5},\mathrm{7},\mathrm{11},\mathrm{13},\mathrm{17},\mathrm{19},\mathrm{23}\right\} \\ $$ $$=\mathrm{2}^{\mathrm{9}} =\mathrm{512} \\ $$ $${total}\:{solutions}=\frac{\mathrm{512}}{\mathrm{2}}=\mathrm{256} \\ $$ $${divide}\:{by}\:{two}\:{since}\:{half}\:{of} \\ $$ $${cases}\:{will}\:{have}\:{m}\:{and}\:{n}\:{swapped}. \\ $$ $${and}\:{only}\:{one}\:{of}\:{them}\:{will}\:{have} \\ $$ $${m}<{n}. \\ $$
Commented bymrW1 last updated on 20/May/17

$${how}\:{is}\:{to}\:{understand}\:{the}\:{condiction} \\ $$ $${that}\:{m}\:{and}\:{n}\:{are}\:{relatively}\:{prime}? \\ $$
Commented byprakash jain last updated on 20/May/17

$$\mathrm{Since}\:{m}\:\mathrm{and}\:{n}\:\mathrm{are}\:\mathrm{relatively}\:\mathrm{prime} \\ $$ $$\mathrm{and}\:\mathrm{distinct}\:\mathrm{prime}\:\mathrm{factor}\:\mathrm{of}\:\mathrm{25}!\:\mathrm{are} \\ $$ $$\left\{\mathrm{2},\:\mathrm{3},\:\mathrm{5},\:\mathrm{7},\:\mathrm{11},\:\mathrm{13},\:\mathrm{17},\:\mathrm{19},\:\mathrm{23}\right\} \\ $$ $$\mathrm{we}\:\mathrm{chose}\:\mathrm{of}\:\mathrm{subset}\:\mathrm{of}\:\mathrm{above}\:\mathrm{set} \\ $$ $$\mathrm{say}\:\left\{\mathrm{2},\mathrm{3},\mathrm{5}\right\} \\ $$ $${m}=\mathrm{2}^{{a}} \mathrm{3}^{{b}} \mathrm{5}^{{c}} \\ $$ $${n}=\mathrm{7}^{{d}} \mathrm{11}^{{e}} \mathrm{13}^{{f}} \mathrm{17}^{{g}} \mathrm{19}^{{h}} \mathrm{23}^{{i}} \\ $$ $$\mathrm{so}\:{m}\:\mathrm{and}\:{n}\:\mathrm{are}\:\mathrm{relative}\:\mathrm{prime}. \\ $$ $$\mathrm{but}\:\mathrm{when}\:\mathrm{we}\:\mathrm{select} \\ $$ $${m}=\mathrm{7}^{{d}} \mathrm{11}^{{e}} \mathrm{13}^{{f}} \mathrm{17}^{{g}} \mathrm{19}^{{h}} \mathrm{23}^{{i}} \\ $$ $${n}=\mathrm{2}^{{a}} \mathrm{3}^{{b}} \mathrm{5}^{{c}} \\ $$ $$\mathrm{we}\:\mathrm{discard}\:\mathrm{one}\:\mathrm{of}\:\mathrm{the}\:\mathrm{choice}\:\mathrm{so}\:\mathrm{divide}\:\mathrm{by}\:\mathrm{2}. \\ $$
Commented bymrW1 last updated on 20/May/17

$${Thanks}! \\ $$
