
Question and Answers Forum
Question Number 133897 by bemath last updated on 25/Feb/21
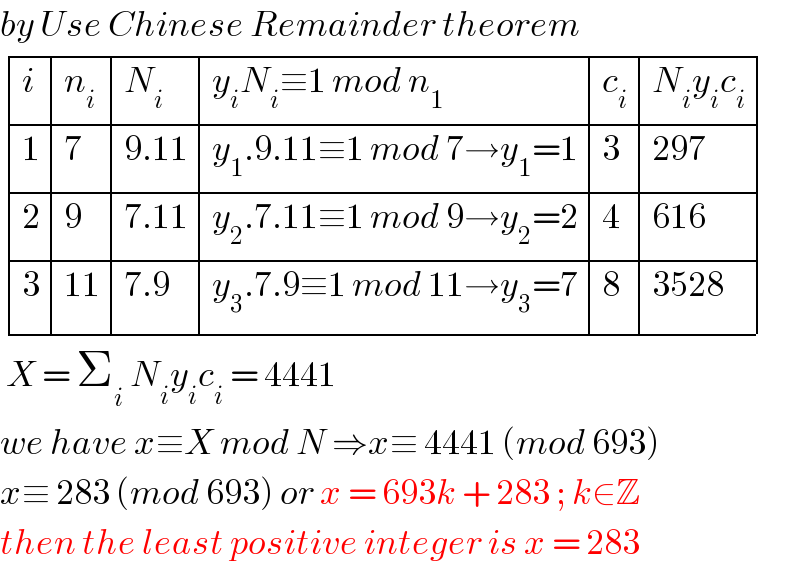
Answered by john_santu last updated on 25/Feb/21
Commented by talminator2856791 last updated on 25/Feb/21

Answered by talminator2856791 last updated on 25/Feb/21
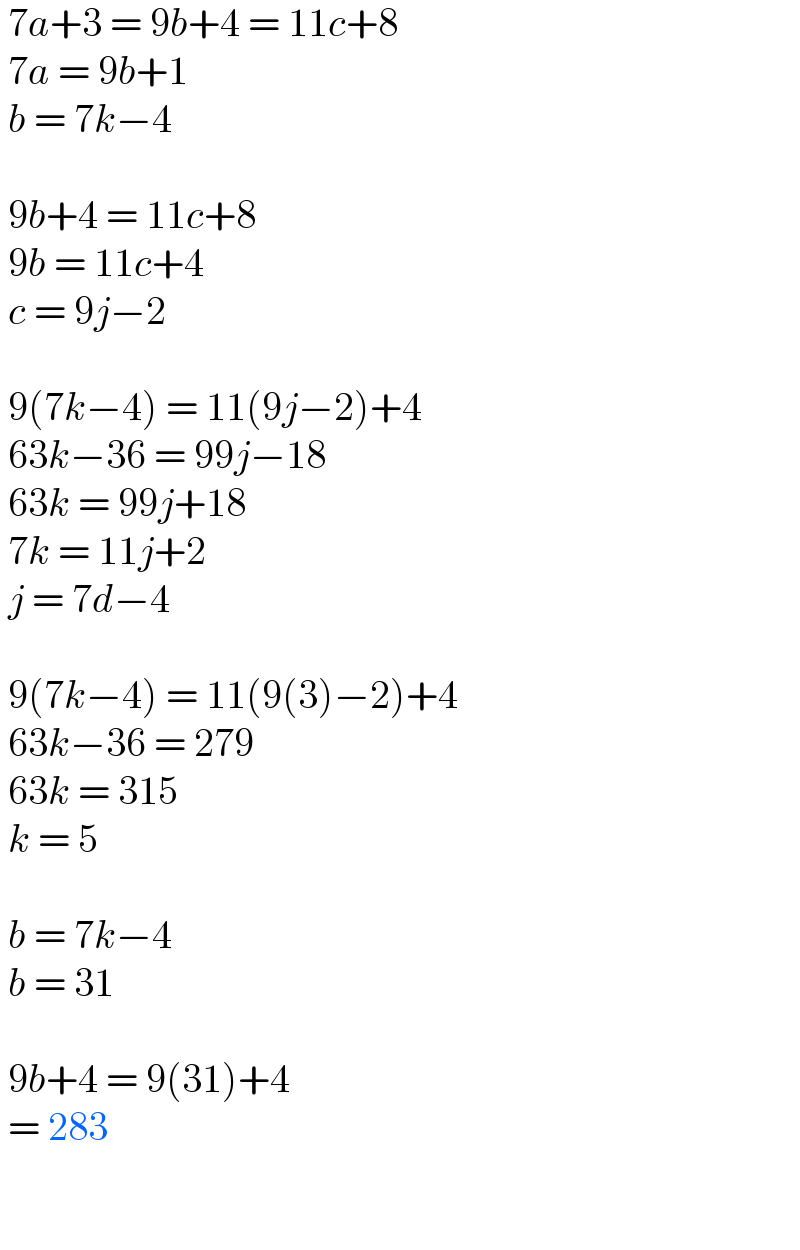
| ||
Question and Answers Forum | ||
Question Number 133897 by bemath last updated on 25/Feb/21 | ||
 | ||
Answered by john_santu last updated on 25/Feb/21 | ||
 | ||
| ||
Commented by talminator2856791 last updated on 25/Feb/21 | ||
 | ||
Answered by talminator2856791 last updated on 25/Feb/21 | ||
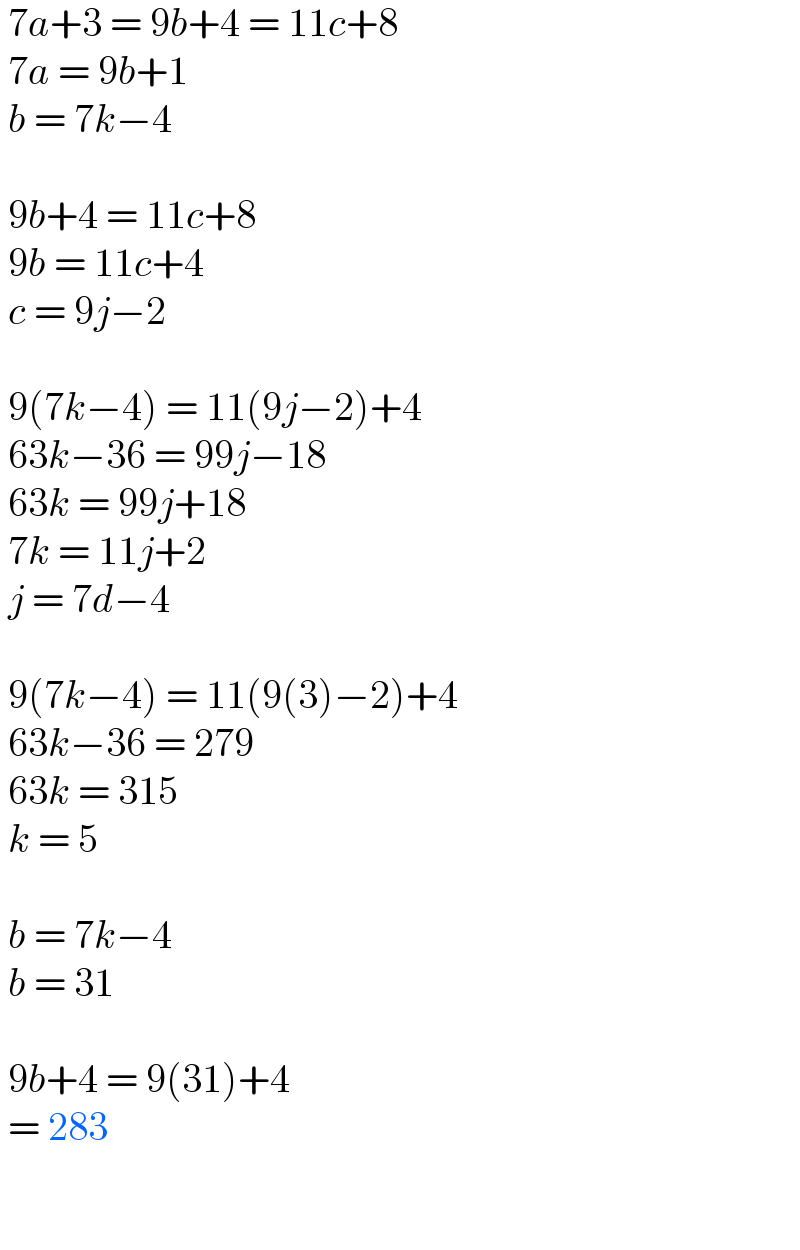 | ||
| ||