
Question and Answers Forum
Question Number 13606 by Tinkutara last updated on 21/May/17
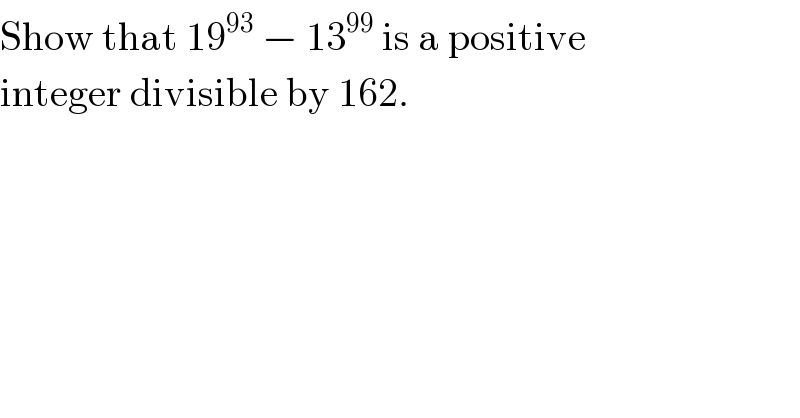
Answered by mrW1 last updated on 21/May/17

Commented by b.e.h.i.8.3.4.1.7@gmail.com last updated on 21/May/17

Commented by RasheedSindhi last updated on 22/May/17

Commented by Tinkutara last updated on 22/May/17

Commented by RasheedSindhi last updated on 22/May/17

Commented by Tinkutara last updated on 22/May/17
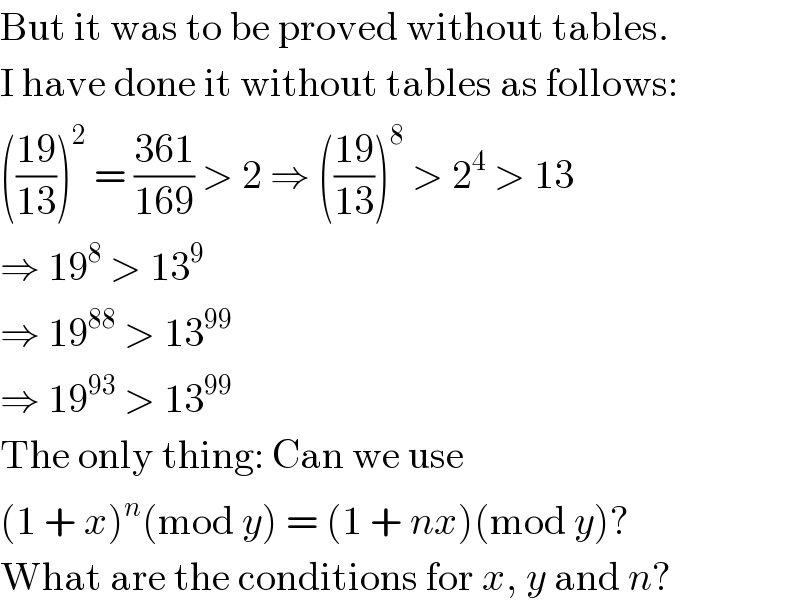
Commented by prakash jain last updated on 22/May/17

Commented by prakash jain last updated on 22/May/17

