Question and Answers Forum

All Question   Topic List

Question Number 136604 by bemath last updated on 23/Mar/21

What is the equation of a tangent to a circle whose equation is x^2+y^2−2x−4y=1 at point (1+√5,3)\n

Answered by EDWIN88 last updated on 23/Mar/21

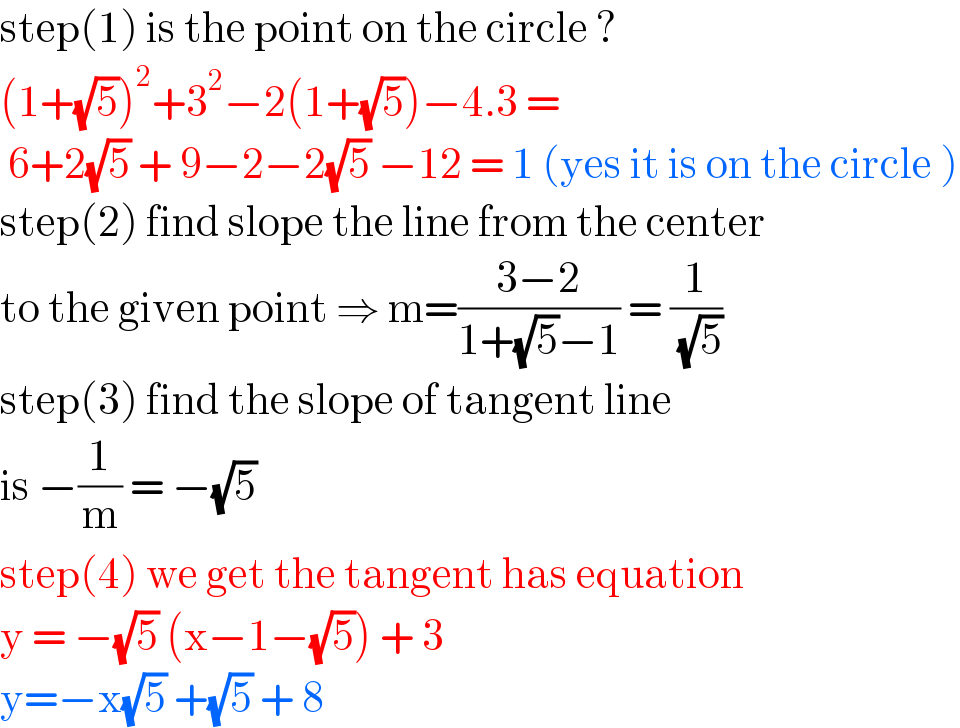
step(1) is the point on the circle ? (1+(√5))^2 +3^2 −2(1+(√5))−4.3 = 6+2(√5) + 9−2−2(√5) −12 = 1 (yes it is on the circle ) step(2) find slope the line from the center to the given point ⇒ m=((3−2)/(1+(√5)−1)) = (1/( (√5))) step(3) find the slope of tangent line is −(1/m) = −(√5) step(4) we get the tangent has equation y = −(√5) (x−1−(√5)) + 3 y=−x(√5) +(√5) + 8

step(1)isthepointonthecircle? Extra close brace or missing open brace Extra close brace or missing open brace step(2)findslopethelinefromthecenter tothegivenpoint⇒m=3−21+5−1=15 step(3)findtheslopeoftangentline is−1m=−5 Extra close brace or missing open brace Extra close brace or missing open brace y=−x5+5+8

Commented bybemath last updated on 24/Mar/21

Answered by greg_ed last updated on 24/Mar/21

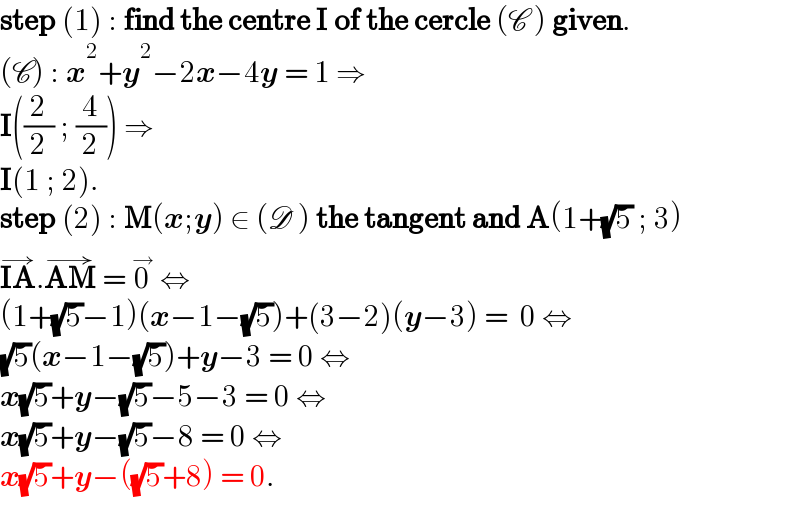
step (1) : find the centre I of the cercle (C ) given. (C) : x^2 +y^2 −2x−4y = 1 ⇒ I((2/2) ; (4/2)) ⇒ I(1 ; 2). step (2) : M(x;y) ∈ (D ) the tangent and A(1+(√5) ; 3) IA^(→) .AM^(→) = 0^→ ⇔ (1+(√5)−1)(x−1−(√5))+(3−2)(y−3) = 0 ⇔ (√5)(x−1−(√5))+y−3 = 0 ⇔ x(√5)+y−(√5)−5−3 = 0 ⇔ x(√5)+y−(√5)−8 = 0 ⇔ x(√5)+y−((√5)+8) = 0.

step(1):findthecentreIofthecercle(C)given. (C):x2+y2−2x−4y=1⇒ I(22;42)⇒ I(1;2). step(2):M(x;y)∈(D)thetangentandA(1+5;3) IA→.AM→=0→⇔ (1+5−1)(x−1−5)+(3−2)(y−3)=0⇔ 5(x−1−5)+y−3=0⇔ x5+y−5−5−3=0⇔ x5+y−5−8=0⇔ Extra close brace or missing open brace

Commented bybemath last updated on 24/Mar/21

via vector

viavector

Terms of Service

Privacy Policy

Contact: info@tinkutara.com