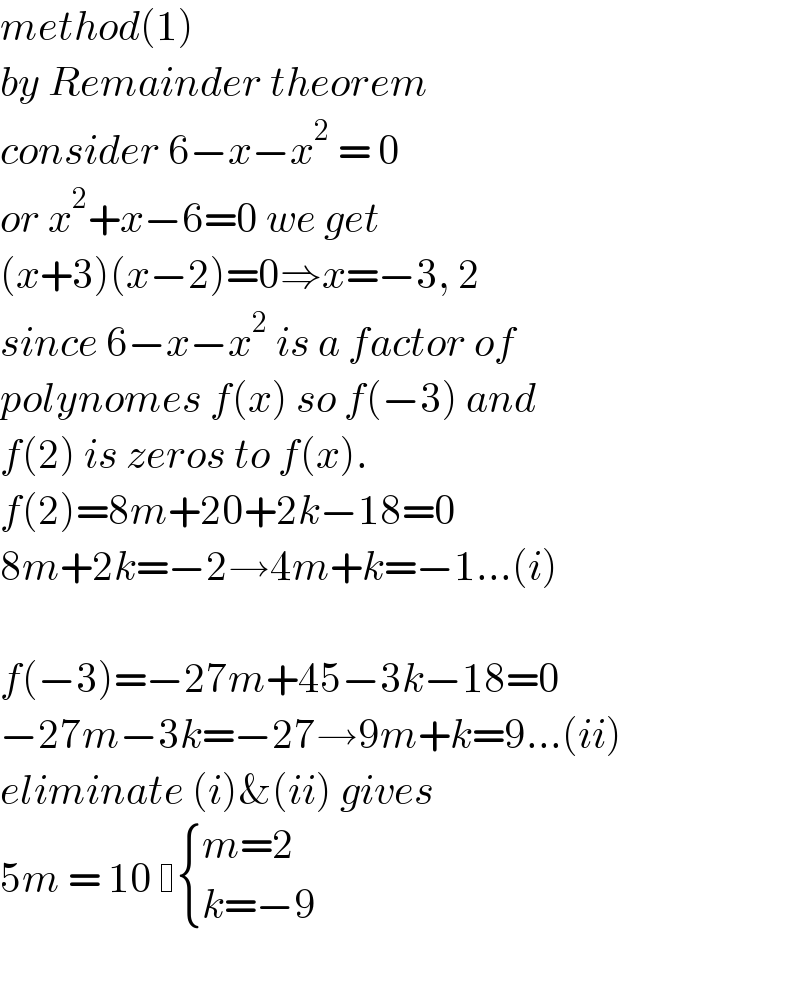Question and Answers Forum

All Questions      Topic List

Relation and Functions Questions

Previous in All Question      Next in All Question      

Previous in Relation and Functions      Next in Relation and Functions      

Question Number 136693 by liberty last updated on 25/Mar/21

Commented by Olaf last updated on 25/Mar/21

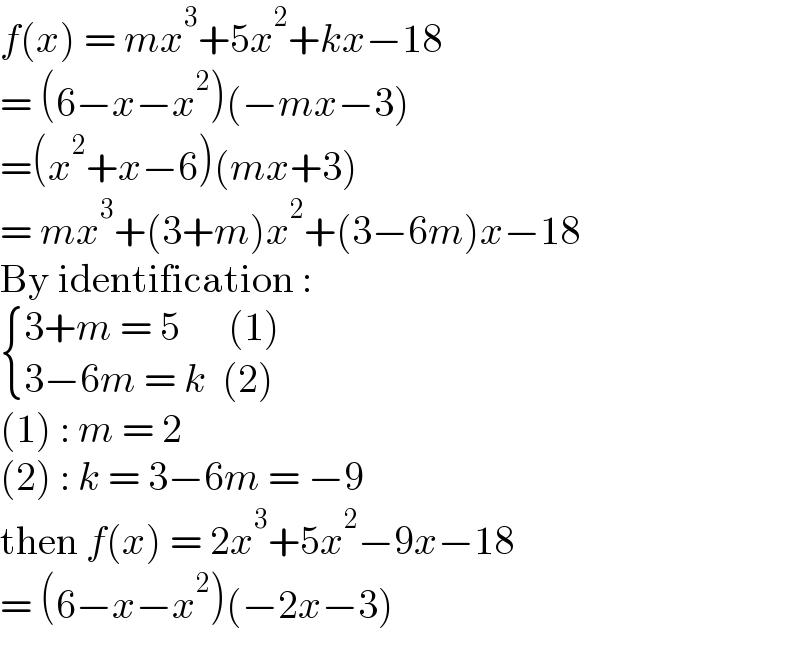
f(x) = mx^3 +5x^2 +kx−18 = (6−x−x^2 )(−mx−3) =(x^2 +x−6)(mx+3) = mx^3 +(3+m)x^2 +(3−6m)x−18 By identification : { ((3+m = 5 (1))),((3−6m = k (2))) :} (1) : m = 2 (2) : k = 3−6m = −9 then f(x) = 2x^3 +5x^2 −9x−18 = (6−x−x^2 )(−2x−3)

$${f}\left({x}\right)\:=\:{mx}^{\mathrm{3}} +\mathrm{5}{x}^{\mathrm{2}} +{kx}−\mathrm{18} \\ $$$$=\:\left(\mathrm{6}−{x}−{x}^{\mathrm{2}} \right)\left(−{mx}−\mathrm{3}\right) \\ $$$$=\left({x}^{\mathrm{2}} +{x}−\mathrm{6}\right)\left({mx}+\mathrm{3}\right) \\ $$$$=\:{mx}^{\mathrm{3}} +\left(\mathrm{3}+{m}\right){x}^{\mathrm{2}} +\left(\mathrm{3}−\mathrm{6}{m}\right){x}−\mathrm{18} \\ $$$$\mathrm{By}\:\mathrm{identification}\:: \\ $$$$\begin{cases}{\mathrm{3}+{m}\:=\:\mathrm{5}\:\:\:\:\:\:\left(\mathrm{1}\right)}\\{\mathrm{3}−\mathrm{6}{m}\:=\:{k}\:\:\left(\mathrm{2}\right)}\end{cases} \\ $$$$\left(\mathrm{1}\right)\::\:{m}\:=\:\mathrm{2} \\ $$$$\left(\mathrm{2}\right)\::\:{k}\:=\:\mathrm{3}−\mathrm{6}{m}\:=\:−\mathrm{9} \\ $$$$\mathrm{then}\:{f}\left({x}\right)\:=\:\mathrm{2}{x}^{\mathrm{3}} +\mathrm{5}{x}^{\mathrm{2}} −\mathrm{9}{x}−\mathrm{18} \\ $$$$=\:\left(\mathrm{6}−{x}−{x}^{\mathrm{2}} \right)\left(−\mathrm{2}{x}−\mathrm{3}\right) \\ $$

Answered by Rasheed.Sindhi last updated on 25/Mar/21

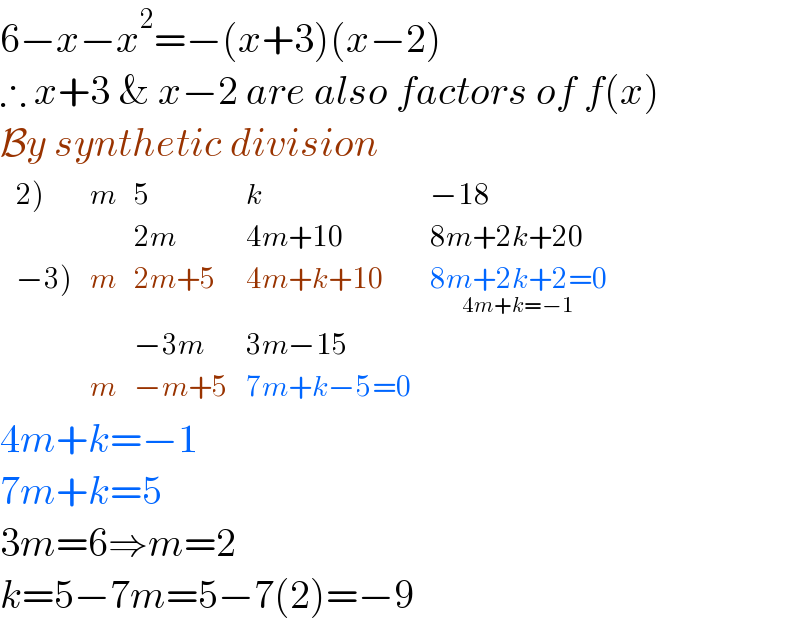
6−x−x^2 =−(x+3)(x−2) ∴ x+3 & x−2 are also factors of f(x) By synthetic division determinant (((2)),m,5,k,(−18)),(,,(2m),(4m+10),(8m+2k+20)),((−3)),m,(2m+5),(4m+k+10),(8m+2k+2=0_(4m+k=−1) )),(,,(−3m),(3m−15),),(,m,(−m+5),(7m+k−5=0),)) 4m+k=−1 7m+k=5 3m=6⇒m=2 k=5−7m=5−7(2)=−9

$$\mathrm{6}−{x}−{x}^{\mathrm{2}} =−\left({x}+\mathrm{3}\right)\left({x}−\mathrm{2}\right) \\ $$$$\therefore\:{x}+\mathrm{3}\:\&\:{x}−\mathrm{2}\:{are}\:{also}\:{factors}\:{of}\:{f}\left({x}\right) \\ $$$$\mathcal{B}{y}\:{synthetic}\:{division} \\ $$$$\begin{array}{|c|c|c|c|c|}{\left.\mathrm{2}\right)}&\hline{{m}}&\hline{\mathrm{5}}&\hline{{k}}&\hline{−\mathrm{18}}\\{}&\hline{}&\hline{\mathrm{2}{m}}&\hline{\mathrm{4}{m}+\mathrm{10}}&\hline{\mathrm{8}{m}+\mathrm{2}{k}+\mathrm{20}}\\{\left.−\mathrm{3}\right)}&\hline{{m}}&\hline{\mathrm{2}{m}+\mathrm{5}}&\hline{\mathrm{4}{m}+{k}+\mathrm{10}}&\hline{\underset{\mathrm{4}{m}+{k}=−\mathrm{1}} {\mathrm{8}{m}+\mathrm{2}{k}+\mathrm{2}=\mathrm{0}}}\\{}&\hline{}&\hline{−\mathrm{3}{m}}&\hline{\mathrm{3}{m}−\mathrm{15}}&\hline{}\\{}&\hline{{m}}&\hline{−{m}+\mathrm{5}}&\hline{\mathrm{7}{m}+{k}−\mathrm{5}=\mathrm{0}}&\hline{}\\\hline\end{array} \\ $$$$\mathrm{4}{m}+{k}=−\mathrm{1} \\ $$$$\mathrm{7}{m}+{k}=\mathrm{5} \\ $$$$\mathrm{3}{m}=\mathrm{6}\Rightarrow{m}=\mathrm{2} \\ $$$${k}=\mathrm{5}−\mathrm{7}{m}=\mathrm{5}−\mathrm{7}\left(\mathrm{2}\right)=−\mathrm{9} \\ $$

Answered by bramlexs22 last updated on 25/Mar/21

method(1) by Remainder theorem consider 6−x−x^2 = 0 or x^2 +x−6=0 we get (x+3)(x−2)=0⇒x=−3, 2 since 6−x−x^2 is a factor of polynomes f(x) so f(−3) and f(2) is zeros to f(x). f(2)=8m+20+2k−18=0 8m+2k=−2→4m+k=−1...(i) f(−3)=−27m+45−3k−18=0 −27m−3k=−27→9m+k=9...(ii) eliminate (i)&(ii) gives 5m = 10 { ((m=2)),((k=−9)) :}

$${method}\left(\mathrm{1}\right) \\ $$$${by}\:{Remainder}\:{theorem} \\ $$$${consider}\:\mathrm{6}−{x}−{x}^{\mathrm{2}} \:=\:\mathrm{0} \\ $$$${or}\:{x}^{\mathrm{2}} +{x}−\mathrm{6}=\mathrm{0}\:{we}\:{get} \\ $$$$\left({x}+\mathrm{3}\right)\left({x}−\mathrm{2}\right)=\mathrm{0}\Rightarrow{x}=−\mathrm{3},\:\mathrm{2} \\ $$$${since}\:\mathrm{6}−{x}−{x}^{\mathrm{2}} \:{is}\:{a}\:{factor}\:{of} \\ $$$${polynomes}\:{f}\left({x}\right)\:{so}\:{f}\left(−\mathrm{3}\right)\:{and} \\ $$$${f}\left(\mathrm{2}\right)\:{is}\:{zeros}\:{to}\:{f}\left({x}\right). \\ $$$${f}\left(\mathrm{2}\right)=\mathrm{8}{m}+\mathrm{20}+\mathrm{2}{k}−\mathrm{18}=\mathrm{0} \\ $$$$\mathrm{8}{m}+\mathrm{2}{k}=−\mathrm{2}\rightarrow\mathrm{4}{m}+{k}=−\mathrm{1}...\left({i}\right) \\ $$$$ \\ $$$${f}\left(−\mathrm{3}\right)=−\mathrm{27}{m}+\mathrm{45}−\mathrm{3}{k}−\mathrm{18}=\mathrm{0} \\ $$$$−\mathrm{27}{m}−\mathrm{3}{k}=−\mathrm{27}\rightarrow\mathrm{9}{m}+{k}=\mathrm{9}...\left({ii}\right) \\ $$$${eliminate}\:\left({i}\right)\&\left({ii}\right)\:{gives} \\ $$$$\mathrm{5}{m}\:=\:\mathrm{10}\: \begin{cases}{{m}=\mathrm{2}}\\{{k}=−\mathrm{9}}\end{cases} \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com