
Question and Answers Forum
Question Number 136950 by BHOOPENDRA last updated on 28/Mar/21

Answered by Olaf last updated on 28/Mar/21
![f^∧ ^c (ν) = ∫_(−∞) ^(+∞) f(s)cos(2πνs)ds = Ref^∧ (ν) F((1/s)) = −iπsign(ν) F(g(s)sin(as)) = (1/2)[g^∧ (ν−(a/(2π)))+g^∧ (ν+(a/(2π)))] F(((sin(as))/s)) = (1/2)[−iπsign(ν−(a/(2π)))−iπsign(ν+(a/(2π)))] F(((sin(as))/s)) = −((iπ)/2)[sign(ν−(a/(2π)))+sign(ν+(a/(2π)))] f^∧ ^c (ν) = Ref^∧ (ν) = 0 ????](Q136978.png)
Answered by mathmax by abdo last updated on 28/Mar/21
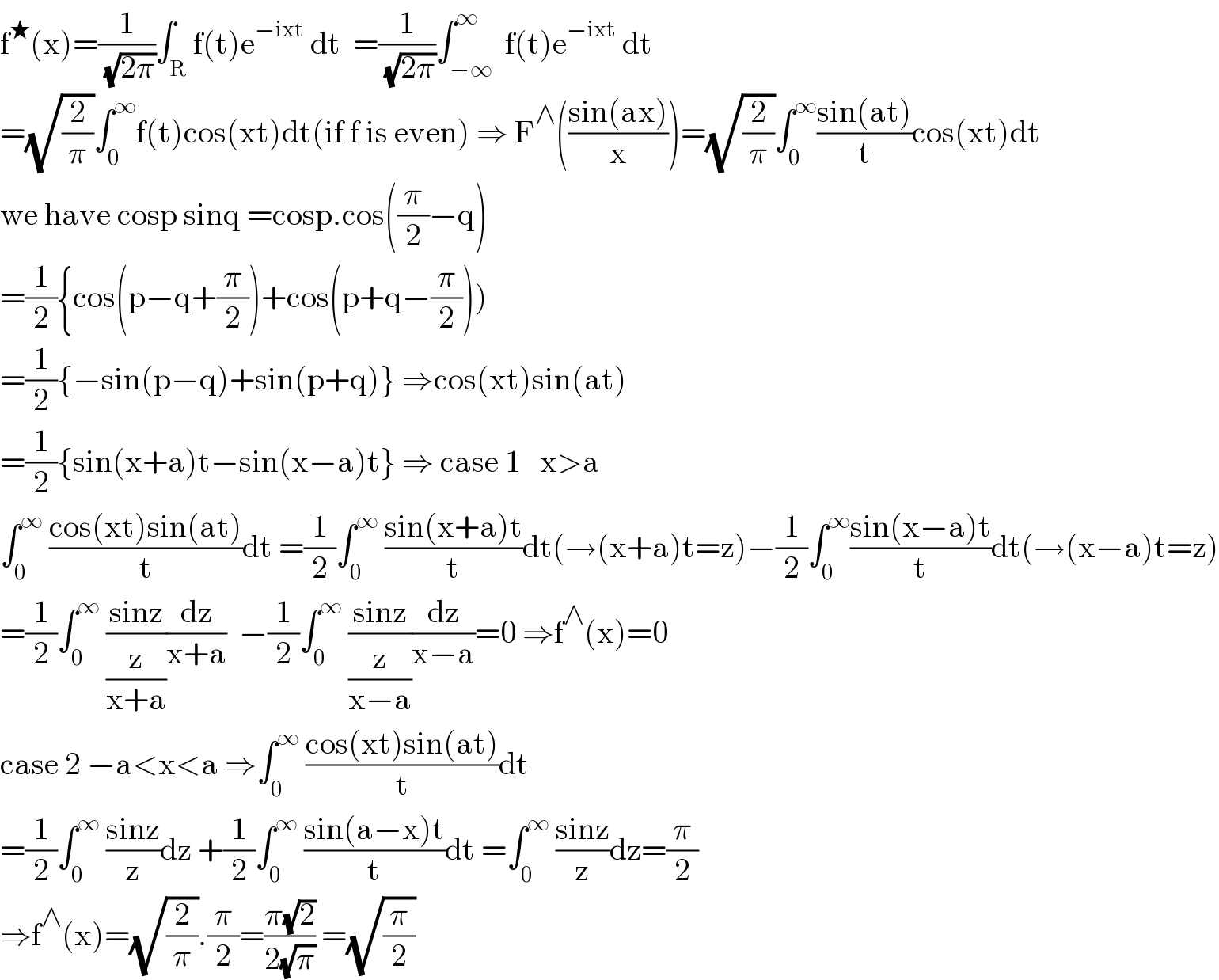
| ||
Question and Answers Forum | ||
Question Number 136950 by BHOOPENDRA last updated on 28/Mar/21 | ||
 | ||
Answered by Olaf last updated on 28/Mar/21 | ||
![f^∧ ^c (ν) = ∫_(−∞) ^(+∞) f(s)cos(2πνs)ds = Ref^∧ (ν) F((1/s)) = −iπsign(ν) F(g(s)sin(as)) = (1/2)[g^∧ (ν−(a/(2π)))+g^∧ (ν+(a/(2π)))] F(((sin(as))/s)) = (1/2)[−iπsign(ν−(a/(2π)))−iπsign(ν+(a/(2π)))] F(((sin(as))/s)) = −((iπ)/2)[sign(ν−(a/(2π)))+sign(ν+(a/(2π)))] f^∧ ^c (ν) = Ref^∧ (ν) = 0 ????](Q136978.png) | ||
| ||
Answered by mathmax by abdo last updated on 28/Mar/21 | ||
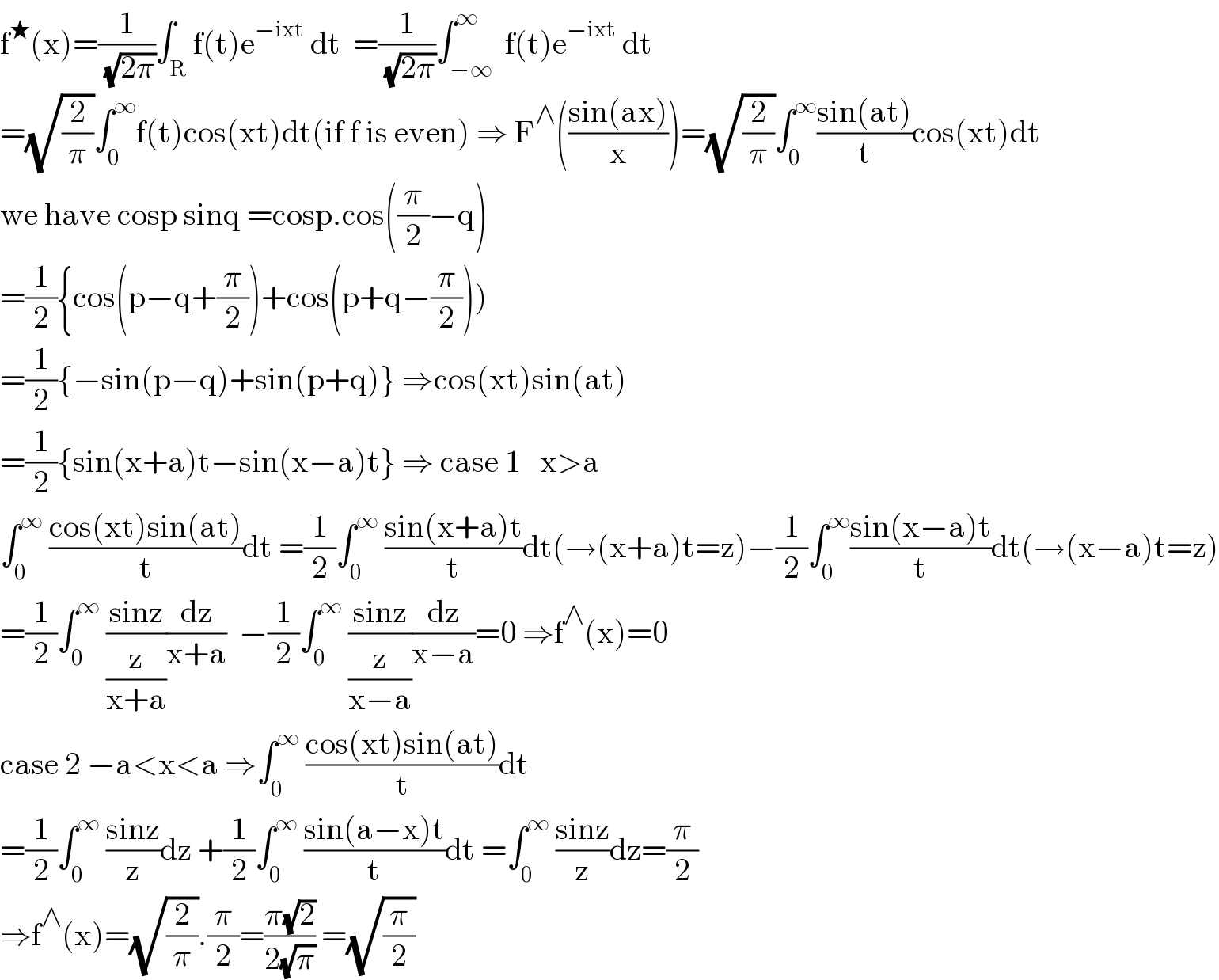 | ||
| ||