Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 1379 by 314159 last updated on 27/Jul/15

Find all positive integers n such that n≤2014 and 3^(n−1) .n will be a perfect square integer.

$${Find}\:{all}\:{positive}\:{integers}\:{n}\:{such}\:{that}\:\: \\ $$$${n}\leqslant\mathrm{2014}\:{and}\:\mathrm{3}^{{n}−\mathrm{1}} .{n}\:\:{will}\:{be}\:{a}\:{perfect}\:{square}\:{integer}. \\ $$

Commented by 123456 last updated on 26/Jul/15

m≡0,1,2,3,4(mod 5) n=m^2 ≡0,1,4(mod 5) n−1≡0(mod 2) n≡1(mod 2) N.C: { ((n≡0(mod 5)∨n≡1(mod 5)∨n≡4(mod5))),((n≡1(mod 2))) :}

$${m}\equiv\mathrm{0},\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{4}\left(\mathrm{mod}\:\mathrm{5}\right) \\ $$$${n}={m}^{\mathrm{2}} \equiv\mathrm{0},\mathrm{1},\mathrm{4}\left(\mathrm{mod}\:\mathrm{5}\right) \\ $$$${n}−\mathrm{1}\equiv\mathrm{0}\left(\mathrm{mod}\:\mathrm{2}\right) \\ $$$${n}\equiv\mathrm{1}\left(\mathrm{mod}\:\mathrm{2}\right) \\ $$$$\mathrm{N}.\mathrm{C}:\begin{cases}{{n}\equiv\mathrm{0}\left(\mathrm{mod}\:\mathrm{5}\right)\vee{n}\equiv\mathrm{1}\left(\mathrm{mod}\:\mathrm{5}\right)\vee{n}\equiv\mathrm{4}\left(\mathrm{mod5}\right)}\\{{n}\equiv\mathrm{1}\left(\mathrm{mod}\:\mathrm{2}\right)}\end{cases} \\ $$

Commented by 314159 last updated on 27/Jul/15

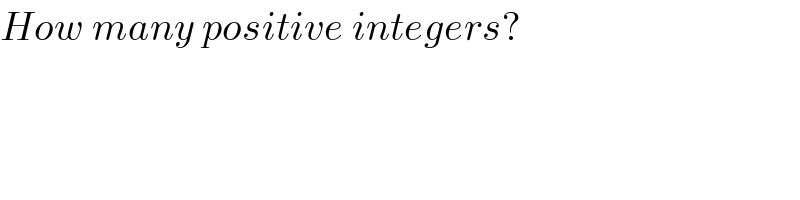
How many positive integers?

$${How}\:{many}\:{positive}\:{integers}? \\ $$

Commented by prakash jain last updated on 27/Jul/15

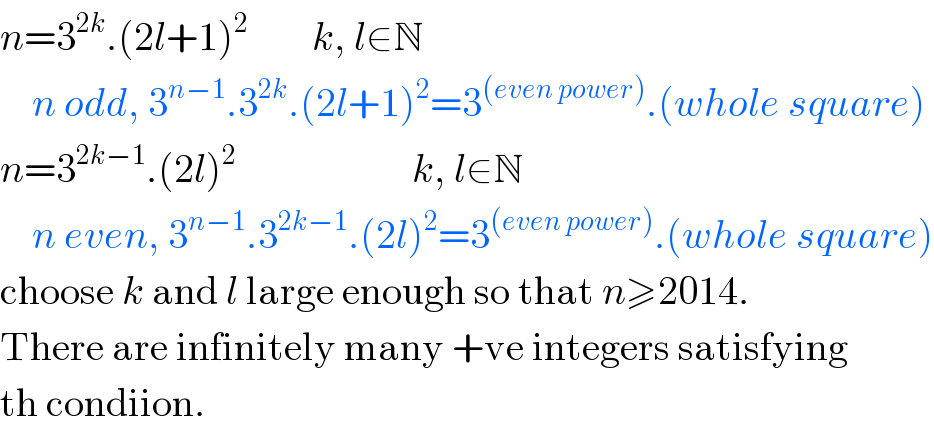
n=3^(2k) .(2l+1)^2 k, l∈N n odd, 3^(n−1) .3^(2k) .(2l+1)^2 =3^((even power)) .(whole square) n=3^(2k−1) .(2l)^2 k, l∈N n even, 3^(n−1) .3^(2k−1) .(2l)^2 =3^((even power)) .(whole square) choose k and l large enough so that n≥2014. There are infinitely many +ve integers satisfying th condiion.

$${n}=\mathrm{3}^{\mathrm{2}{k}} .\left(\mathrm{2}{l}+\mathrm{1}\right)^{\mathrm{2}} \:\:\:\:\:\:\:\:{k},\:{l}\in\mathbb{N} \\ $$$$\:\:\:\:{n}\:{odd},\:\mathrm{3}^{{n}−\mathrm{1}} .\mathrm{3}^{\mathrm{2}{k}} .\left(\mathrm{2}{l}+\mathrm{1}\right)^{\mathrm{2}} =\mathrm{3}^{\left({even}\:{power}\right)} .\left({whole}\:{square}\right) \\ $$$${n}=\mathrm{3}^{\mathrm{2}{k}−\mathrm{1}} .\left(\mathrm{2}{l}\right)^{\mathrm{2}} \:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{k},\:{l}\in\mathbb{N} \\ $$$$\:\:\:\:{n}\:{even},\:\mathrm{3}^{{n}−\mathrm{1}} .\mathrm{3}^{\mathrm{2}{k}−\mathrm{1}} .\left(\mathrm{2}{l}\right)^{\mathrm{2}} =\mathrm{3}^{\left({even}\:{power}\right)} .\left({whole}\:{square}\right) \\ $$$$\mathrm{choose}\:{k}\:\mathrm{and}\:{l}\:\mathrm{large}\:\mathrm{enough}\:\mathrm{so}\:\mathrm{that}\:{n}\geqslant\mathrm{2014}. \\ $$$$\mathrm{There}\:\mathrm{are}\:\mathrm{infinitely}\:\mathrm{many}\:+\mathrm{ve}\:\mathrm{integers}\:\mathrm{satisfying} \\ $$$$\mathrm{th}\:\mathrm{condiion}. \\ $$

Commented by 314159 last updated on 27/Jul/15

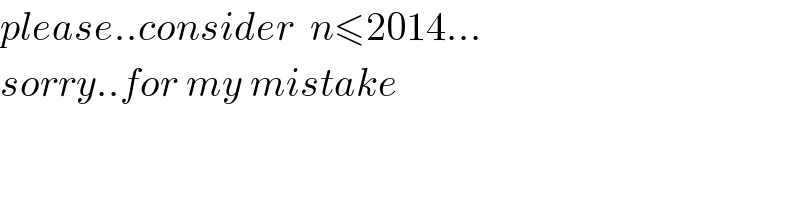
please..consider n≤2014... sorry..for my mistake

$${please}..{consider}\:\:{n}\leqslant\mathrm{2014}... \\ $$$${sorry}..{for}\:{my}\:{mistake} \\ $$

Commented by 314159 last updated on 28/Jul/15

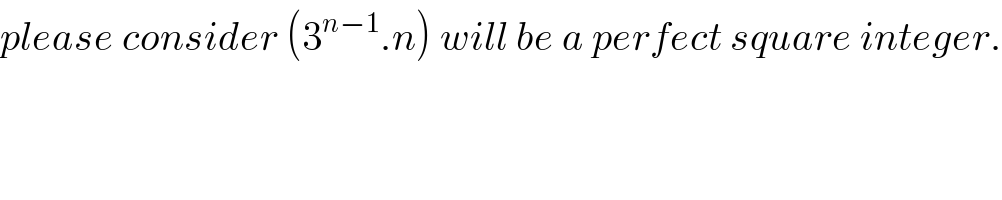
please consider (3^(n−1) .n) will be a perfect square integer.

$${please}\:{consider}\:\left(\mathrm{3}^{{n}−\mathrm{1}} .{n}\right)\:{will}\:{be}\:{a}\:{perfect}\:{square}\:{integer}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com