
Question and Answers Forum
Question Number 139811 by mnjuly1970 last updated on 01/May/21

Answered by mathmax by abdo last updated on 01/May/21
![Φ=∫_0 ^1 (√x)log((1/(1−x)))dx ⇒Φ=−∫_0 ^1 (√x)log(1−x)dx =_((√x)=t) −∫_0 ^1 t log(1−t^2 )(2t)dt =−2∫_0 ^1 t^2 log(1−t^2 )dt =−2{ [((t^3 −1)/3)log(1−t^2 )]_0 ^1 −∫_0 ^1 ((t^3 −1)/3)×((−2t)/(1−t^2 ))dt} =−(4/3) ∫_0 ^1 ((t^4 −t)/(1−t^2 ))dt =(4/3)∫_0 ^1 ((t(t^3 −1))/((t−1)(t+1)))dt =(4/3) ∫_0 ^1 ((t(t^2 +t+1))/(t+1))dt =(4/3)∫_0 ^1 ((t^3 +t^2 +t)/(t+1))dt =_(t+1=y) (4/3)∫_1 ^2 (((y−1)^3 +(y−1)^2 +y−1)/y)dy =(4/3)∫_1 ^2 ((y^3 −3y^2 +3y−1+y^2 −2y+1+y−1)/y)dy =(4/3)∫_1 ^2 ((y^3 −2y^2 +2y−1)/y)dy =(4/3)∫_1 ^2 (y^2 −2y+2−(1/y))dy =(4/3)[(y^3 /3)−y^2 +2y−log∣y∣]_1 ^2 =(4/3){(8/3)−4+4−log2−(1/3)+1−2} =(4/3){(7/3)−1−log2} =(4/3)((4/3)−log2) =((16)/9)−(4/3)log2](Q139827.png)
Commented by mnjuly1970 last updated on 01/May/21

Commented by mathmax by abdo last updated on 02/May/21

Answered by Ar Brandon last updated on 16/May/21

Commented by mnjuly1970 last updated on 01/May/21

Commented by SLVR last updated on 01/May/21

Commented by Ar Brandon last updated on 01/May/21
Commented by Ar Brandon last updated on 01/May/21
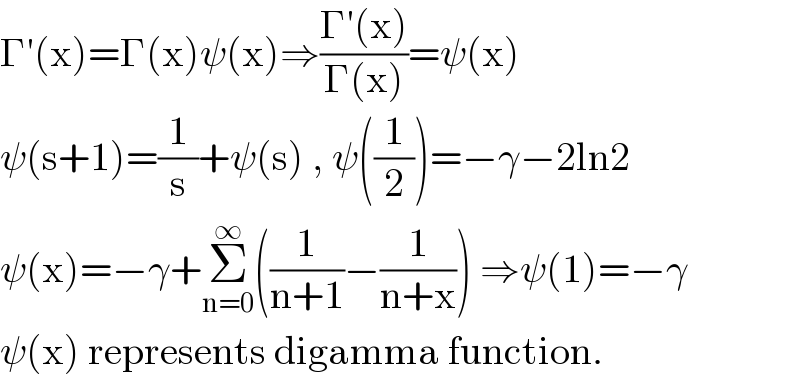
Commented by SLVR last updated on 01/May/21
Commented by Ar Brandon last updated on 01/May/21

