A class has 13 children. To play a game one child is the referee and the other children are divided in three teams with four children in each team. In how many ways can the class play the game?


Question and Answers Forum

All Questions Topic List
Permutation and Combination Questions
Previous in All Question Next in All Question
Previous in Permutation and Combination Next in Permutation and Combination
Question Number 142765 by mr W last updated on 05/Jun/21

$A c l a s s h a s 13 c h i l d r e n . T o p l a y a$ $g a m e o n e c h i l d i s t h e r e f e r e e a n d t h e$ $o t h e r c h i l d r e n a r e d i v i d e d i n t h r e e$ $t e a m s w i t h f o u r c h i l d r e n i n e a c h t e a m .$ $I n h o w m a n y w a y s c a n t h e c l a s s p l a y$ $t h e g a m e ?$
Commented by mr W last updated on 06/Jun/21

$g e n e r a l l y i f n d i s t i n c t o b j e c t s a r e$ $d i v i d e d i n s e v e r a l i d e n t i c a l b o x e s$ $w i t h n_{1}, n_{2}, . . ., n_{m} o b j e c t s r e s p e c t i v e l y .$ $n_{1} + n_{2} + . . . + n_{m} = n . t h e n u m b e r o f w a y s$ $i s \frac{n!}{n_{1}! n_{2}! . . . n_{m}!} .$ $i n c u r r e n t c a s e i t i s \frac{13!}{{(4!)}^{3} 1!} = 450450 .$ $o n e c a n a l s o g e t :$ $f o r t h e f i r s t g r o u p t h e r e a r e C_{4}^{13}$ $w a y s . f o r t h e s e c o n d g r o u p C_{4}^{9} w a y s$ $a n d t h e t h i r d g r o u p C_{4}^{5} w a y s . t h e$ $r e m a i n i n g o n e i s a u t o m a t i c a l l t h e$ $r e f e r e e .$ $\Rightarrow C_{4}^{13} \times C_{4}^{9} \times C_{4}^{5} = 450450 .$ $o r t o s e l e c t t h e r e f e r e e t h e r e a r e$ $C_{1}^{13} w a y s . f o r t h e f i r s t g r o u p C_{4}^{12} w a y s$ $a n d t h e s e c o n d g r o u p C_{4}^{8} w a y s, t h e$ $r e m a i n i n g c h i l d r e n a r e a u t o m a t i c a l l$ $t h e t h i r d g r o u p .$ $\Rightarrow C_{1}^{13} \times C_{4}^{12} \times C_{4}^{8} = 450450$
	Answered by gsk2684 last updated on 05/Jun/21

	$selecting a referee in 13 ways .$ $remaining 12 can be grouped into three each contain$ $4 children . It can be done in \frac{12!}{{(4!)}^{3} 3!}$ $simultaneously (13) (\frac{12!}{{(4!)}^{3} . 3!})$
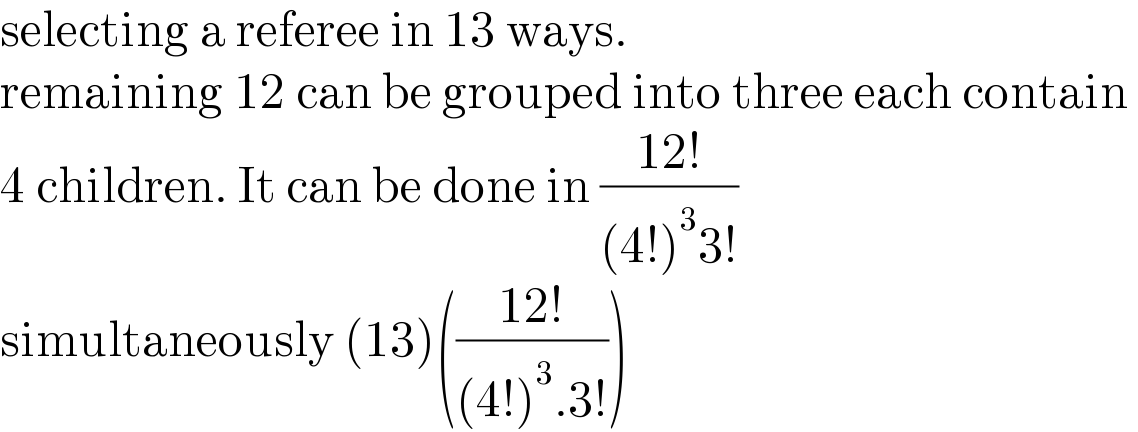
	Commented by mr W last updated on 05/Jun/21

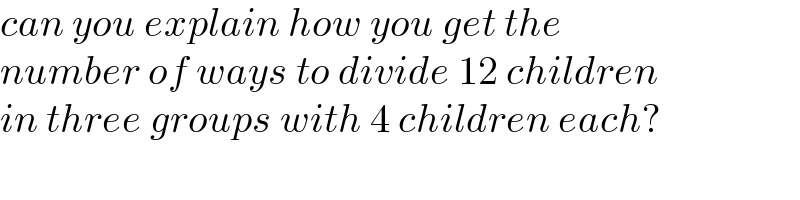
	$c a n y o u e x p l a i n h o w y o u g e t t h e$ $n u m b e r o f w a y s t o d i v i d e 12 c h i l d r e n$ $i n t h r e e g r o u p s w i t h 4 c h i l d r e n e a c h ?$
	Commented by gsk2684 last updated on 06/Jun/21

	$selecing 4 from 12 in 12_{C_{4}} ways for first group$ $selecing 4 from remain 8 in 8_{C_{4}} ways for second group$ $selecing 4 from remain 4 in 4_{C_{4}} ways for third group$ $but groups have equal members$ $so they can be interchange entirely$ $3 gruops can be interchanged$ $among themselves in 3! ways .$ $so required number of ways$ $is \frac{12_{C_{4}} . 8_{C_{4}} . 4_{C_{4}}}{3!} = \frac{12!}{{(4!)}^{3} . 3!}$
	Commented by mr W last updated on 06/Jun/21

	$t h a n k s f o r e x p l a n a t i o n! b u t t h e$ $a n s w e r i s n o t c o r r e c t i^{'} m a f r a i d .$ $s i n c e t h e o r d e r o f t h e g r o u p s {d o e s n}^{'} t$ $m a t t e r, t h e n u m b e r o f w a y s i s j u s t$ $\frac{12!}{{(4!)}^{3}} .$ $e v e n i f t h e o r d e r o f t h e g r o u p s$ $m a t t e r s, t h e n u m b e r o f w a y s i s$ $\frac{12!}{{(4!)}^{3}} \times 3!, n o t \frac{12!}{{(4!)}^{3} \times 3!} .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum