Question and Answers Forum

All Questions      Topic List

Integration Questions

Previous in All Question      Next in All Question      

Previous in Integration      Next in Integration      

Question Number 142875 by Snail last updated on 06/Jun/21

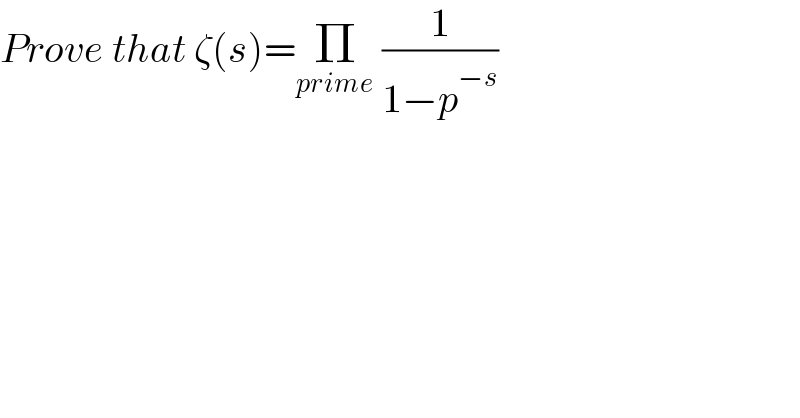
Prove that ζ(s)=Π_(prime) (1/(1−p^(−s) ))

$${Prove}\:{that}\:\zeta\left({s}\right)=\underset{{prime}} {\prod}\:\frac{\mathrm{1}}{\mathrm{1}−{p}^{−{s}} } \\ $$

Answered by Dwaipayan Shikari last updated on 06/Jun/21

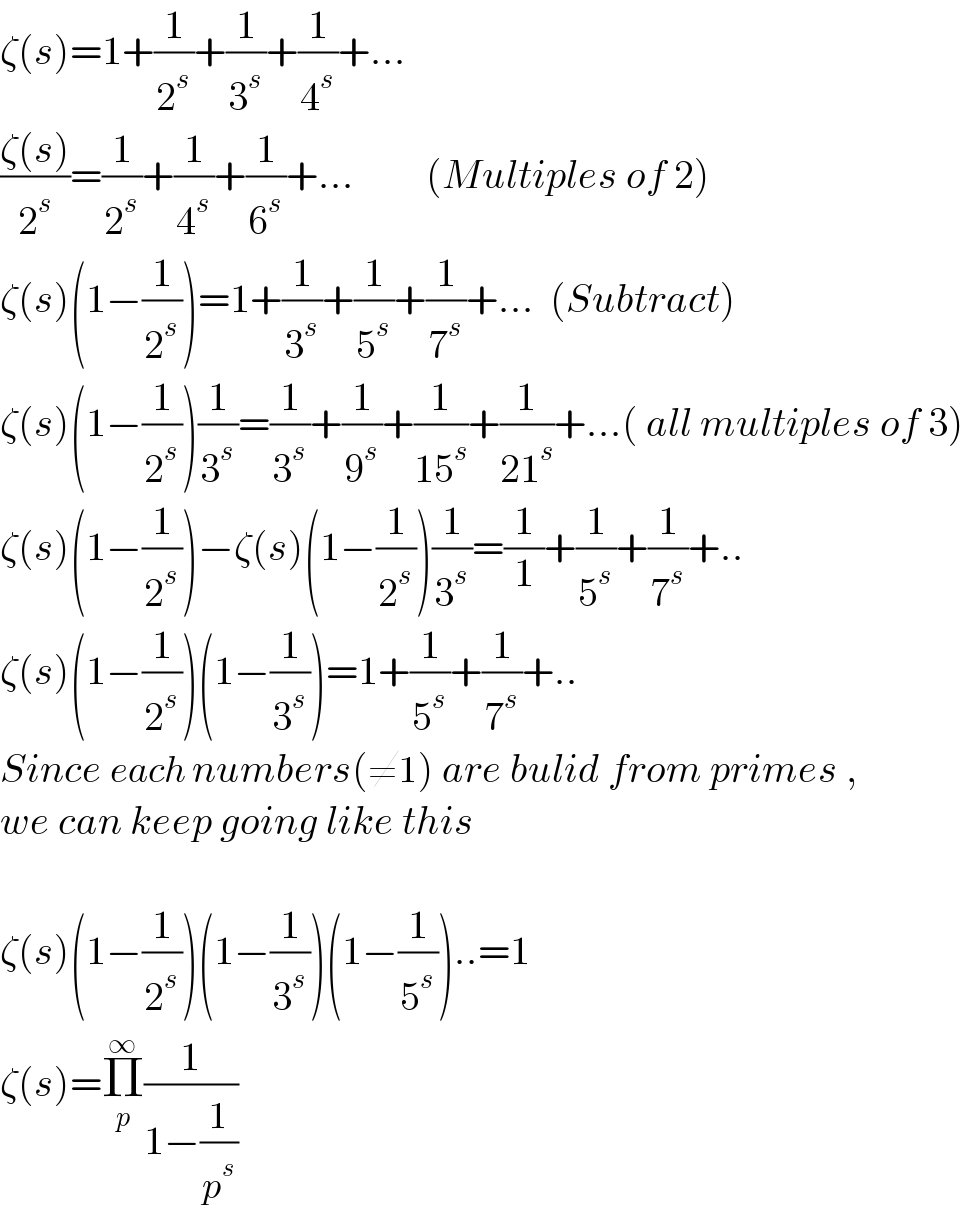
ζ(s)=1+(1/2^s )+(1/3^s )+(1/4^s )+... ((ζ(s))/2^s )=(1/2^s )+(1/4^s )+(1/6^s )+... (Multiples of 2) ζ(s)(1−(1/2^s ))=1+(1/3^s )+(1/5^s )+(1/7^s )+... (Subtract) ζ(s)(1−(1/2^s ))(1/3^s )=(1/3^s )+(1/9^s )+(1/(15^s ))+(1/(21^s ))+...( all multiples of 3) ζ(s)(1−(1/2^s ))−ζ(s)(1−(1/2^s ))(1/3^s )=(1/1)+(1/5^s )+(1/7^s )+.. ζ(s)(1−(1/2^s ))(1−(1/3^s ))=1+(1/5^s )+(1/7^s )+.. Since each numbers(≠1) are bulid from primes , we can keep going like this ζ(s)(1−(1/2^s ))(1−(1/3^s ))(1−(1/5^s ))..=1 ζ(s)=Π_p ^∞ (1/(1−(1/p^s )))

$$\zeta\left({s}\right)=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{2}^{{s}} }+\frac{\mathrm{1}}{\mathrm{3}^{{s}} }+\frac{\mathrm{1}}{\mathrm{4}^{{s}} }+... \\ $$$$\frac{\zeta\left({s}\right)}{\mathrm{2}^{{s}} }=\frac{\mathrm{1}}{\mathrm{2}^{{s}} }+\frac{\mathrm{1}}{\mathrm{4}^{{s}} }+\frac{\mathrm{1}}{\mathrm{6}^{{s}} }+...\:\:\:\:\:\:\:\:\:\left({Multiples}\:{of}\:\mathrm{2}\right) \\ $$$$\zeta\left({s}\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{s}} }\right)=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{3}^{{s}} }+\frac{\mathrm{1}}{\mathrm{5}^{{s}} }+\frac{\mathrm{1}}{\mathrm{7}^{{s}} }+...\:\:\left({Subtract}\right) \\ $$$$\zeta\left({s}\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{s}} }\right)\frac{\mathrm{1}}{\mathrm{3}^{{s}} }=\frac{\mathrm{1}}{\mathrm{3}^{{s}} }+\frac{\mathrm{1}}{\mathrm{9}^{{s}} }+\frac{\mathrm{1}}{\mathrm{15}^{{s}} }+\frac{\mathrm{1}}{\mathrm{21}^{{s}} }+...\left(\:{all}\:{multiples}\:{of}\:\mathrm{3}\right) \\ $$$$\zeta\left({s}\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{s}} }\right)−\zeta\left({s}\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{s}} }\right)\frac{\mathrm{1}}{\mathrm{3}^{{s}} }=\frac{\mathrm{1}}{\mathrm{1}}+\frac{\mathrm{1}}{\mathrm{5}^{{s}} }+\frac{\mathrm{1}}{\mathrm{7}^{{s}} }+.. \\ $$$$\zeta\left({s}\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{s}} }\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}^{{s}} }\right)=\mathrm{1}+\frac{\mathrm{1}}{\mathrm{5}^{{s}} }+\frac{\mathrm{1}}{\mathrm{7}^{{s}} }+..\:\: \\ $$$${Since}\:{each}\:{numbers}\left(\neq\mathrm{1}\right)\:{are}\:{bulid}\:{from}\:{primes}\:, \\ $$$${we}\:{can}\:{keep}\:{going}\:{like}\:{this} \\ $$$$ \\ $$$$\zeta\left({s}\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{2}^{{s}} }\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{3}^{{s}} }\right)\left(\mathrm{1}−\frac{\mathrm{1}}{\mathrm{5}^{{s}} }\right)..=\mathrm{1} \\ $$$$\zeta\left({s}\right)=\underset{{p}} {\overset{\infty} {\prod}}\frac{\mathrm{1}}{\mathrm{1}−\frac{\mathrm{1}}{{p}^{{s}} }} \\ $$

Commented by Snail last updated on 06/Jun/21

Thanks

$${Thanks} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com