Question Number 142910 by BHOOPENDRA last updated on 07/Jun/21
Answered by qaz last updated on 07/Jun/21
![y(t)=t+e^(−2t) +∫_0 ^t y(τ)e^(2(t−τ)) dτ L=(1/s^2 )+(1/(s+2))+(L/(s−2))..............L=L(y(t))(s) ⇒L=(((s^2 +s+2)(s−2))/(s^2 (s+2)(s−3))) =(2/3)∙(1/s^2 )+(B/s)+(4/5)∙(1/(s+2))+((14)/(45))∙(1/(s−3)) B=lim_(s→0) (d/ds)[s^2 L]=lim_(s→0) (d/ds)[(((s^2 +s+2)(s−2))/((s+2)(s−3)))] =lim_(s→0) (([(2s+1)(s−2)+(s^2 +s+2)](s+2)(s−3)−(s^2 +s+2)(s−2)(2s−1))/([(s+2)(s−3)]^2 )) =−(1/9) ⇒ L=(2/3)∙(1/s^2 )−(1/9)∙(1/s)+(4/5)∙(1/(s+2))+((14)/(45))∙(1/(s−3)) ⇒ y(t)=L^(−1) =(2/3)t−(1/9)+(4/5)e^(−2t) +((14)/(45))e^(3t)](Q142925.png)
$$\mathrm{y}\left(\mathrm{t}\right)=\mathrm{t}+\mathrm{e}^{−\mathrm{2t}} +\int_{\mathrm{0}} ^{\mathrm{t}} \mathrm{y}\left(\tau\right)\mathrm{e}^{\mathrm{2}\left(\mathrm{t}−\tau\right)} \mathrm{d}\tau \\ $$$$\mathscr{L}=\frac{\mathrm{1}}{\mathrm{s}^{\mathrm{2}} }+\frac{\mathrm{1}}{\mathrm{s}+\mathrm{2}}+\frac{\mathscr{L}}{\mathrm{s}−\mathrm{2}}..............\mathscr{L}=\mathscr{L}\left(\mathrm{y}\left(\mathrm{t}\right)\right)\left(\mathrm{s}\right) \\ $$$$\Rightarrow\mathscr{L}=\frac{\left(\mathrm{s}^{\mathrm{2}} +\mathrm{s}+\mathrm{2}\right)\left(\mathrm{s}−\mathrm{2}\right)}{\mathrm{s}^{\mathrm{2}} \left(\mathrm{s}+\mathrm{2}\right)\left(\mathrm{s}−\mathrm{3}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{2}}{\mathrm{3}}\centerdot\frac{\mathrm{1}}{\mathrm{s}^{\mathrm{2}} }+\frac{\mathrm{B}}{\mathrm{s}}+\frac{\mathrm{4}}{\mathrm{5}}\centerdot\frac{\mathrm{1}}{\mathrm{s}+\mathrm{2}}+\frac{\mathrm{14}}{\mathrm{45}}\centerdot\frac{\mathrm{1}}{\mathrm{s}−\mathrm{3}} \\ $$$$\mathrm{B}=\underset{\mathrm{s}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{d}}{\mathrm{ds}}\left[\mathrm{s}^{\mathrm{2}} \mathscr{L}\right]=\underset{\mathrm{s}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\mathrm{d}}{\mathrm{ds}}\left[\frac{\left(\mathrm{s}^{\mathrm{2}} +\mathrm{s}+\mathrm{2}\right)\left(\mathrm{s}−\mathrm{2}\right)}{\left(\mathrm{s}+\mathrm{2}\right)\left(\mathrm{s}−\mathrm{3}\right)}\right] \\ $$$$ \\ $$$$\:\:\:\:=\underset{\mathrm{s}\rightarrow\mathrm{0}} {\mathrm{lim}}\frac{\left[\left(\mathrm{2s}+\mathrm{1}\right)\left(\mathrm{s}−\mathrm{2}\right)+\left(\mathrm{s}^{\mathrm{2}} +\mathrm{s}+\mathrm{2}\right)\right]\left(\mathrm{s}+\mathrm{2}\right)\left(\mathrm{s}−\mathrm{3}\right)−\left(\mathrm{s}^{\mathrm{2}} +\mathrm{s}+\mathrm{2}\right)\left(\mathrm{s}−\mathrm{2}\right)\left(\mathrm{2s}−\mathrm{1}\right)}{\left[\left(\mathrm{s}+\mathrm{2}\right)\left(\mathrm{s}−\mathrm{3}\right)\right]^{\mathrm{2}} } \\ $$$$\:\:\:\:\:=−\frac{\mathrm{1}}{\mathrm{9}} \\ $$$$\Rightarrow\:\:\:\:\:\mathscr{L}=\frac{\mathrm{2}}{\mathrm{3}}\centerdot\frac{\mathrm{1}}{\mathrm{s}^{\mathrm{2}} }−\frac{\mathrm{1}}{\mathrm{9}}\centerdot\frac{\mathrm{1}}{\mathrm{s}}+\frac{\mathrm{4}}{\mathrm{5}}\centerdot\frac{\mathrm{1}}{\mathrm{s}+\mathrm{2}}+\frac{\mathrm{14}}{\mathrm{45}}\centerdot\frac{\mathrm{1}}{\mathrm{s}−\mathrm{3}} \\ $$$$\Rightarrow\:\:\:\:\:\:\mathrm{y}\left(\mathrm{t}\right)=\mathscr{L}^{−\mathrm{1}} =\frac{\mathrm{2}}{\mathrm{3}}\mathrm{t}−\frac{\mathrm{1}}{\mathrm{9}}+\frac{\mathrm{4}}{\mathrm{5}}\mathrm{e}^{−\mathrm{2t}} +\frac{\mathrm{14}}{\mathrm{45}}\mathrm{e}^{\mathrm{3t}} \\ $$
Commented by BHOOPENDRA last updated on 07/Jun/21
$${tq}\:{sir} \\ $$
Answered by Olaf_Thorendsen last updated on 07/Jun/21
$$ \\ $$$${y}\left({t}\right)\:=\:{t}+{e}^{−\mathrm{2}{t}} +{e}^{\mathrm{2}{t}} \int_{\mathrm{0}} ^{{t}} {y}\left(\tau\right){e}^{−\mathrm{2}\tau} {d}\tau \\ $$$${y}\left(\mathrm{0}\right)\:=\:\mathrm{0}+{e}^{\mathrm{0}} +{e}^{\mathrm{0}} ×\mathrm{0}\:=\:\mathrm{1} \\ $$$${e}^{−\mathrm{2}{t}} {y}\left({t}\right)\:=\:{e}^{−\mathrm{2}{t}} {t}+{e}^{−\mathrm{4}{t}} +\int_{\mathrm{0}} ^{{t}} {y}\left(\tau\right){e}^{−\mathrm{2}\tau} {d}\tau \\ $$$${e}^{−\mathrm{2}{t}} \left({y}'\left({t}\right)−\mathrm{2}{y}\left({t}\right)\right)\:=\:{e}^{−\mathrm{2}{t}} \left(\mathrm{1}−\mathrm{2}{t}\right) \\ $$$$−\mathrm{4}{e}^{−\mathrm{4}{t}} +{y}\left({t}\right){e}^{−\mathrm{2}{t}} \\ $$$${y}'\left({t}\right)−\mathrm{3}{y}\left({t}\right)\:=\:\mathrm{1}−\mathrm{2}{t}−\mathrm{4}{e}^{−\mathrm{2}{t}} \\ $$$${y}\left({t}\right)\:=\:\frac{\mathrm{2}}{\mathrm{3}}{t}−\frac{\mathrm{1}}{\mathrm{9}}+\frac{\mathrm{4}}{\mathrm{5}}{e}^{−\mathrm{2}{t}} +{ae}^{\mathrm{3}{t}} \\ $$$${y}\left(\mathrm{0}\right)\:=\:−\frac{\mathrm{1}}{\mathrm{9}}+\frac{\mathrm{4}}{\mathrm{5}}+{a}\:=\:\mathrm{1}\:\Rightarrow\:{a}\:=\:\frac{\mathrm{14}}{\mathrm{45}} \\ $$$${y}\left({t}\right)\:=\:\frac{\mathrm{2}}{\mathrm{3}}{t}−\frac{\mathrm{1}}{\mathrm{9}}+\frac{\mathrm{4}}{\mathrm{5}}{e}^{−\mathrm{2}{t}} +\frac{\mathrm{14}}{\mathrm{45}}{e}^{\mathrm{3}{t}} \\ $$
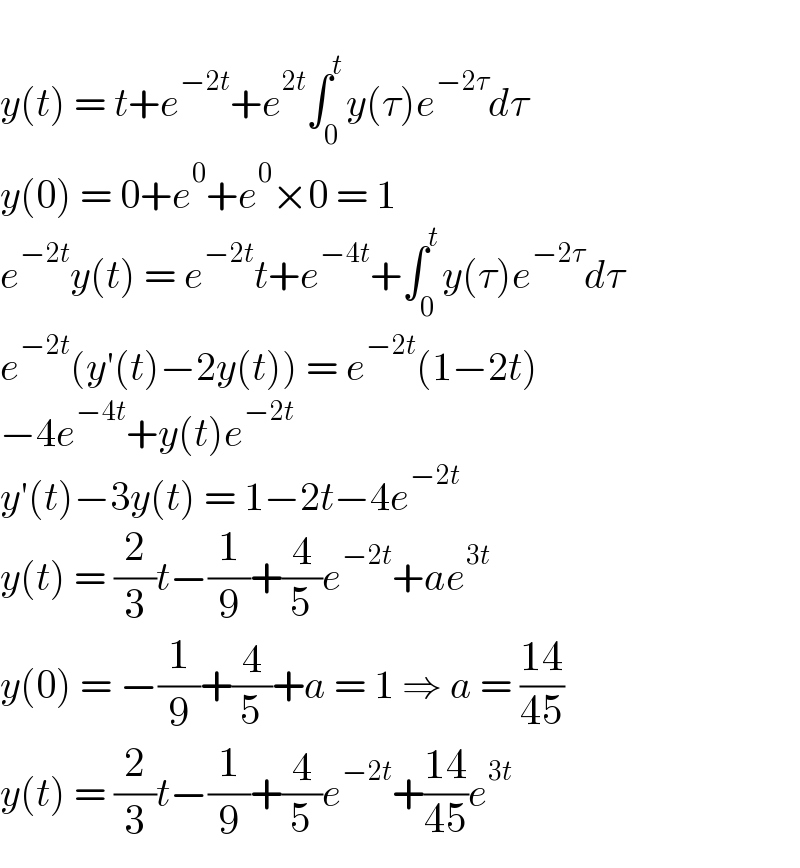
Commented by BHOOPENDRA last updated on 07/Jun/21
$${tq}\:{sir} \\ $$