Question and Answers Forum

All Questions      Topic List

Limits Questions

Previous in All Question      Next in All Question      

Previous in Limits      Next in Limits      

Question Number 143064 by EDWIN88 last updated on 09/Jun/21

$$\underset{x\to 0}{\lim }\frac{\sqrt[3]{1+{\mathrm{x}}^2}-\sqrt[4]{1-2\mathrm{x}}}{\mathrm{x}+{\mathrm{x}}^2}=?$$$$\underset{x\to 1}{\lim }\frac{\sqrt[3]{7+{\mathrm{x}}^2}-\sqrt{3+{\mathrm{x}}^2}}{\mathrm{x}-1}=?$$

Answered by bramlexs22 last updated on 09/Jun/21

Answered by Mathspace last updated on 09/Jun/21

$$f\left(x\right)=\frac{{(1+x^2)}^3-{(1-2x)}^{\frac{1}{4}}}{x^2+x}\Rightarrow$$$$f\left(x\right)\sim \frac{1+3x^2-(1-\frac{x}{2})}{x^2+x}\Rightarrow$$$$f\left(x\right)\sim \frac{3x^2+\frac{x}{2}}{x^2+x}=\frac{3x+\frac{1}{2}}{x+1}\Rightarrow$$$${lim}_{x\to 0}f\left(x\right)=\frac{1}{2}$$

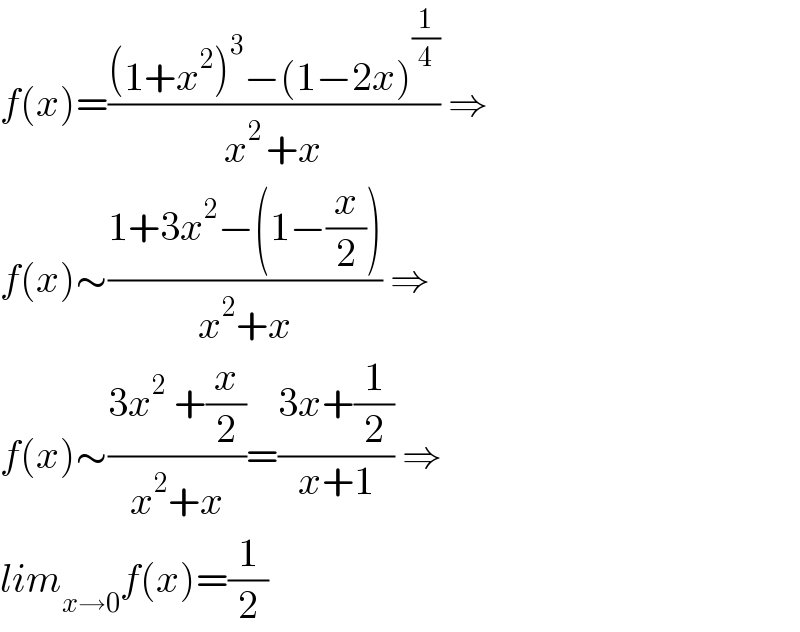
Answered by Mathspace last updated on 09/Jun/21

$$g\left(x\right)=\frac{{(7+x^2)}^{\frac{1}{3}}-{(3+x^2)}^{\frac{1}{2}}}{x-1}$$$$wedothechangementx-1=t(sot\to 0)$$$$g\left(x\right)=g(t+1)=\frac{{(7+{(t+1)}^2)}^{\frac{1}{3}}-{(3+{(t+1)}^2)}^{\frac{1}{2}}}{t}$$$$=\frac{{(7+t^2+2t+1)}^{\frac{1}{3}}-{(3+t^2+2t+1)}^{\frac{1}{2}}}{t}$$$$=\frac{{(8+t^2+2t)}^{\frac{1}{3}}-{(4+t^2+2t)}^{\frac{1}{2}}}{t}$$$$g(t+1)=\frac{2{(1+\frac{t^2+2t}{8})}^{\frac{1}{3}}-2{(1+\frac{t^2+2t}{4})}^{\frac{1}{2}}}{t}$$$$\sim \frac{2(1+\frac{1}{24}(t^2+2t))-2(1+\frac{1}{8}(t^2+2t))}{t}$$$$=\frac{\frac{1}{12}(t^2+2t)-\frac{1}{4}(t^2+2t)}{t}$$$$=-\frac{1}{6}\times \frac{t^2+2t}{t}=-\frac{1}{6}(t+2)\to -\frac{1}{3}$$$$\Rightarrow {lim}_{t\to 1}g\left(x\right)=-\frac{1}{3}$$

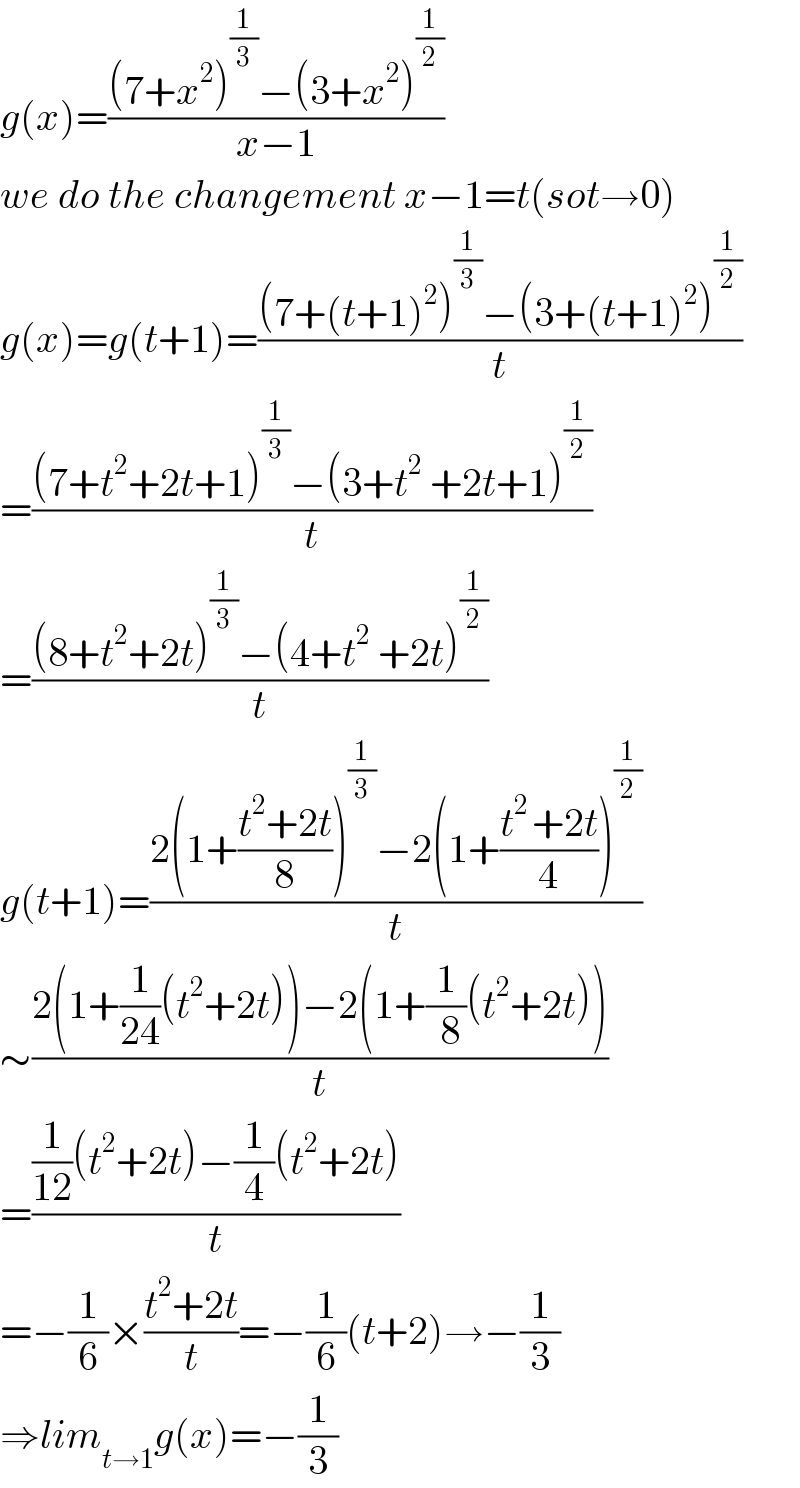
Answered by Olaf_Thorendsen last updated on 09/Jun/21

$$f\left(x\right)=\frac{\sqrt[3]{1+x^2}-\sqrt[4]{1-2x}}{x+x^2}$$$$f\left(x\right)\underset{0}{\sim }\frac{(1+\frac{1}{3}{x}^2)-(1+\frac{1}{4}(-2x))}{x+x^2}$$$$f\left(x\right)\underset{0}{\sim }\frac{\frac{1}{3}{x}^2+\frac{1}{2}x}{x+x^2}\to \frac{1}{2}$$$$g\left(x\right)=\frac{\sqrt[3]{7+x^2}-\sqrt{3+x^2}}{x-1}$$$$g(x+1)=\frac{\sqrt[3]{8+2x+x^2}-\sqrt{4+2x+x^2}}{x}$$$$g(x+1)=\frac{2\sqrt[3]{1+\frac{1}{4}x+\frac{1}{8}{x}^2}-2\sqrt{1+\frac{1}{2}x+\frac{1}{4}{x}^2}}{x}$$$$g(x+1)\underset{0}{\sim }\frac{2(1+\frac{1}{3}(\frac{1}{4}x+\frac{1}{8}{x}^2))-2(1+\frac{1}{2}(\frac{1}{2}x+\frac{1}{4}{x}^2))}{x}$$$$g(x+1)\underset{0}{\sim }\frac{\frac{2}{3}(\frac{1}{4}x+\frac{1}{8}{x}^2)-(\frac{1}{2}x+\frac{1}{4}{x}^2)}{x}\to \frac{1}{6}-\frac{1}{2}=-\frac{1}{3}$$$$$$$$$$$$$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com