
Question and Answers Forum
Question Number 143167 by pticantor last updated on 10/Jun/21
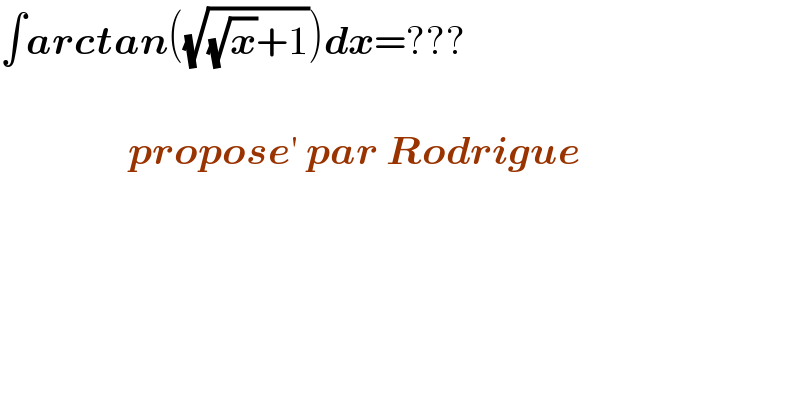
Answered by Olaf_Thorendsen last updated on 10/Jun/21
![F(x) = ∫arctan((√((√x)+1)))dx F(x) = xarctan((√((√x)+1)))−∫x(((1/(2(√x)))/(2(√((√x)+1))))/(1+((√x)+1))) dx F(x) = xarctan((√((√x)+1)))−(1/4)∫((√x)/( ((√x)+2)(√((√x)+1)))) dx G(x) = ∫((√x)/( ((√x)+2)(√((√x)+1)))) dx Let u = (√x) G(u) = ∫(u/( (u+2)(√(u+1)))) 2udu G(u) = ∫((2u^2 )/( (u+2)(√(u+1)))) du Let v = (√(u+1)) G(v) = ∫((2(v^2 −1)^2 )/( (v^2 −1+2)v)) 2vdv G(v) = ∫((4(v^2 −1)^2 )/( v^2 +1)) dv G(v) = ∫[4v^2 −12+((16)/( v^2 +1))] dv G(v) = (4/3)v^3 −12v+16arctanv G(u) = (4/3)(u+1)^(3/2) −12(√(u+1))+16arctan((√(u+1))) G(x) = (4/3)((√x)+1)^(3/2) −12(√((√x)+1))+16arctan((√((√x)+1))) F(x) = xarctan((√((√x)+1)))−(1/4)G(x) F(x) = xarctan((√((√x)+1)))− (1/3)((√x)+1)^(3/2) +3(√((√x)+1))−4arctan((√((√x)+1))) F(x) = (x−4)arctan((√((√x)+1)))− (1/3)((√x)+1)^(3/2) +3(√((√x)+1))](Q143173.png)
Answered by mathmax by abdo last updated on 11/Jun/21

| ||
Question and Answers Forum | ||
Question Number 143167 by pticantor last updated on 10/Jun/21 | ||
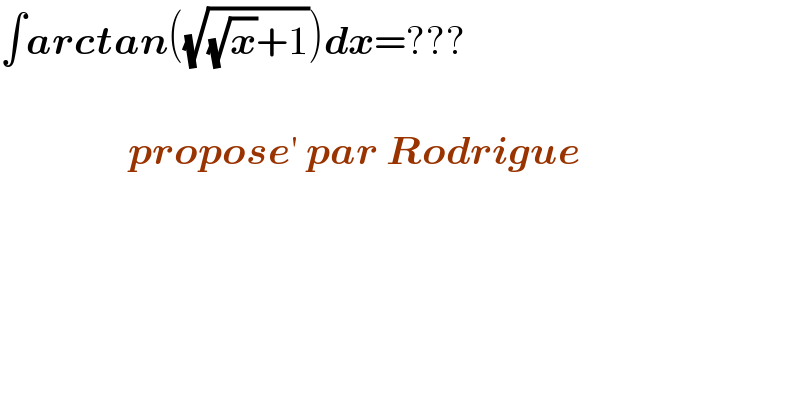 | ||
Answered by Olaf_Thorendsen last updated on 10/Jun/21 | ||
![F(x) = ∫arctan((√((√x)+1)))dx F(x) = xarctan((√((√x)+1)))−∫x(((1/(2(√x)))/(2(√((√x)+1))))/(1+((√x)+1))) dx F(x) = xarctan((√((√x)+1)))−(1/4)∫((√x)/( ((√x)+2)(√((√x)+1)))) dx G(x) = ∫((√x)/( ((√x)+2)(√((√x)+1)))) dx Let u = (√x) G(u) = ∫(u/( (u+2)(√(u+1)))) 2udu G(u) = ∫((2u^2 )/( (u+2)(√(u+1)))) du Let v = (√(u+1)) G(v) = ∫((2(v^2 −1)^2 )/( (v^2 −1+2)v)) 2vdv G(v) = ∫((4(v^2 −1)^2 )/( v^2 +1)) dv G(v) = ∫[4v^2 −12+((16)/( v^2 +1))] dv G(v) = (4/3)v^3 −12v+16arctanv G(u) = (4/3)(u+1)^(3/2) −12(√(u+1))+16arctan((√(u+1))) G(x) = (4/3)((√x)+1)^(3/2) −12(√((√x)+1))+16arctan((√((√x)+1))) F(x) = xarctan((√((√x)+1)))−(1/4)G(x) F(x) = xarctan((√((√x)+1)))− (1/3)((√x)+1)^(3/2) +3(√((√x)+1))−4arctan((√((√x)+1))) F(x) = (x−4)arctan((√((√x)+1)))− (1/3)((√x)+1)^(3/2) +3(√((√x)+1))](Q143173.png) | ||
| ||
Answered by mathmax by abdo last updated on 11/Jun/21 | ||
 | ||
| ||