
Question and Answers Forum
Question Number 143439 by ERA last updated on 14/Jun/21
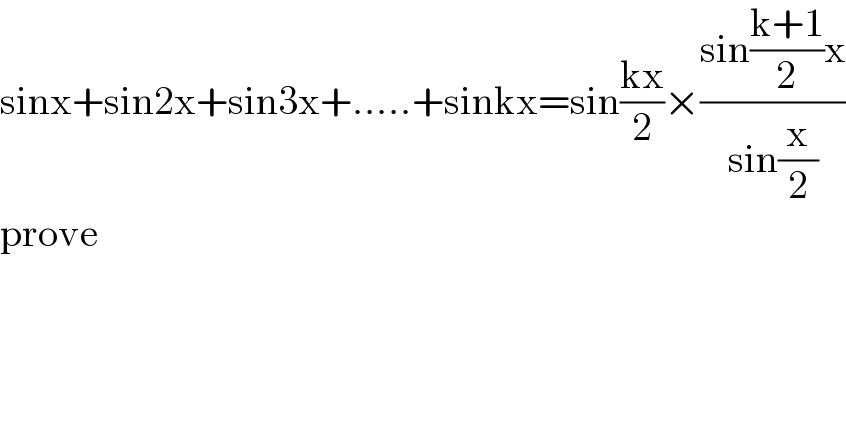
Answered by Mathspace last updated on 14/Jun/21
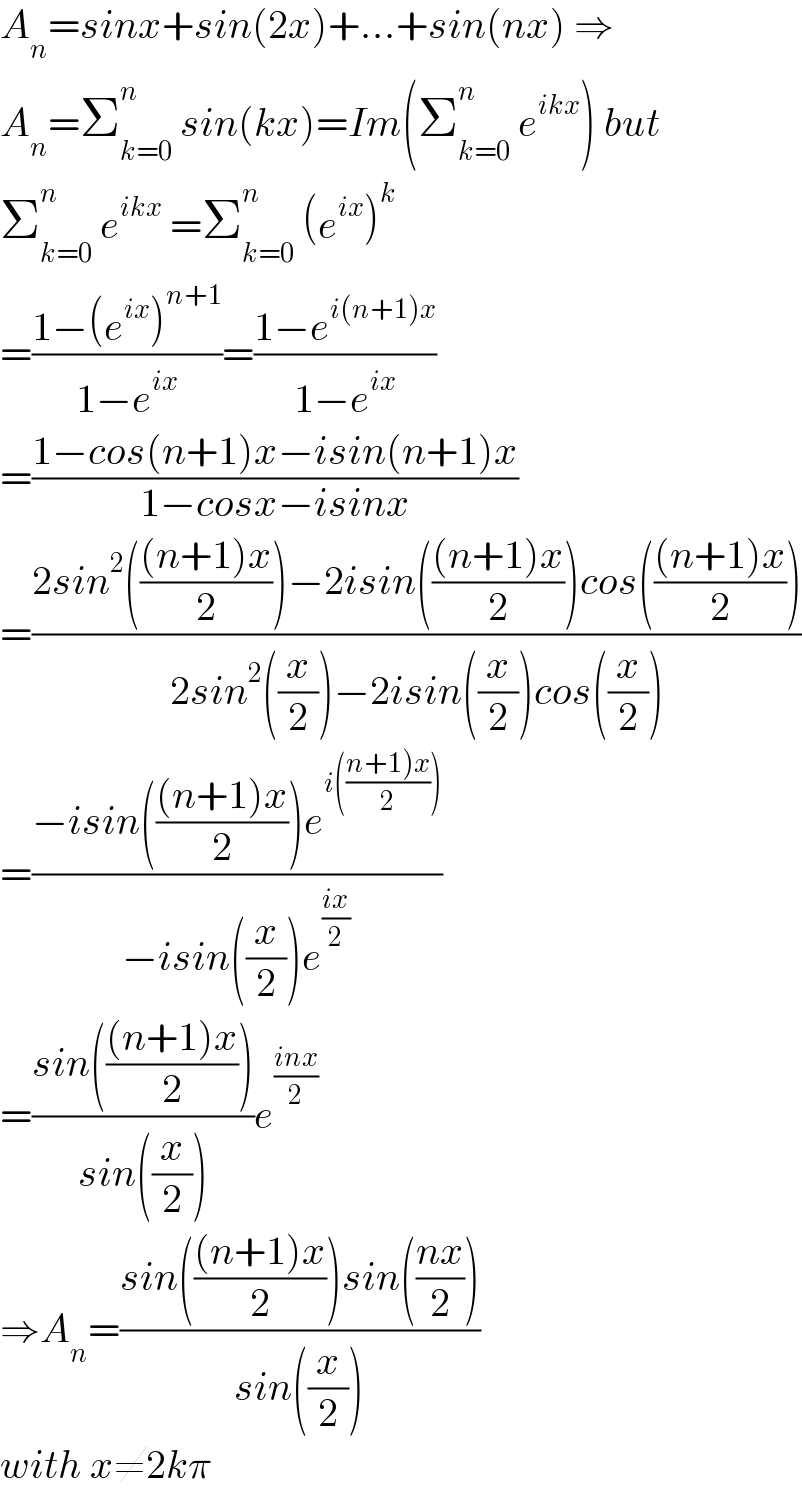
Answered by gsk2684 last updated on 14/Jun/21
![Σ_(t=1) ^k sin tx=imΣ_(t=1) ^k e^(itx) =imΣ_(t=1) ^k (e^(ix) )^t =im((e^(ix) ((e^(ix) )^k −1))/(e^(ix) −1)) =im((e^(i(k+1)x) −e^(ix) )/(e^(ix) −1)) =im{(e^(i(k+1)x) −e^(ix) )(e^(−ix) −1)/((cos x −1)^2 +sin^2 x)} =im((e^(ikx) −e^(i(k+1)x) −1+e^(ix) )/(2(1−cos x))) =(sin kx + sin x −sin (k+1)x)/(4 sin^2 (x/2)) =((2sin (((k+1)x)/2)cos(((k−1)x)/2)−2sin (((k+1)x)/2)cos (((k+1)x)/2))/(4sin^2 (x/2))) ={2sin (((k+1)x)/2).[cos(((kx)/2)−(x/2))−cos(((kx)/2)+(x/2))]}/4sin^2 (x/2) =((2sin (((k+1)x)/2).2sin ((kx)/2)sin (x/2))/(4sin^2 (x/2)))](Q143445.png)
| ||
Question and Answers Forum | ||
Question Number 143439 by ERA last updated on 14/Jun/21 | ||
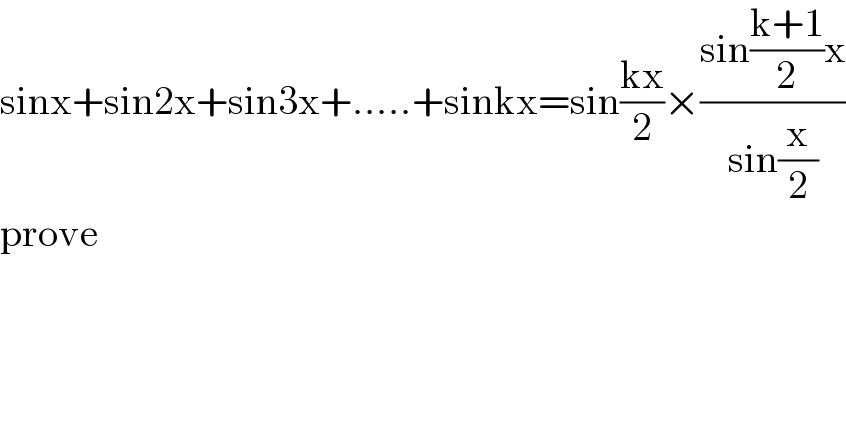 | ||
Answered by Mathspace last updated on 14/Jun/21 | ||
 | ||
| ||
Answered by gsk2684 last updated on 14/Jun/21 | ||
![Σ_(t=1) ^k sin tx=imΣ_(t=1) ^k e^(itx) =imΣ_(t=1) ^k (e^(ix) )^t =im((e^(ix) ((e^(ix) )^k −1))/(e^(ix) −1)) =im((e^(i(k+1)x) −e^(ix) )/(e^(ix) −1)) =im{(e^(i(k+1)x) −e^(ix) )(e^(−ix) −1)/((cos x −1)^2 +sin^2 x)} =im((e^(ikx) −e^(i(k+1)x) −1+e^(ix) )/(2(1−cos x))) =(sin kx + sin x −sin (k+1)x)/(4 sin^2 (x/2)) =((2sin (((k+1)x)/2)cos(((k−1)x)/2)−2sin (((k+1)x)/2)cos (((k+1)x)/2))/(4sin^2 (x/2))) ={2sin (((k+1)x)/2).[cos(((kx)/2)−(x/2))−cos(((kx)/2)+(x/2))]}/4sin^2 (x/2) =((2sin (((k+1)x)/2).2sin ((kx)/2)sin (x/2))/(4sin^2 (x/2)))](Q143445.png) | ||
| ||