
Question and Answers Forum
Question Number 144413 by imjagoll last updated on 25/Jun/21
![lim_(x→1) [ (1/(4−4(√x)))−(1/(5−5(x)^(1/5) )) ] =?](Q144413.png)
Answered by Olaf_Thorendsen last updated on 25/Jun/21
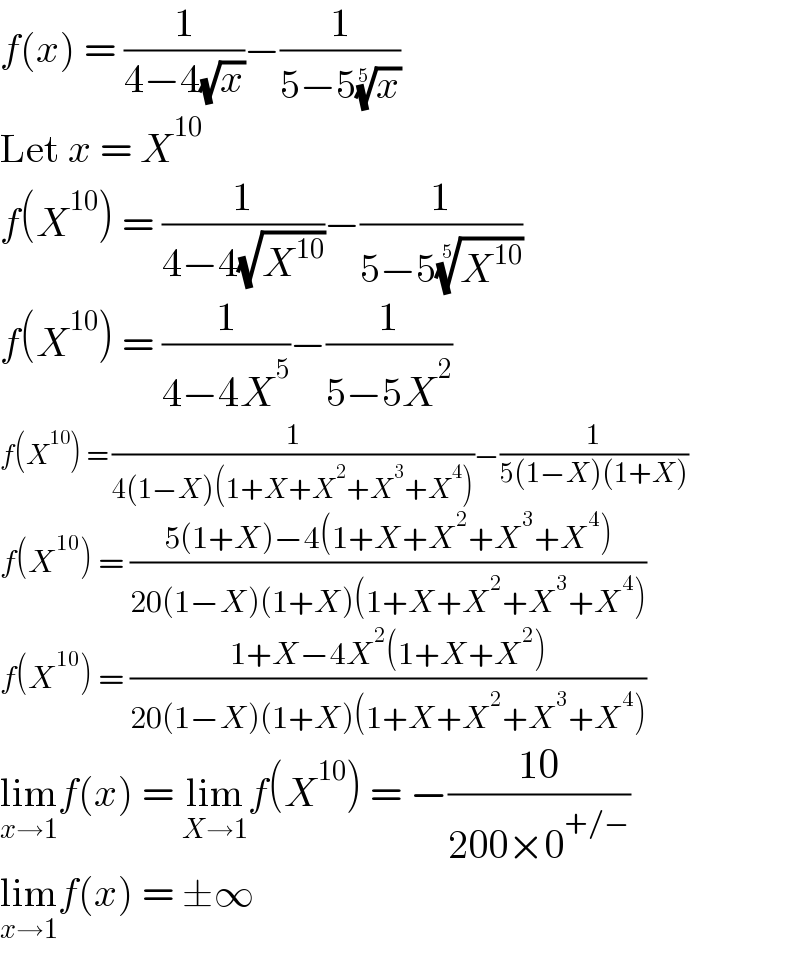
Answered by mathmax by abdo last updated on 25/Jun/21
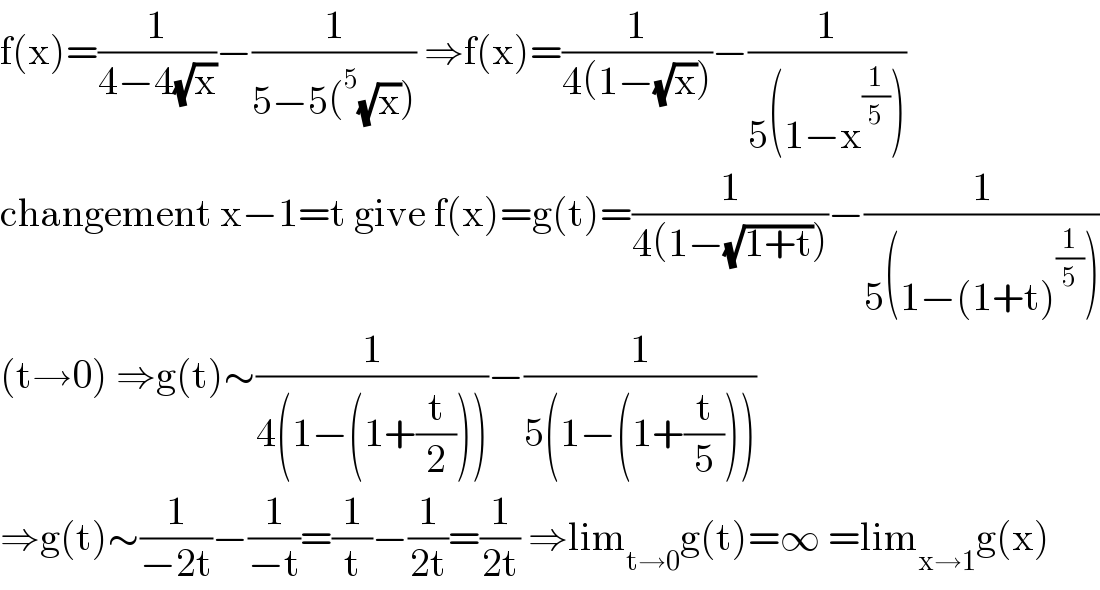
| ||
Question and Answers Forum | ||
Question Number 144413 by imjagoll last updated on 25/Jun/21 | ||
![lim_(x→1) [ (1/(4−4(√x)))−(1/(5−5(x)^(1/5) )) ] =?](Q144413.png) | ||
Answered by Olaf_Thorendsen last updated on 25/Jun/21 | ||
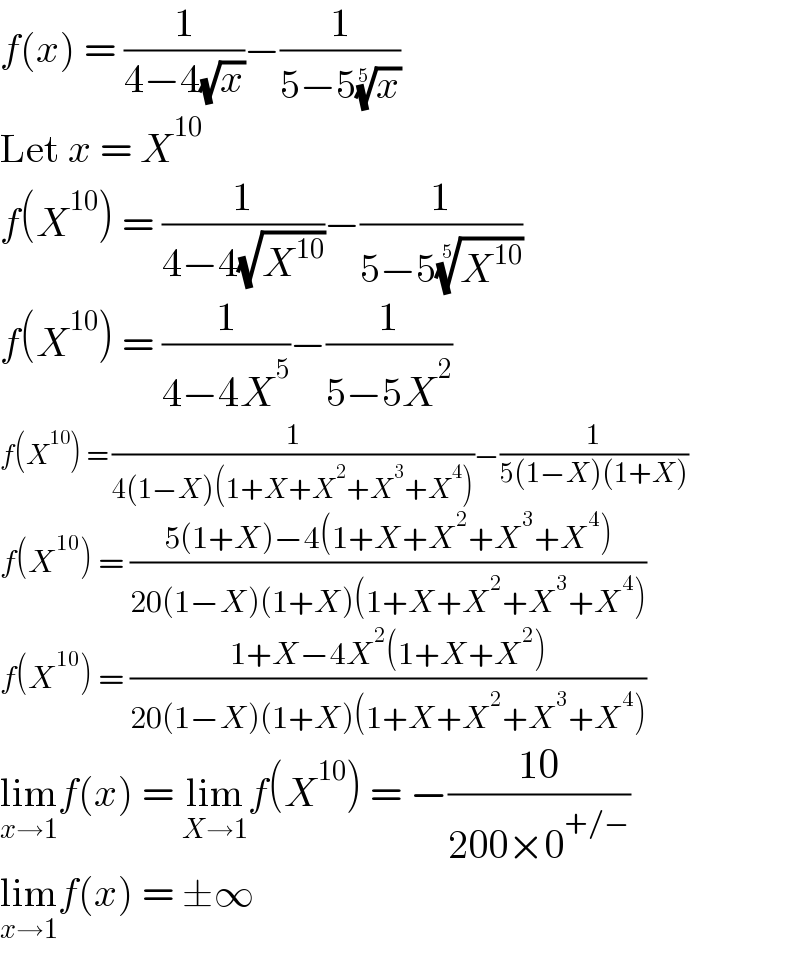 | ||
| ||
Answered by mathmax by abdo last updated on 25/Jun/21 | ||
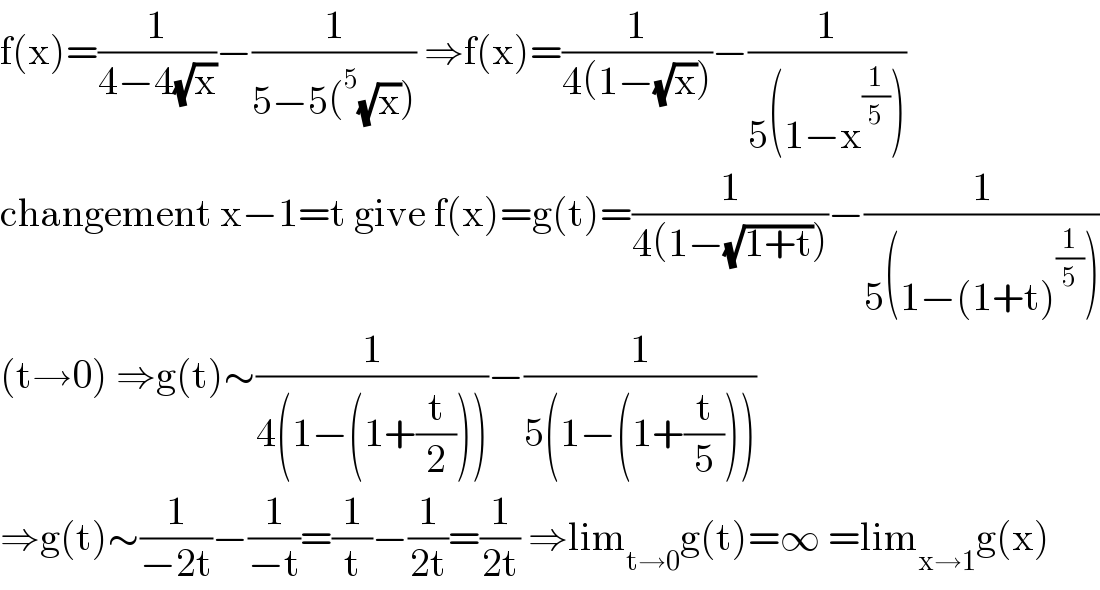 | ||
| ||