Math Question and Answers


Question and Answers Forum

All Questions Topic List
Mechanics Questions
Previous in All Question Next in All Question
Previous in Mechanics Next in Mechanics
Question Number 144420 by ajfour last updated on 25/Jun/21

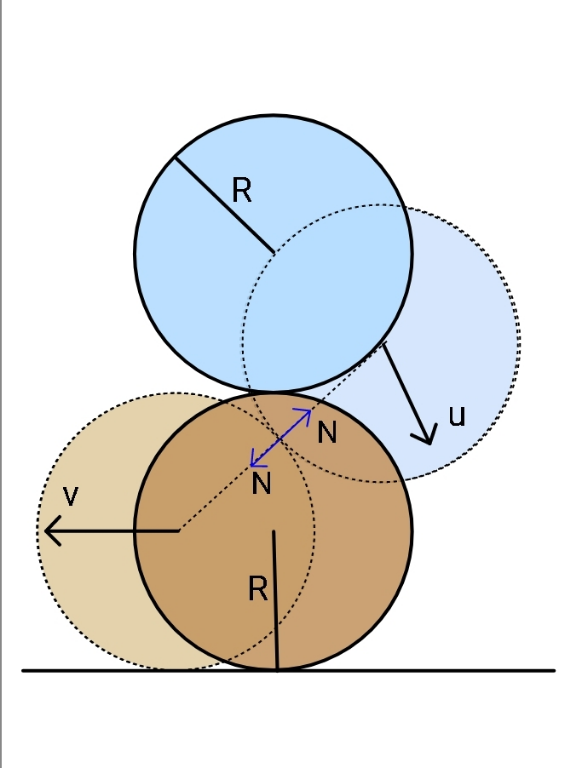
Commented by ajfour last updated on 25/Jun/21

$T w o c y l i n d e r s o n e o n t o p o f$ $a n o t h e r a r e r e l e a s e d a n d$ $m o t i o n i n i t i a t e d w i t h a m i l d$ $d i s t u r b a n c e . I f a l l s u r f a c e s$ $a r e f r i c t i o n l e s s f i n d t h e$ $s p e e d s u a n d v o f t h e c y l i n d e r s$ $j u s t w h e n t h e y l o o s e c o n t a c t$ $w i t h e a c h o t h e r .$
	Answered by ajfour last updated on 25/Jun/21

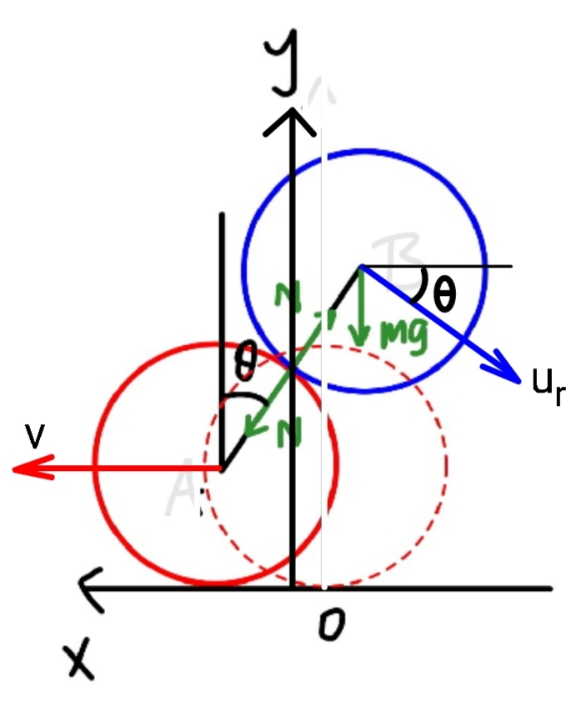
	Commented by ajfour last updated on 25/Jun/21
	$energy conserva^n : ....(i) mg(2R)(1−cos θ) =(1/2)m{v^2 +(u_r cos θ−v)^2 +u_r ^2 sin^2 θ} momentum conserva^n : mv=m(u_r cos θ−v) ⇒ u_r cos θ=2v ...(ii) m(centripetal acc.)=F_r ((mu_r ^2 )/(2R))=mgcos θ ....(iii) ⇒ 2gR=(u_r ^2 /(cos θ)) ....(A) now using (ii) (v^2 /u_r ^2 )=((cos^2 θ)/4) .....(B) using all (A),(B) in ..(i) ((2(1−cos θ))/(cos θ))=((cos^2 θ)/2)+1−cos^2 θ ⇒ 4−4cos θ=−cos^3 θ+2cos θ ⇒ cos^3 θ−6cos θ+4=0 ⇒ cos θ=(√3)−1 now v^2 =((u_r ^2 cos^2 θ)/4)=((gRcos^3 θ)/2) v^2 =((gR((√3)−1)^3 )/2)=gR(3(√3)−5) v=(√(gR(3(√3)−5)))$
	$e n e r g y {c o n s e r v a}^{n} : . . . . (i)$ $m g (2 R) (1 - \cos θ)$ $= \frac{1}{2} m {v^{2} + {(u_{r} \cos θ - v)}^{2} + u_{r}^{2} \sin^{2} θ}$ $m o m e n t u m {c o n s e r v a}^{n} :$ $m v = m (u_{r} \cos θ - v)$ $\Rightarrow u_{r} \cos θ = 2 v . . . (i i)$ $m (c e n t r i p e t a l a c c .) = F_{r}$ $\frac{{m u}_{r}^{2}}{2 R} = m g \cos θ . . . . (i i i)$ $\Rightarrow 2 g R = \frac{u_{r}^{2}}{\cos θ} . . . . (A)$ $n o w u s i n g (i i)$ $\frac{v^{2}}{u_{r}^{2}} = \frac{\cos^{2} θ}{4} . . . . . (B)$ $u s i n g a l l (A), (B) i n . . (i)$ $\frac{2 (1 - \cos θ)}{\cos θ} = \frac{\cos^{2} θ}{2} + 1 - \cos^{2} θ$ $\Rightarrow 4 - 4 \cos θ = - \cos^{3} θ + 2 \cos θ$ $\Rightarrow \cos^{3} θ - 6 \cos θ + 4 = 0$ $\Rightarrow \cos θ = \sqrt{3} - 1$ $n o w v^{2} = \frac{u_{r}^{2} \cos^{2} θ}{4} = \frac{g R \cos^{3} θ}{2}$ $v^{2} = \frac{g R {(\sqrt{3} - 1)}^{3}}{2} = g R (3 \sqrt{3} - 5)$ $v = \sqrt{g R (3 \sqrt{3} - 5)}$
	Answered by mr W last updated on 25/Jun/21

	Commented by mr W last updated on 25/Jun/21

	$x_{B} = x_{A} - 2 R \sin θ$ $y_{B} = R + 2 R \cos θ$ $ω = \frac{d θ}{d t}$ $v = \frac{{d x}_{A}}{d t}$ $a = \frac{d v}{d t}$ $u_{B x} = \frac{{d x}_{B}}{d t} = v - 2 R \cos θ ω = - v$ $\Rightarrow v = R \cos θ ω$ $u_{B y} = \frac{{d y}_{B}}{d t} = - 2 R \sin θ ω$ $\frac{1}{2} m (v^{2} + u_{B x}^{2} + u_{B y}^{2}) = m g 2 R (1 - \cos θ)$ $v^{2} + {(v - 2 R \cos θ ω)}^{2} + {(- 2 R \sin θ ω)}^{2} = 4 g R (1 - \cos θ)$ $R^{2} \cos^{2} θ ω^{2} + R^{2} \cos^{2} θ ω^{2} + 4 R^{2} \sin^{2} θ ω^{2} = 4 g R (1 - \cos θ)$ $(1 + \sin^{2} θ) ω^{2} = 2 g (1 - \cos θ)$ $\Rightarrow ω^{2} = \frac{2 g (1 - \cos θ)}{R (1 + \sin^{2} θ)}$ $\Rightarrow ω = \sqrt{\frac{2 g (1 - \cos θ)}{R (2 - \cos^{2} θ)}}$ $2 ω \frac{d ω}{d θ} = \frac{2 g}{R} [\frac{\sin θ}{1 + \sin^{2} θ} - \frac{2 \sin θ \cos θ (1 - \cos θ)}{{(1 + \sin^{2} θ)}^{2}}]$ $ω \frac{d ω}{d θ} = \frac{g \sin θ (\cos^{2} θ - 2 \cos θ + 2)}{R {(1 + \sin^{2} θ)}^{2}}$ $a = \frac{d v}{d t} = R ω (- \sin θ ω + \cos θ \frac{d ω}{d θ})$ $m a = N \sin θ$ $N = 0 \Rightarrow a = 0$ $- \sin θ ω + \cos θ \frac{d ω}{d θ} = 0$ $\tan θ ω^{2} = ω \frac{d ω}{d θ} = \frac{g \sin θ (\cos^{2} θ - 2 \cos θ + 2)}{R {(1 + \sin^{2} θ)}^{2}}$ $\tan θ \frac{2 g (1 - \cos θ)}{R (1 + \sin^{2} θ)} = \frac{g \sin θ (\cos^{2} θ - 2 \cos θ + 2)}{{(1 + \sin^{2} θ)}^{2}}$ $\frac{2 (1 - \cos θ)}{\cos θ} = \frac{\cos^{2} θ - 2 \cos θ + 2}{1 + \sin^{2} θ}$ $\frac{2 - 2 \cos θ}{\cos θ} = \frac{\cos^{2} θ - 2 \cos θ + 2}{2 - \cos^{2} θ}$ $\cos^{3} θ - 6 \cos θ + 4 = 0$ $(\cos θ - 2) (\cos^{2} θ + 2 \cos θ - 2) = 0$ $\Rightarrow \cos θ = \sqrt{3} - 1$ $\Rightarrow θ = \cos^{- 1} (\sqrt{3} - 1) \approx 42.9 °$ $v = \sqrt{\frac{2 g R (1 - \cos θ) \cos^{2} θ}{2 - \cos^{2} θ}}$ $\Rightarrow v = (2 - \sqrt{3}) \sqrt{(\sqrt{3} + 1) g R} \approx 0.443 \sqrt{g R}$
	Commented by ajfour last updated on 25/Jun/21
	Thanks Sir, right answer!
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum