
Previous in Differential Equation Next in Differential Equation
Question Number 14444 by tawa tawa last updated on 31/May/17
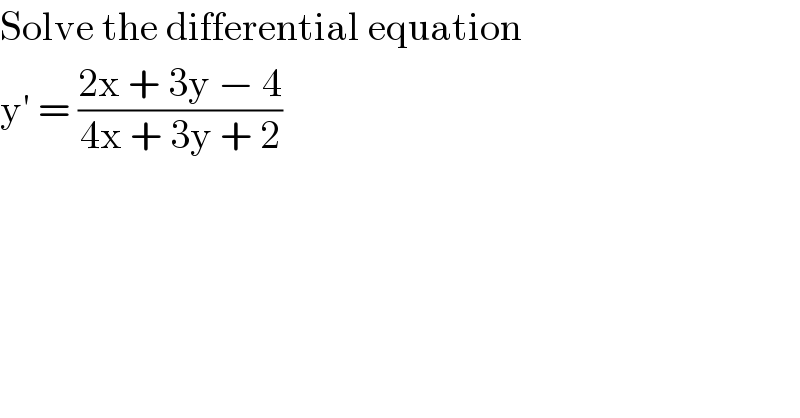
$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{differential}\:\mathrm{equation}\: \\ $$$$\mathrm{y}'\:=\:\frac{\mathrm{2x}\:+\:\mathrm{3y}\:−\:\mathrm{4}}{\mathrm{4x}\:+\:\mathrm{3y}\:+\:\mathrm{2}} \\ $$
Answered by mrW1 last updated on 31/May/17
![let x=u+p and y=v+q 2x+3y−4=2u+3v+2p+3q−4 4x+3y+2=4u+3v+4p+3q+2 let 2p+3q−4=0 ...(i) 4p+3q+2=0 ...(ii) (ii)−(i): 2p+6=0 ⇒p=−3 2(i)−(ii): 3q−10=0 ⇒q=((10)/3) ⇒x=u−3 ⇒y=v+((10)/3) dx=du dy=dv ⇒(dv/du)=((2u+3v)/(4u+3v)) let v=tu (dv/du)=t+u(dt/du) ⇒t+u(dt/du)=((2+3t)/(4+3t)) u(dt/du)=((2+3t)/(4+3t))−t=−((3t^2 −t−2)/(4+3t))=−(((3t+2)(t−1))/(3t+4)) ((3t+4)/((3t+2)(t−1)))dt=−(du/u) ((3t+2+2)/((3t+2)(t−1)))dt=−(du/u) [(3/5)((1/(t−1)))−(6/5)((1/(3t+2)))]dt=−(du/u) ∫[(3/5)((1/(t−1)))−(6/5)((1/(3t+2)))]dt=−∫(du/u) (3/5)∫(1/(t−1)) dt−(6/5)∫(1/(3t+2)) dt=−∫(du/u) (3/5)ln (t−1)−((18)/5)ln (3t+2)=−ln u+C_1 (3/5)ln ((v/u)−1)−((18)/5)ln (((3v)/u)+2)=−ln u+C_1 (3/5)ln (((y−((10)/3))/(x+3))−1)−((18)/5)ln (((3y−10)/(x+3))+2)=−ln (x+3)+C_1 (3/5)ln (((y−x−3)/(x+3)))−((18)/5)ln (((3y+2x−4)/(x+3)))=−ln (x+3)+C_1 3ln (((y−x−3)/(x+3)))+5ln (x+3)−18ln (((3y+2x−4)/(x+3)))=C 3ln (y−x−3)+20ln (x+3)−18ln (3y+2x−4)=C or (((y−x−3)^3 (x+3)^(20) )/((3y+2x−4)^(18) ))=C](Q14449.png)
$${let}\:{x}={u}+{p}\:{and}\:{y}={v}+{q} \\ $$$$\mathrm{2}{x}+\mathrm{3}{y}−\mathrm{4}=\mathrm{2}{u}+\mathrm{3}{v}+\mathrm{2}{p}+\mathrm{3}{q}−\mathrm{4} \\ $$$$\mathrm{4}{x}+\mathrm{3}{y}+\mathrm{2}=\mathrm{4}{u}+\mathrm{3}{v}+\mathrm{4}{p}+\mathrm{3}{q}+\mathrm{2} \\ $$$${let} \\ $$$$\mathrm{2}{p}+\mathrm{3}{q}−\mathrm{4}=\mathrm{0}\:\:\:...\left({i}\right) \\ $$$$\mathrm{4}{p}+\mathrm{3}{q}+\mathrm{2}=\mathrm{0}\:\:\:...\left({ii}\right) \\ $$$$\left({ii}\right)−\left({i}\right): \\ $$$$\mathrm{2}{p}+\mathrm{6}=\mathrm{0} \\ $$$$\Rightarrow{p}=−\mathrm{3} \\ $$$$\mathrm{2}\left({i}\right)−\left({ii}\right): \\ $$$$\mathrm{3}{q}−\mathrm{10}=\mathrm{0} \\ $$$$\Rightarrow{q}=\frac{\mathrm{10}}{\mathrm{3}} \\ $$$$\Rightarrow{x}={u}−\mathrm{3} \\ $$$$\Rightarrow{y}={v}+\frac{\mathrm{10}}{\mathrm{3}} \\ $$$${dx}={du} \\ $$$${dy}={dv} \\ $$$$\Rightarrow\frac{{dv}}{{du}}=\frac{\mathrm{2}{u}+\mathrm{3}{v}}{\mathrm{4}{u}+\mathrm{3}{v}} \\ $$$${let}\:{v}={tu} \\ $$$$\frac{{dv}}{{du}}={t}+{u}\frac{{dt}}{{du}} \\ $$$$\Rightarrow{t}+{u}\frac{{dt}}{{du}}=\frac{\mathrm{2}+\mathrm{3}{t}}{\mathrm{4}+\mathrm{3}{t}} \\ $$$${u}\frac{{dt}}{{du}}=\frac{\mathrm{2}+\mathrm{3}{t}}{\mathrm{4}+\mathrm{3}{t}}−{t}=−\frac{\mathrm{3}{t}^{\mathrm{2}} −{t}−\mathrm{2}}{\mathrm{4}+\mathrm{3}{t}}=−\frac{\left(\mathrm{3}{t}+\mathrm{2}\right)\left({t}−\mathrm{1}\right)}{\mathrm{3}{t}+\mathrm{4}} \\ $$$$\frac{\mathrm{3}{t}+\mathrm{4}}{\left(\mathrm{3}{t}+\mathrm{2}\right)\left({t}−\mathrm{1}\right)}{dt}=−\frac{{du}}{{u}} \\ $$$$\frac{\mathrm{3}{t}+\mathrm{2}+\mathrm{2}}{\left(\mathrm{3}{t}+\mathrm{2}\right)\left({t}−\mathrm{1}\right)}{dt}=−\frac{{du}}{{u}} \\ $$$$\left[\frac{\mathrm{3}}{\mathrm{5}}\left(\frac{\mathrm{1}}{{t}−\mathrm{1}}\right)−\frac{\mathrm{6}}{\mathrm{5}}\left(\frac{\mathrm{1}}{\mathrm{3}{t}+\mathrm{2}}\right)\right]{dt}=−\frac{{du}}{{u}} \\ $$$$\int\left[\frac{\mathrm{3}}{\mathrm{5}}\left(\frac{\mathrm{1}}{{t}−\mathrm{1}}\right)−\frac{\mathrm{6}}{\mathrm{5}}\left(\frac{\mathrm{1}}{\mathrm{3}{t}+\mathrm{2}}\right)\right]{dt}=−\int\frac{{du}}{{u}} \\ $$$$\frac{\mathrm{3}}{\mathrm{5}}\int\frac{\mathrm{1}}{{t}−\mathrm{1}}\:{dt}−\frac{\mathrm{6}}{\mathrm{5}}\int\frac{\mathrm{1}}{\mathrm{3}{t}+\mathrm{2}}\:{dt}=−\int\frac{{du}}{{u}} \\ $$$$\frac{\mathrm{3}}{\mathrm{5}}\mathrm{ln}\:\left({t}−\mathrm{1}\right)−\frac{\mathrm{18}}{\mathrm{5}}\mathrm{ln}\:\left(\mathrm{3}{t}+\mathrm{2}\right)=−\mathrm{ln}\:{u}+{C}_{\mathrm{1}} \\ $$$$\frac{\mathrm{3}}{\mathrm{5}}\mathrm{ln}\:\left(\frac{{v}}{{u}}−\mathrm{1}\right)−\frac{\mathrm{18}}{\mathrm{5}}\mathrm{ln}\:\left(\frac{\mathrm{3}{v}}{{u}}+\mathrm{2}\right)=−\mathrm{ln}\:{u}+{C}_{\mathrm{1}} \\ $$$$\frac{\mathrm{3}}{\mathrm{5}}\mathrm{ln}\:\left(\frac{{y}−\frac{\mathrm{10}}{\mathrm{3}}}{{x}+\mathrm{3}}−\mathrm{1}\right)−\frac{\mathrm{18}}{\mathrm{5}}\mathrm{ln}\:\left(\frac{\mathrm{3}{y}−\mathrm{10}}{{x}+\mathrm{3}}+\mathrm{2}\right)=−\mathrm{ln}\:\left({x}+\mathrm{3}\right)+{C}_{\mathrm{1}} \\ $$$$\frac{\mathrm{3}}{\mathrm{5}}\mathrm{ln}\:\left(\frac{{y}−{x}−\mathrm{3}}{{x}+\mathrm{3}}\right)−\frac{\mathrm{18}}{\mathrm{5}}\mathrm{ln}\:\left(\frac{\mathrm{3}{y}+\mathrm{2}{x}−\mathrm{4}}{{x}+\mathrm{3}}\right)=−\mathrm{ln}\:\left({x}+\mathrm{3}\right)+{C}_{\mathrm{1}} \\ $$$$\mathrm{3ln}\:\left(\frac{{y}−{x}−\mathrm{3}}{{x}+\mathrm{3}}\right)+\mathrm{5ln}\:\left({x}+\mathrm{3}\right)−\mathrm{18ln}\:\left(\frac{\mathrm{3}{y}+\mathrm{2}{x}−\mathrm{4}}{{x}+\mathrm{3}}\right)={C} \\ $$$$\mathrm{3ln}\:\left({y}−{x}−\mathrm{3}\right)+\mathrm{20ln}\:\left({x}+\mathrm{3}\right)−\mathrm{18ln}\:\left(\mathrm{3}{y}+\mathrm{2}{x}−\mathrm{4}\right)={C} \\ $$$${or} \\ $$$$\frac{\left({y}−{x}−\mathrm{3}\right)^{\mathrm{3}} \left({x}+\mathrm{3}\right)^{\mathrm{20}} }{\left(\mathrm{3}{y}+\mathrm{2}{x}−\mathrm{4}\right)^{\mathrm{18}} }={C} \\ $$
Commented by tawa tawa last updated on 31/May/17

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}.\:\mathrm{I}\:\mathrm{really}\:\mathrm{appreciate}\:\mathrm{your}\:\mathrm{effort}. \\ $$
