Question and Answers Forum

All Questions      Topic List

Vector Questions

Previous in All Question      Next in All Question      

Previous in Vector      Next in Vector      

Question Number 14587 by tawa tawa last updated on 02/Jun/17

Determine the roots of the equation x^3 + 64 = 0 in the polar form a + jb, Where a and b are real.

$$\mathrm{Determine}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation}\:\:\mathrm{x}^{\mathrm{3}} \:+\:\mathrm{64}\:=\:\mathrm{0}\:\:\mathrm{in}\:\mathrm{the}\:\mathrm{polar}\:\mathrm{form}\:\:\mathrm{a}\:+\:\mathrm{jb}, \\ $$$$\mathrm{Where}\:\:\mathrm{a}\:\:\mathrm{and}\:\:\mathrm{b}\:\:\mathrm{are}\:\mathrm{real}. \\ $$

Answered by mrW1 last updated on 03/Jun/17

x=r(cos θ+i sin θ) x^3 =r^3 (cos 3ϑ+i sin 3θ)=−64=64(−1+0 i) ⇒r^3 =64⇒r=^3 (√(64))=4 ⇒cos 3θ=−1 ⇒sin 3θ=0 ⇒3θ=(2n−1)π, n=1,2,3 ⇒θ_1 =(π/3) ⇒θ_2 =π ⇒θ_3 =((5π)/3) x_1 =4(cos (π/3)+i sin (π/3))=2+2(√3) i x_2 =4(cos π+i sin π)=−4+0 i x_3 =4(cos ((5π)/3)+i sin ((5π)/3))=2−2(√3) i

$${x}={r}\left(\mathrm{cos}\:\theta+{i}\:\mathrm{sin}\:\theta\right) \\ $$$${x}^{\mathrm{3}} ={r}^{\mathrm{3}} \left(\mathrm{cos}\:\mathrm{3}\vartheta+{i}\:\mathrm{sin}\:\mathrm{3}\theta\right)=−\mathrm{64}=\mathrm{64}\left(−\mathrm{1}+\mathrm{0}\:{i}\right) \\ $$$$\Rightarrow{r}^{\mathrm{3}} =\mathrm{64}\Rightarrow{r}=\:^{\mathrm{3}} \sqrt{\mathrm{64}}=\mathrm{4} \\ $$$$\Rightarrow\mathrm{cos}\:\mathrm{3}\theta=−\mathrm{1} \\ $$$$\Rightarrow\mathrm{sin}\:\mathrm{3}\theta=\mathrm{0} \\ $$$$\Rightarrow\mathrm{3}\theta=\left(\mathrm{2}{n}−\mathrm{1}\right)\pi,\:{n}=\mathrm{1},\mathrm{2},\mathrm{3} \\ $$$$\Rightarrow\theta_{\mathrm{1}} =\frac{\pi}{\mathrm{3}} \\ $$$$\Rightarrow\theta_{\mathrm{2}} =\pi \\ $$$$\Rightarrow\theta_{\mathrm{3}} =\frac{\mathrm{5}\pi}{\mathrm{3}} \\ $$$${x}_{\mathrm{1}} =\mathrm{4}\left(\mathrm{cos}\:\frac{\pi}{\mathrm{3}}+{i}\:\mathrm{sin}\:\frac{\pi}{\mathrm{3}}\right)=\mathrm{2}+\mathrm{2}\sqrt{\mathrm{3}}\:{i} \\ $$$${x}_{\mathrm{2}} =\mathrm{4}\left(\mathrm{cos}\:\pi+{i}\:\mathrm{sin}\:\pi\right)=−\mathrm{4}+\mathrm{0}\:{i} \\ $$$${x}_{\mathrm{3}} =\mathrm{4}\left(\mathrm{cos}\:\frac{\mathrm{5}\pi}{\mathrm{3}}+{i}\:\mathrm{sin}\:\frac{\mathrm{5}\pi}{\mathrm{3}}\right)=\mathrm{2}−\mathrm{2}\sqrt{\mathrm{3}}\:{i} \\ $$

Commented by RasheedSoomro last updated on 04/Jun/17

This short way is new for me!

$$\mathcal{T}{his}\:{short}\:{way}\:{is}\:{new}\:{for}\:{me}! \\ $$

Commented by mrW1 last updated on 03/Jun/17

or x^3 =−64 ((x/(−4)))^3 =1 ⇒(x/(−4))=1,ω,ω^2 ⇒x=−4,−4ω,−4ω^2

$${or} \\ $$$${x}^{\mathrm{3}} =−\mathrm{64} \\ $$$$\left(\frac{{x}}{−\mathrm{4}}\right)^{\mathrm{3}} =\mathrm{1} \\ $$$$\Rightarrow\frac{{x}}{−\mathrm{4}}=\mathrm{1},\omega,\omega^{\mathrm{2}} \\ $$$$\Rightarrow{x}=−\mathrm{4},−\mathrm{4}\omega,−\mathrm{4}\omega^{\mathrm{2}} \\ $$

Commented by tawa tawa last updated on 04/Jun/17

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Commented by tawa tawa last updated on 04/Jun/17

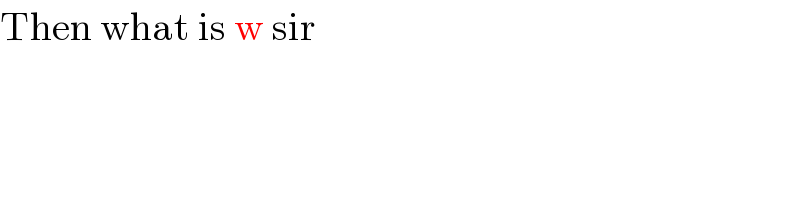
Then what is w sir

$$\mathrm{Then}\:\mathrm{what}\:\mathrm{is}\:\mathrm{w}\:\mathrm{sir} \\ $$

Commented by Tinkutara last updated on 04/Jun/17

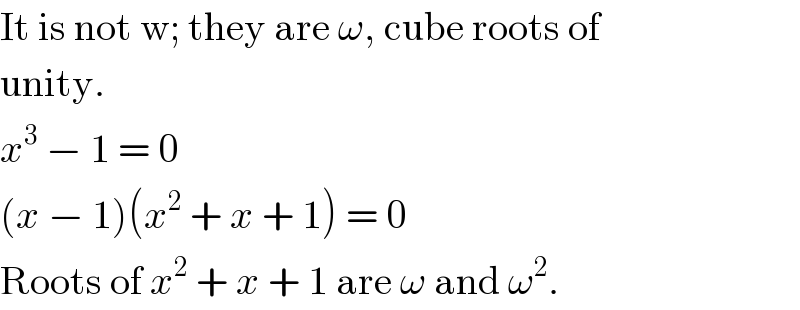
It is not w; they are ω, cube roots of unity. x^3 − 1 = 0 (x − 1)(x^2 + x + 1) = 0 Roots of x^2 + x + 1 are ω and ω^2 .

$$\mathrm{It}\:\mathrm{is}\:\mathrm{not}\:\mathrm{w};\:\mathrm{they}\:\mathrm{are}\:\omega,\:\mathrm{cube}\:\mathrm{roots}\:\mathrm{of} \\ $$$$\mathrm{unity}. \\ $$$${x}^{\mathrm{3}} \:−\:\mathrm{1}\:=\:\mathrm{0} \\ $$$$\left({x}\:−\:\mathrm{1}\right)\left({x}^{\mathrm{2}} \:+\:{x}\:+\:\mathrm{1}\right)\:=\:\mathrm{0} \\ $$$$\mathrm{Roots}\:\mathrm{of}\:{x}^{\mathrm{2}} \:+\:{x}\:+\:\mathrm{1}\:\mathrm{are}\:\omega\:\mathrm{and}\:\omega^{\mathrm{2}} . \\ $$

Commented by tawa tawa last updated on 04/Jun/17

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Commented by mrW1 last updated on 04/Jun/17

ω and ω^2 stand for −(1/2)±((√3)/2)i

$$\omega\:{and}\:\omega^{\mathrm{2}} \:{stand}\:{for}\:−\frac{\mathrm{1}}{\mathrm{2}}\pm\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}{i} \\ $$

Commented by tawa tawa last updated on 04/Jun/17

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Commented by tawa tawa last updated on 04/Jun/17

God bless you sir.

$$\mathrm{God}\:\mathrm{bless}\:\mathrm{you}\:\mathrm{sir}. \\ $$

Commented by arnabpapu550@gmail.com last updated on 13/Jun/17

To dear tawa. To find the qube root of 1 put x^3 =1 ∴ x^3 −1=0 or, (x−1)(x^2 +x+1)=0 ∴ (x−1)=0 ⇒x=1 or, (x^2 +x+1)=0 ∴ x=((−1±(√(1−4.1.1 )))/2)=((−1±i(√3))/2) It is denoted by ω and ω^2 . Where ω=((−1+i(√3))/2) ω^2 =((−1−i(√3))/2) [You may varify it] Properties: (a) ω^3 =1 ∴ ω^4 =ω^3 .ω=ω (b) 1+ω+ω^2 =0

$$\mathrm{To}\:\mathrm{dear}\:\mathrm{tawa}. \\ $$$$\mathrm{To}\:\mathrm{find}\:\mathrm{the}\:\mathrm{qube}\:\mathrm{root}\:\mathrm{of}\:\mathrm{1}\:\mathrm{put}\:\mathrm{x}^{\mathrm{3}} =\mathrm{1} \\ $$$$\therefore\:\mathrm{x}^{\mathrm{3}} −\mathrm{1}=\mathrm{0} \\ $$$$\mathrm{or},\:\left(\mathrm{x}−\mathrm{1}\right)\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\therefore\:\left(\mathrm{x}−\mathrm{1}\right)=\mathrm{0}\:\Rightarrow\mathrm{x}=\mathrm{1} \\ $$$$\mathrm{or},\:\left(\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)=\mathrm{0} \\ $$$$\therefore\:\:\mathrm{x}=\frac{−\mathrm{1}\pm\sqrt{\mathrm{1}−\mathrm{4}.\mathrm{1}.\mathrm{1}\:}}{\mathrm{2}}=\frac{−\mathrm{1}\pm\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\mathrm{It}\:\mathrm{is}\:\mathrm{denoted}\:\mathrm{by}\:\omega\:\mathrm{and}\:\omega^{\mathrm{2}} . \\ $$$$\mathrm{Where}\:\omega=\frac{−\mathrm{1}+\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}} \\ $$$$\omega^{\mathrm{2}} =\frac{−\mathrm{1}−\mathrm{i}\sqrt{\mathrm{3}}}{\mathrm{2}}\:\:\:\:\:\:\:\:\:\:\left[\mathrm{You}\:\mathrm{may}\:\mathrm{varify}\:\mathrm{it}\right] \\ $$$$\mathrm{Properties}: \\ $$$$\left(\mathrm{a}\right)\:\omega^{\mathrm{3}} =\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\therefore\:\omega^{\mathrm{4}} =\omega^{\mathrm{3}} .\omega=\omega \\ $$$$\left(\mathrm{b}\right)\:\mathrm{1}+\omega+\omega^{\mathrm{2}} =\mathrm{0} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com