{ ((x^2 +2y^2 +xy=37)),((y^2 +2x^2 +2xy=26)) :} ⇒ x^2 +y^2 =?


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 146936 by mathdanisur last updated on 16/Jul/21
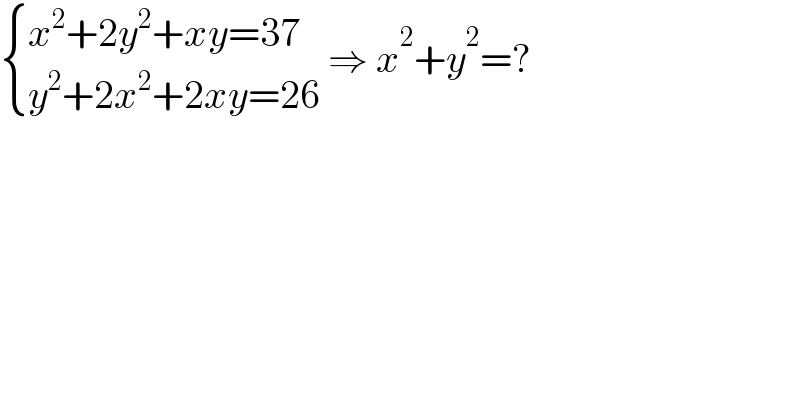
${ ((x^2 +2y^2 +xy=37)),((y^2 +2x^2 +2xy=26)) :} ⇒ x^2 +y^2 =?$
${\begin{cases} x^{2} + 2 y^{2} + x y = 37 \\ y^{2} + 2 x^{2} + 2 x y = 26 \end{cases} \Rightarrow x^{2} + y^{2} = ?$
	Answered by TheHoneyCat last updated on 16/Jul/21
	$let S= { ((x^2 +2y^2 +xy=37)),((y^2 +2x^2 +2xy=26)) :} ⇔ { ((x^2 +2y^2 +xy=37)),((−3y^2 =26−2×37)) :} ⇔ { ((x^2 +2y^2 +xy=37)),((y^2 =16)) :} ⇔ { ((x^2 +32+xy=37)),((y^2 =16)) :} ⇔ { ((x^2 +xy−5=0)),((y^2 =16)) :} since y^2 +4×5=36>0 x∈{((−y±(√(y^2 −4×5)))/2)} so: S⇔ { ((x∈{−y/2±3})),((y^2 =16)) :} S⇔ { ((x∈{−2±3,2±3})),((y^2 =16)) :} S⇒ { ((x^2 ∈{2^2 ±2×2×3+3^2 })),((y^2 =16)) :}I just developped (a±b)^2 thus S⇒ { ((x^2 ∈{13±12})),((y^2 =16)) :} so S⇒x^2 +y^2 ∈{17,41}_■ By the way (this has nothing to do with the question) those are two elipses so the number of solutions for (x,y) less (or equal) to 4 the two elispes beeing centered on (0,0) the set of solutions is symetrical that is to say that if (x,y) is solution (x,y) is too. you can then randomly try some values and notice that : (x,y)=(1,4) and (x,y)=(5,−4) are solutions so you have directly what you wanted this method is not as great as what I first did. because is relies on you making a lucky guess however if you have access to geogebra (or some equivalent) this makes the problem trivial plus, you can mix the methods. (for instance, once I have reached y^2 =16 with the first method, you might want to check for obvious values of x) I hope you find that note interesting :−)$
	$let S = {\begin{cases} x^{2} + 2 y^{2} + x y = 37 \\ y^{2} + 2 x^{2} + 2 x y = 26 \end{cases}$ $\Leftrightarrow {\begin{cases} x^{2} + 2 y^{2} + x y = 37 \\ - 3 y^{2} = 26 - 2 \times 37 \end{cases}$ $\Leftrightarrow {\begin{cases} x^{2} + 2 y^{2} + x y = 37 \\ y^{2} = 16 \end{cases}$ $\Leftrightarrow {\begin{cases} x^{2} + 32 + x y = 37 \\ y^{2} = 16 \end{cases}$ $\Leftrightarrow {\begin{cases} x^{2} + x y - 5 = 0 \\ y^{2} = 16 \end{cases}$ $since y^{2} + 4 \times 5 = 36 > 0 x \in {\frac{- y \pm \sqrt{y^{2} - 4 \times 5}}{2}}$ $so :$ $S \Leftrightarrow {\begin{cases} x \in {- y / 2 \pm 3} \\ y^{2} = 16 \end{cases}$ $S \Leftrightarrow {\begin{cases} x \in {- 2 \pm 3, 2 \pm 3} \\ y^{2} = 16 \end{cases}$ $S \Rightarrow {\begin{cases} x^{2} \in {2^{2} \pm 2 \times 2 \times 3 + 3^{2}} \\ y^{2} = 16 \end{cases} I j u s t d e v e l o p p e d {(a \pm b)}^{2}$ $thus S \Rightarrow {\begin{cases} x^{2} \in {13 \pm 12} \\ y^{2} = 16 \end{cases}$ $so S \Rightarrow x^{2} + y^{2} \in {17, 41}_{◼}$ $B y t h e w a y (t h i s h a s n o t h i n g t o d o w i t h t h e q u e s t i o n)$ $t h o s e a r e t w o e l i p s e s s o t h e n u m b e r o f s o l u t i o n s f o r (x, y) l e s s (o r e q u a l) t o 4$ $t h e t w o e l i s p e s b e e i n g c e n t e r e d o n (0, 0) t h e s e t o f s o l u t i o n s i s s y m e t r i c a l$ $that is to say that if (x, y) is solution (x, y) is too .$ $y o u c a n t h e n r a n d o m l y t r y s o m e v a l u e s a n d n o t i c e t h a t :$ $(x, y) = (1, 4) a n d (x, y) = (5, - 4) a r e s o l u t i o n s$ $s o y o u h a v e d i r e c t l y w h a t y o u w a n t e d$ $t h i s m e t h o d i s n o t a s g r e a t a s w h a t I f i r s t d i d .$ $b e c a u s e i s r e l i e s o n y o u m a k i n g a l u c k y g u e s s$ $h o w e v e r i f y o u h a v e a c c e s s t o g e o g e b r a (o r s o m e e q u i v a l e n t) t h i s m a k e s t h e p r o b l e m t r i v i a l$ $p l u s, y o u c a n m i x t h e m e t h o d s .$ $(f o r i n s t a n c e, o n c e I h a v e r e a c h e d y^{2} = 16 w i t h t h e f i r s t m e t h o d, y o u m i g h t w a n t t o c h e c k f o r o b v i o u s v a l u e s o f x)$ $I h o p e y o u f i n d t h a t n o t e i n t e r e s t i n g$ $: -)$
	Commented by mathdanisur last updated on 16/Jul/21

	$t h a n k y o u S e r$
	Answered by mr W last updated on 16/Jul/21

	$l e t x^{2} + y^{2} = r^{2}$ $\Rightarrow x = r \cos θ, y = r \sin θ$ $r^{2} + r^{2} \sin^{2} θ + r^{2} \cos θ \sin θ = 37 . . . (i)$ $r^{2} + r^{2} \cos^{2} θ + 2 r^{2} \cos θ \sin θ = 26 . . . (i i)$ $(i) + (i i) :$ $3 r^{2} + 3 r^{2} \cos θ \sin θ = 63$ $r^{2} + r^{2} \frac{\sin 2 θ}{2} = 21 . . . (i i i)$ $\Rightarrow \sin 2 θ = \frac{42}{r^{2}} - 2$ $(i i) - (i) :$ $r^{2} \cos 2 θ + r^{2} \frac{\sin 2 θ}{2} = - 11 . . . (i v)$ $(i v) - (i i i) :$ $r^{2} \cos 2 θ - r^{2} = - 32$ $\Rightarrow \cos 2 θ = 1 - \frac{32}{r^{2}}$ ${(\frac{42}{r^{2}} - 2)}^{2} + {(1 - \frac{32}{r^{2}})}^{2} = 1$ ${(42 - 2 r^{2})}^{2} + {(r^{2} - 32)}^{2} = r^{4}$ $r^{4} - 58 r^{2} + 697 = 0$ $\Rightarrow r^{2} = \frac{58 \pm \sqrt{58^{2} - 4 \times 697}}{2} = \frac{58 \pm 24}{2} = 41, 17$ $\Rightarrow x^{2} + y^{2} = 17 o r 41$
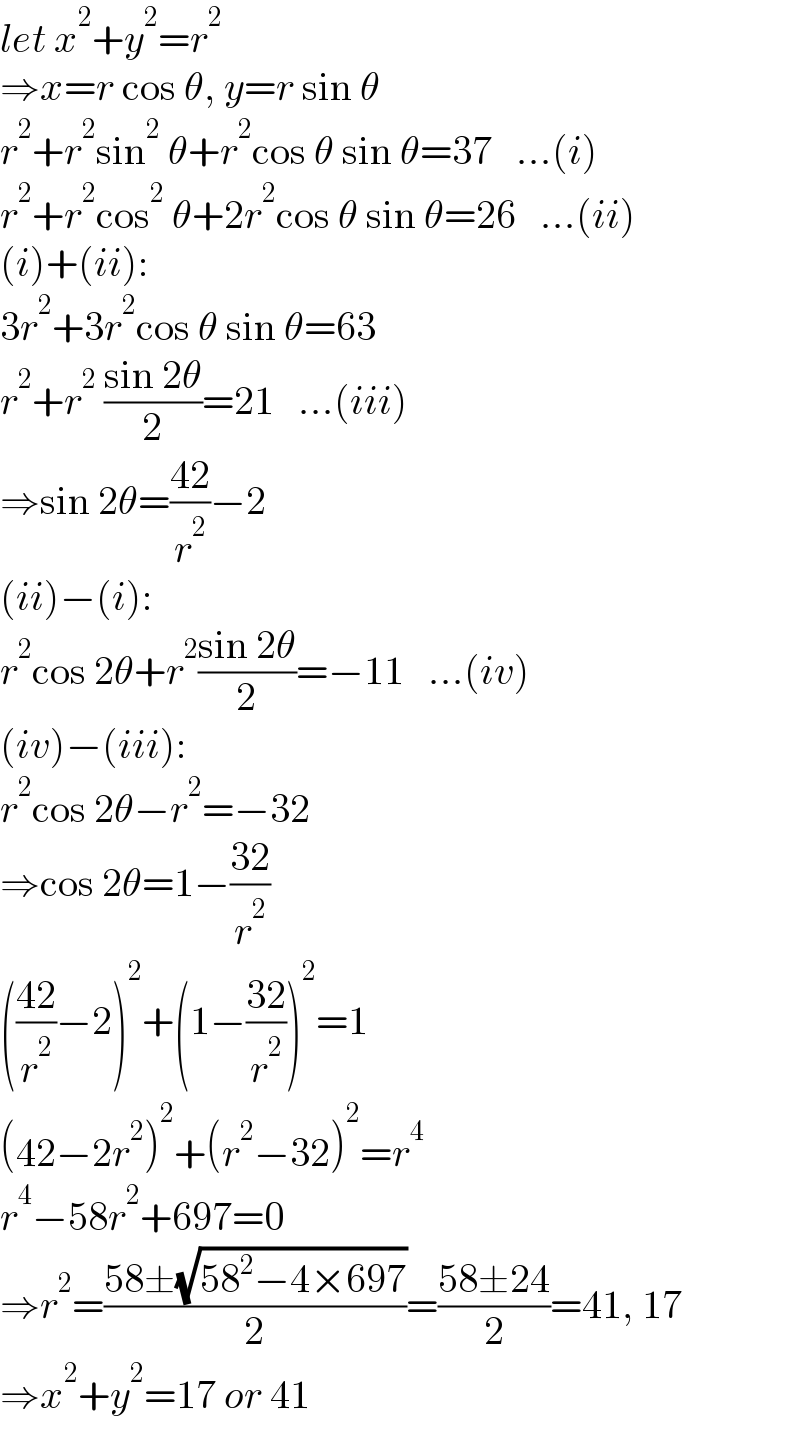
	Commented by behi834171 last updated on 16/Jul/21

	$v e r y n i c e d e a r m a s t e r!$
	Commented by mathdanisur last updated on 16/Jul/21

	$t h a n k y o u S e r c o o l$
	Commented by Tawa11 last updated on 23/Jul/21

	$great$
	Answered by behi834171 last updated on 17/Jul/21
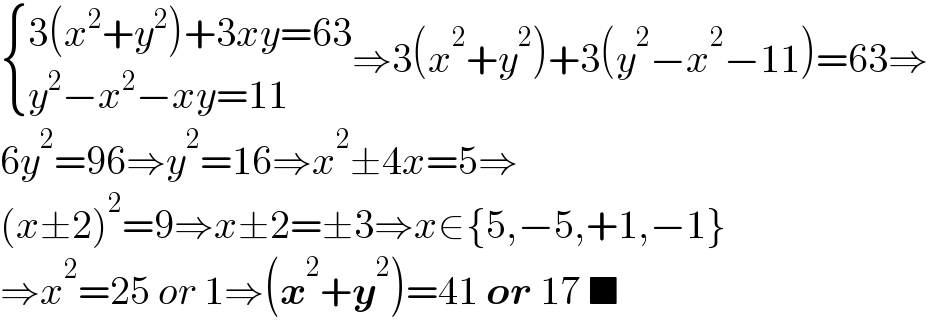
	${ ((3(x^2 +y^2 )+3xy=63)),((y^2 −x^2 −xy=11)) :}⇒3(x^2 +y^2 )+3(y^2 −x^2 −11)=63⇒ 6y^2 =96⇒y^2 =16⇒x^2 ±4x=5⇒ (x±2)^2 =9⇒x±2=±3⇒x∈{5,−5,+1,−1} ⇒x^2 =25 or 1⇒(x^2 +y^2 )=41 or 17 ■$
	${\begin{cases} 3 (x^{2} + y^{2}) + 3 x y = 63 \\ y^{2} - x^{2} - x y = 11 \end{cases} \Rightarrow 3 (x^{2} + y^{2}) + 3 (y^{2} - x^{2} - 11) = 63 \Rightarrow$ $6 y^{2} = 96 \Rightarrow y^{2} = 16 \Rightarrow x^{2} \pm 4 x = 5 \Rightarrow$ ${(x \pm 2)}^{2} = 9 \Rightarrow x \pm 2 = \pm 3 \Rightarrow x \in {5, - 5, + 1, - 1}$ $\Rightarrow x^{2} = 25 o r 1 \Rightarrow (x^{2} + y^{2}) = 41 o r 17 ◼$
	Commented by mathdanisur last updated on 16/Jul/21

	$t h a n k s S e r c o o l$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum