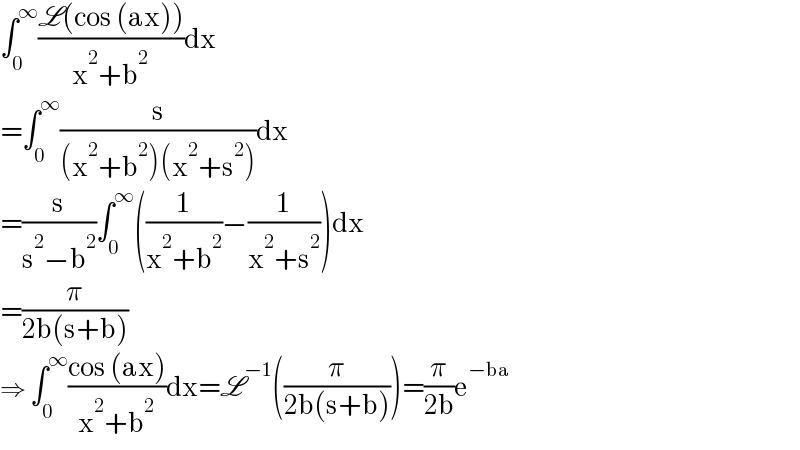Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 147322 by Gbenga last updated on 19/Jul/21

Commented by Mrsof last updated on 19/Jul/21

$$I={\int }_0^{\mathrm{\infty }}\frac{cos\left(ax\right)}{x^2+b^2}dx{=}_{x=z}2I={\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}\frac{cos\left(az\right)}{z^2+b^2}dz$$$$$$$$2I=Re\left({\int }_{-\mathrm{\infty }}^{\mathrm{\infty }}\frac{e^{aiz}}{(z-bi)(z+bi)}dz\right)$$$$$$$$thefunctionhasaresideoofz=bi$$$$$$$$Res(f,bi)={lim}_{z\to bi}(z-bi)\times \frac{e^{aiz}}{(z-bi)(z+bi)}=\frac{e^{-ab}}{2bi}$$$$$$$$2I=2\pi i\left(Res(f,bi)\right)\Rightarrow I=\pi i\times \frac{e^{-ab}}{2bi}=\frac{\pi e^{-ab}}{2b}$$$$$$$$⟨M.T⟩$$

Commented by Gbenga last updated on 19/Jul/21

$$thanks$$

Commented by Mrsof last updated on 20/Jul/21

$$youarewelcome$$

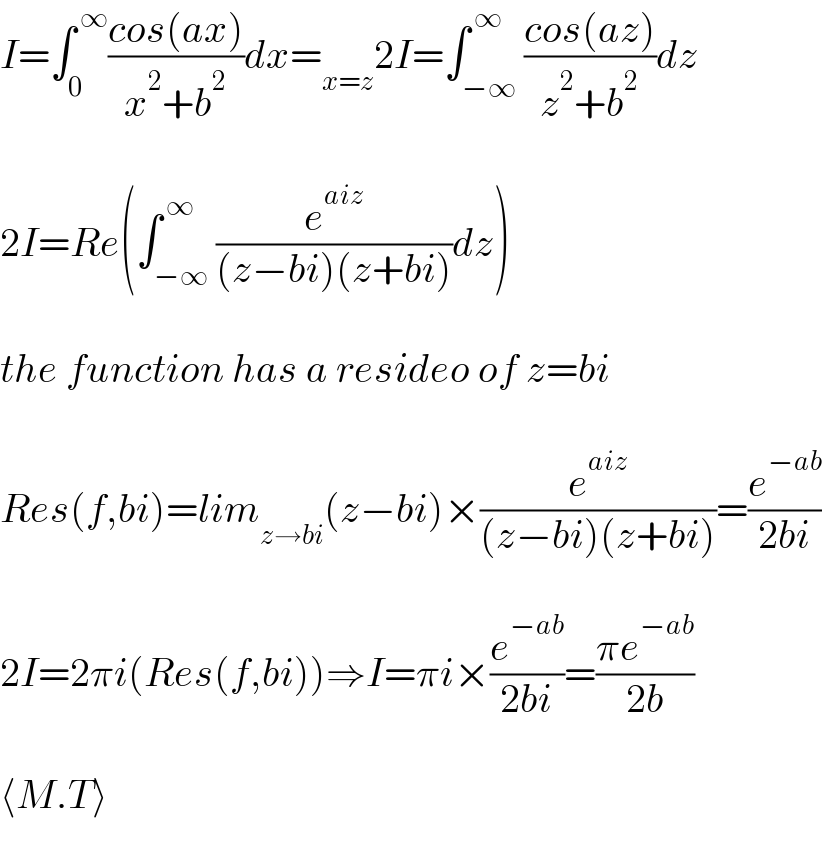
Answered by qaz last updated on 20/Jul/21

$${\int }_0^{\mathrm{\infty }}\frac{\mathcal{L}\left(\cos \left(\mathrm{ax}\right)\right)}{{\mathrm{x}}^2+{\mathrm{b}}^2}\mathrm{dx}$$$$={\int }_0^{\mathrm{\infty }}\frac{\mathrm{s}}{({\mathrm{x}}^2+{\mathrm{b}}^2)({\mathrm{x}}^2+{\mathrm{s}}^2)}\mathrm{dx}$$$$=\frac{\mathrm{s}}{{\mathrm{s}}^2-{\mathrm{b}}^2}{\int }_0^{\mathrm{\infty }}(\frac{1}{{\mathrm{x}}^2+{\mathrm{b}}^2}-\frac{1}{{\mathrm{x}}^2+{\mathrm{s}}^2})\mathrm{dx}$$$$=\frac{\pi }{2\mathrm{b}(\mathrm{s}+\mathrm{b})}$$$$\Rightarrow {\int }_0^{\mathrm{\infty }}\frac{\cos \left(\mathrm{ax}\right)}{{\mathrm{x}}^2+{\mathrm{b}}^2}\mathrm{dx}={\mathcal{L}}^{-1}\left(\frac{\pi }{2\mathrm{b}(\mathrm{s}+\mathrm{b})}\right)=\frac{\pi }{2\mathrm{b}}{\mathrm{e}}^{-\mathrm{ba}}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com