Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 14742 by Mr Chheang Chantria last updated on 04/Jun/17

$$\mathit{x}+\mathit{y}=\frac{1}{8}$$$$\sqrt[4]{\mathit{x}}+\sqrt[4]{\mathit{y}}=1$$$$\mathit{s}\mathit{o}\mathit{l}\mathit{v}\mathit{e}\mathit{s}\mathit{y}\mathit{s}\mathit{t}\mathit{e}\mathit{m}$$

Commented by Tinkutara last updated on 04/Jun/17

$$\mathrm{It}\mathrm{is}\mathrm{easy}\mathrm{to}\mathrm{verify}\mathrm{that}\frac{1}{16}+\frac{1}{16}=\frac{1}{8}$$$$\mathrm{and}\sqrt[4]{\frac{1}{16}}+\sqrt[4]{\frac{1}{16}}=1$$$$\mathrm{Hence}x=y=\frac{1}{16}$$

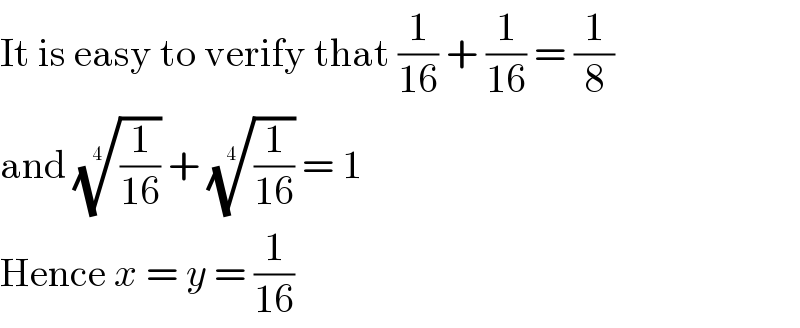
Commented by RasheedSoomro last updated on 04/Jun/17

$$\mathrm{Let}\sqrt[4]{\mathit{x}}=\mathrm{p}\mathrm{and}\sqrt[4]{y}=\mathrm{q}$$$$\Rightarrow \{\begin{array}{l}{\mathrm{p}}^4+{\mathrm{q}}^4=1/8\\ \mathrm{p}+\mathrm{q}=1\end{array}$$$$\Rightarrow \frac{{\mathrm{p}}^4+{\mathrm{q}}^4}{\mathrm{p}+\mathrm{q}}=\frac{1}{8}\wedge ({\mathrm{p}}^4+{\mathrm{q}}^4)(\mathrm{p}+\mathrm{q})=\frac{1}{8}$$$$\Rightarrow \frac{{\mathrm{p}}^4+{\mathrm{q}}^4}{\mathrm{p}+\mathrm{q}}-({\mathrm{p}}^4+{\mathrm{q}}^4)(\mathrm{p}+\mathrm{q})=0$$$$\Rightarrow ({\mathrm{p}}^4+{\mathrm{q}}^4)\{\frac{1}{\mathrm{p}+\mathrm{q}}-(\mathrm{p}+\mathrm{q})\}=0$$$${\mathrm{p}}^4+{\mathrm{q}}^4=0\vee \mathrm{p}+\mathrm{q}-\frac{1}{\mathrm{p}+\mathrm{q}}=0$$$${\mathrm{p}}^4=-{\mathrm{q}}^4\vee \mathrm{p}+\mathrm{q}=\frac{1}{\mathrm{p}+\mathrm{q}}$$$$\mathrm{x}=-\mathrm{y}\vee {(\mathrm{p}+\mathrm{q})}^2=1$$$$\mathrm{p}+\mathrm{q}=\pm 1$$$$\mathrm{p}+\mathrm{q}=1\mathrm{is}\mathrm{what}\mathrm{we}\mathrm{have}\mathrm{been}\mathrm{given}!\mathrm{hahahaa}..$$$$\mathrm{I}\mathrm{reached}\mathrm{at}\mathrm{the}\mathrm{starting}\mathrm{point}\mathrm{again}!!!$$$$\mathrm{p}+\mathrm{q}=-1\mathrm{is}\mathrm{extraneous}(?)$$$$$$$$\mathrm{Oh}\mathrm{no}{\mathrm{I}}^{\prime }\mathrm{ve}\mathrm{gained}\mathrm{something}\mathrm{x}=-\mathrm{y}$$$$\mathrm{But}\mathrm{this}\mathrm{is}\mathrm{again}\mathrm{in}\mathrm{contradiction}\mathrm{to}\mathrm{the}\mathrm{given}$$$$(\mathrm{x}+\mathrm{y}=1/8)$$$$!!!$$$$\mathrm{Continue}$$

Commented by prakash jain last updated on 04/Jun/17

$$\sqrt[4]{x}=u$$$$\sqrt[4]{y}=v$$$$u^4+v^4=\frac{1}{8}$$$$u+v=1$$$$u^2+v^2+2uv=1$$$$u^2+v^2=1-2uv$$$$u^4+v^4+2u^2{v}^2={(1-2uv)}^2$$$$\frac{1}{8}+2u^2{v}^2=1-4uv+4u^2{v}^2$$$$1+16v^2{u}^2=8-32uv+32u^2{v}^2$$$$16u^2{v}^2-32uv+7=0$$$$(4uv-7)(4uv-1)=0$$$$uv=\frac{1}{4}oruv=\frac{7}{4}$$$$uv=\frac{1}{4}$$$$u+v=1$$$${(u-v)}^2={(u+v)}^2-4uv=1-1=0$$$$u=v=\frac{1}{2}\Rightarrow x=\frac{1}{16},y=\frac{1}{16}$$$$uv=\frac{7}{4}$$$${(u-v)}^2=1-7=-6$$$$u-v=\pm i\sqrt{6}$$$$u=\frac{1\pm i\sqrt{6}}{2},v=\frac{1\mp i\sqrt{6}}{2}$$$$x=\frac{1\pm 20i\sqrt{6}}{16},y=\frac{1\mp 20i\sqrt{6}}{16}$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com