
Question and Answers Forum
Question Number 147711 by lapache last updated on 22/Jul/21
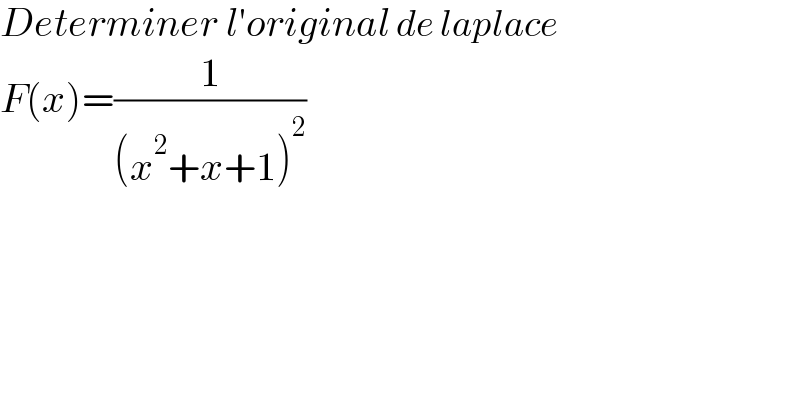
Answered by Olaf_Thorendsen last updated on 23/Jul/21
![G(x) = F(x−(1/2)) = (1/((x^2 +(3/4))^2 )) H(x) = G(((√3)/2)x) = ((16)/9).(1/((x^2 +1)^2 )) H(x) = ((16)/9)[−(1/(4(x+i)^2 ))−(1/(4(x−i)^2 ))+(i/(4(x+i)))−(i/(4(x−i)))] H(x) = (4/9)[−(1/((x+i)^2 ))−(1/((x−i)^2 ))+(i/(x+i))−(i/(x−i))] L^(−1) ((1/(x−a))) = e^(at) et L^(−1) ((1/((x−a)^2 ))) = te^(at) L^(−1) {H}(t) = (4/9)[−te^(−it) −te^(it) +ie^(−it) −ie^(it) ] L^(−1) {H}(t) = (4/9)[−2tcost+2sint] L^(−1) {H}(t) = (8/9)(sint−tcost) L^(−1) {G(x)}(t) = L^(−1) {H((x/((√3)/2)))}(t) L^(−1) {G(x)}(t) = ((√3)/2)((1/((√3)/2))L^(−1) {H((x/((√3)/2)))}(t) ) L^(−1) {G(x)}(t) = ((√3)/2)(L^(−1) {H}(((√3)/2)t)) L^(−1) {G}(t) = (4/(3(√3)))(sin(((√3)/2)t)−((√3)/2)tcos(((√3)/2)t)) L^(−1) {F(x)}(t) = L^(−1) {G(x+(1/2))}(t) L^(−1) {F(x)}(t) = e^(−(1/2)t) L^(−1) {G(x)}(t) L^(−1) {F}(t) = (4/(3(√3)))e^(−(t/2)) (sin(((√3)/2)t)−((√3)/2)tcos(((√3)/2)t))](Q147723.png)
| ||
Question and Answers Forum | ||
Question Number 147711 by lapache last updated on 22/Jul/21 | ||
 | ||
Answered by Olaf_Thorendsen last updated on 23/Jul/21 | ||
![G(x) = F(x−(1/2)) = (1/((x^2 +(3/4))^2 )) H(x) = G(((√3)/2)x) = ((16)/9).(1/((x^2 +1)^2 )) H(x) = ((16)/9)[−(1/(4(x+i)^2 ))−(1/(4(x−i)^2 ))+(i/(4(x+i)))−(i/(4(x−i)))] H(x) = (4/9)[−(1/((x+i)^2 ))−(1/((x−i)^2 ))+(i/(x+i))−(i/(x−i))] L^(−1) ((1/(x−a))) = e^(at) et L^(−1) ((1/((x−a)^2 ))) = te^(at) L^(−1) {H}(t) = (4/9)[−te^(−it) −te^(it) +ie^(−it) −ie^(it) ] L^(−1) {H}(t) = (4/9)[−2tcost+2sint] L^(−1) {H}(t) = (8/9)(sint−tcost) L^(−1) {G(x)}(t) = L^(−1) {H((x/((√3)/2)))}(t) L^(−1) {G(x)}(t) = ((√3)/2)((1/((√3)/2))L^(−1) {H((x/((√3)/2)))}(t) ) L^(−1) {G(x)}(t) = ((√3)/2)(L^(−1) {H}(((√3)/2)t)) L^(−1) {G}(t) = (4/(3(√3)))(sin(((√3)/2)t)−((√3)/2)tcos(((√3)/2)t)) L^(−1) {F(x)}(t) = L^(−1) {G(x+(1/2))}(t) L^(−1) {F(x)}(t) = e^(−(1/2)t) L^(−1) {G(x)}(t) L^(−1) {F}(t) = (4/(3(√3)))e^(−(t/2)) (sin(((√3)/2)t)−((√3)/2)tcos(((√3)/2)t))](Q147723.png) | ||
| ||