Question and Answers Forum

All Questions      Topic List

Others Questions

Previous in All Question      Next in All Question      

Previous in Others      Next in Others      

Question Number 149100 by Naser last updated on 02/Aug/21

Answered by Kamel last updated on 02/Aug/21

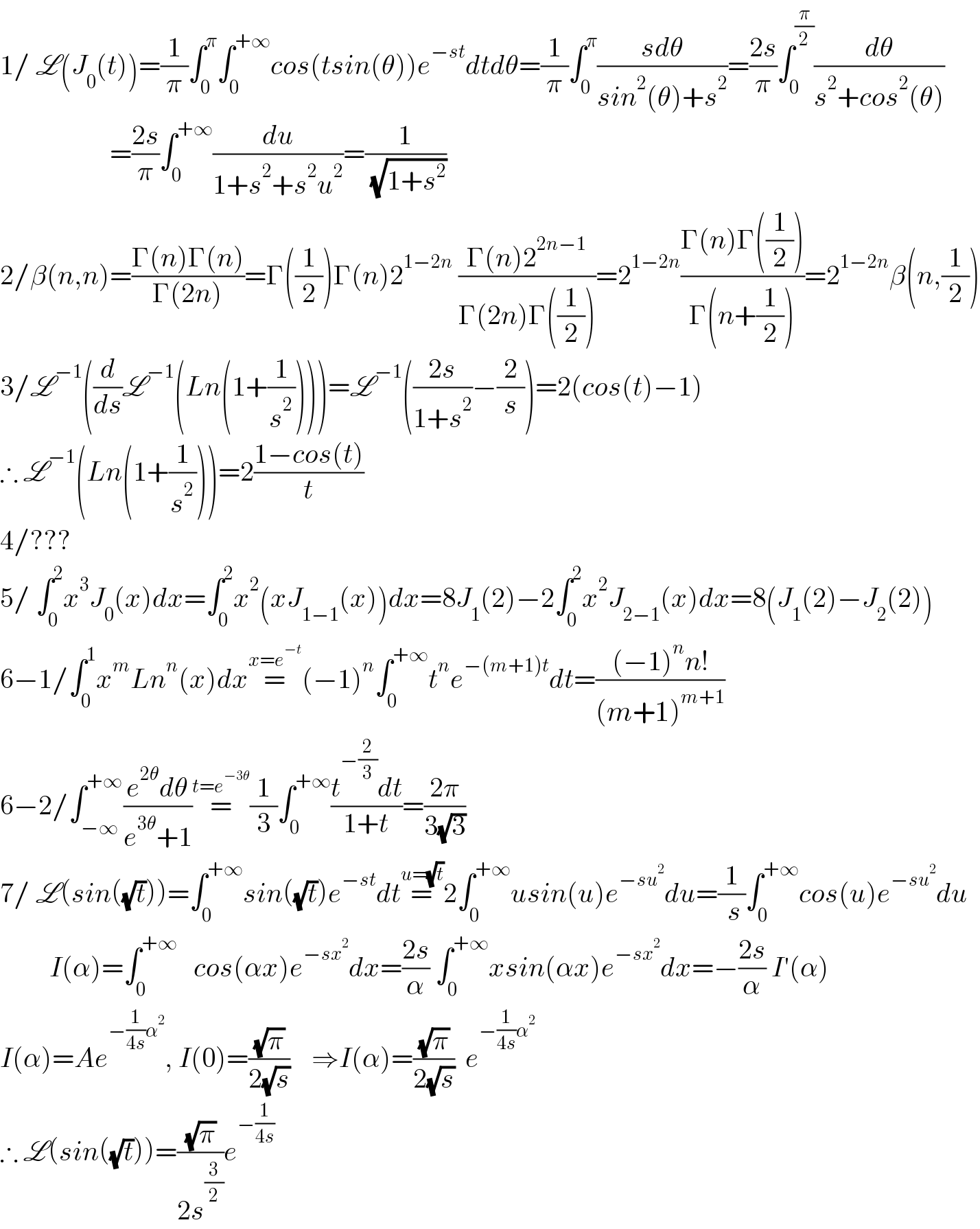
1/ L(J_0 (t))=(1/π)∫_0 ^π ∫_0 ^(+∞) cos(tsin(θ))e^(−st) dtdθ=(1/π)∫_0 ^π ((sdθ)/(sin^2 (θ)+s^2 ))=((2s)/π)∫_0 ^(π/2) (dθ/(s^2 +cos^2 (θ))) =((2s)/π)∫_0 ^(+∞) (du/(1+s^2 +s^2 u^2 ))=(1/( (√(1+s^2 )))) 2/β(n,n)=((Γ(n)Γ(n))/(Γ(2n)))=Γ((1/2))Γ(n)2^(1−2n) ((Γ(n)2^(2n−1) )/(Γ(2n)Γ((1/2))))=2^(1−2n) ((Γ(n)Γ((1/2)))/(Γ(n+(1/2))))=2^(1−2n) β(n,(1/2)) 3/L^(−1) ((d/ds)L^(−1) (Ln(1+(1/s^2 ))))=L^(−1) (((2s)/(1+s^2 ))−(2/s))=2(cos(t)−1) ∴ L^(−1) (Ln(1+(1/s^2 )))=2((1−cos(t))/t) 4/??? 5/ ∫_0 ^2 x^3 J_0 (x)dx=∫_0 ^2 x^2 (xJ_(1−1) (x))dx=8J_1 (2)−2∫_0 ^2 x^2 J_(2−1) (x)dx=8(J_1 (2)−J_2 (2)) 6−1/∫_0 ^1 x^m Ln^n (x)dx=^(x=e^(−t) ) (−1)^n ∫_0 ^(+∞) t^n e^(−(m+1)t) dt=(((−1)^n n!)/((m+1)^(m+1) )) 6−2/∫_(−∞) ^(+∞) ((e^(2θ) dθ)/(e^(3θ) +1))=^(t=e^(−3θ) ) (1/3)∫_0 ^(+∞) ((t^(−(2/3)) dt)/(1+t))=((2π)/(3(√3))) 7/ L(sin((√t)))=∫_0 ^(+∞) sin((√t))e^(−st) dt=^(u=(√t)) 2∫_0 ^(+∞) usin(u)e^(−su^2 ) du=(1/( s))∫_0 ^(+∞) cos(u)e^(−su^2 ) du I(α)=∫_0 ^(+∞) cos(αx)e^(−sx^2 ) dx=((2s)/α) ∫_0 ^(+∞) xsin(αx)e^(−sx^2 ) dx=−((2s)/α) I′(α) I(α)=Ae^(−(1/(4s))α^2 ) , I(0)=((√π)/(2(√s))) ⇒I(α)=((√π)/(2(√s))) e^(−(1/(4s))α^2 ) ∴ L(sin((√t)))=((√π)/(2s^(3/2) ))e^(−(1/(4s)))

$$\mathrm{1}/\:\mathscr{L}\left({J}_{\mathrm{0}} \left({t}\right)\right)=\frac{\mathrm{1}}{\pi}\int_{\mathrm{0}} ^{\pi} \int_{\mathrm{0}} ^{+\infty} {cos}\left({tsin}\left(\theta\right)\right){e}^{−{st}} {dtd}\theta=\frac{\mathrm{1}}{\pi}\int_{\mathrm{0}} ^{\pi} \frac{{sd}\theta}{{sin}^{\mathrm{2}} \left(\theta\right)+{s}^{\mathrm{2}} }=\frac{\mathrm{2}{s}}{\pi}\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{d}\theta}{{s}^{\mathrm{2}} +{cos}^{\mathrm{2}} \left(\theta\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\frac{\mathrm{2}{s}}{\pi}\int_{\mathrm{0}} ^{+\infty} \frac{{du}}{\mathrm{1}+{s}^{\mathrm{2}} +{s}^{\mathrm{2}} {u}^{\mathrm{2}} }=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{s}^{\mathrm{2}} }} \\ $$$$\mathrm{2}/\beta\left({n},{n}\right)=\frac{\Gamma\left({n}\right)\Gamma\left({n}\right)}{\Gamma\left(\mathrm{2}{n}\right)}=\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)\Gamma\left({n}\right)\mathrm{2}^{\mathrm{1}−\mathrm{2}{n}} \:\frac{\Gamma\left({n}\right)\mathrm{2}^{\mathrm{2}{n}−\mathrm{1}} }{\Gamma\left(\mathrm{2}{n}\right)\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}=\mathrm{2}^{\mathrm{1}−\mathrm{2}{n}} \frac{\Gamma\left({n}\right)\Gamma\left(\frac{\mathrm{1}}{\mathrm{2}}\right)}{\Gamma\left({n}+\frac{\mathrm{1}}{\mathrm{2}}\right)}=\mathrm{2}^{\mathrm{1}−\mathrm{2}{n}} \beta\left({n},\frac{\mathrm{1}}{\mathrm{2}}\right) \\ $$$$\mathrm{3}/\mathscr{L}^{−\mathrm{1}} \left(\frac{{d}}{{ds}}\mathscr{L}^{−\mathrm{1}} \left({Ln}\left(\mathrm{1}+\frac{\mathrm{1}}{{s}^{\mathrm{2}} }\right)\right)\right)=\mathscr{L}^{−\mathrm{1}} \left(\frac{\mathrm{2}{s}}{\mathrm{1}+{s}^{\mathrm{2}} }−\frac{\mathrm{2}}{{s}}\right)=\mathrm{2}\left({cos}\left({t}\right)−\mathrm{1}\right) \\ $$$$\therefore\:\mathscr{L}^{−\mathrm{1}} \left({Ln}\left(\mathrm{1}+\frac{\mathrm{1}}{{s}^{\mathrm{2}} }\right)\right)=\mathrm{2}\frac{\mathrm{1}−{cos}\left({t}\right)}{{t}} \\ $$$$\mathrm{4}/??? \\ $$$$\mathrm{5}/\:\int_{\mathrm{0}} ^{\mathrm{2}} {x}^{\mathrm{3}} {J}_{\mathrm{0}} \left({x}\right){dx}=\int_{\mathrm{0}} ^{\mathrm{2}} {x}^{\mathrm{2}} \left({xJ}_{\mathrm{1}−\mathrm{1}} \left({x}\right)\right){dx}=\mathrm{8}{J}_{\mathrm{1}} \left(\mathrm{2}\right)−\mathrm{2}\int_{\mathrm{0}} ^{\mathrm{2}} {x}^{\mathrm{2}} {J}_{\mathrm{2}−\mathrm{1}} \left({x}\right){dx}=\mathrm{8}\left({J}_{\mathrm{1}} \left(\mathrm{2}\right)−{J}_{\mathrm{2}} \left(\mathrm{2}\right)\right) \\ $$$$\mathrm{6}−\mathrm{1}/\int_{\mathrm{0}} ^{\mathrm{1}} {x}^{{m}} {Ln}^{{n}} \left({x}\right){dx}\overset{{x}={e}^{−{t}} } {=}\left(−\mathrm{1}\right)^{{n}} \int_{\mathrm{0}} ^{+\infty} {t}^{{n}} {e}^{−\left({m}+\mathrm{1}\right){t}} {dt}=\frac{\left(−\mathrm{1}\right)^{{n}} {n}!}{\left({m}+\mathrm{1}\right)^{{m}+\mathrm{1}} } \\ $$$$\mathrm{6}−\mathrm{2}/\int_{−\infty} ^{+\infty} \frac{{e}^{\mathrm{2}\theta} {d}\theta}{{e}^{\mathrm{3}\theta} +\mathrm{1}}\overset{{t}={e}^{−\mathrm{3}\theta} } {=}\frac{\mathrm{1}}{\mathrm{3}}\int_{\mathrm{0}} ^{+\infty} \frac{{t}^{−\frac{\mathrm{2}}{\mathrm{3}}} {dt}}{\mathrm{1}+{t}}=\frac{\mathrm{2}\pi}{\mathrm{3}\sqrt{\mathrm{3}}} \\ $$$$\mathrm{7}/\:\mathscr{L}\left({sin}\left(\sqrt{{t}}\right)\right)=\int_{\mathrm{0}} ^{+\infty} {sin}\left(\sqrt{{t}}\right){e}^{−{st}} {dt}\overset{{u}=\sqrt{{t}}} {=}\mathrm{2}\int_{\mathrm{0}} ^{+\infty} {usin}\left({u}\right){e}^{−{su}^{\mathrm{2}} } {du}=\frac{\mathrm{1}}{\:{s}}\int_{\mathrm{0}} ^{+\infty} {cos}\left({u}\right){e}^{−{su}^{\mathrm{2}} } {du} \\ $$$$\:\:\:\:\:\:\:\:\:{I}\left(\alpha\right)=\int_{\mathrm{0}} ^{+\infty} \:\:\:{cos}\left(\alpha{x}\right){e}^{−{sx}^{\mathrm{2}} } {dx}=\frac{\mathrm{2}{s}}{\alpha}\:\int_{\mathrm{0}} ^{+\infty} {xsin}\left(\alpha{x}\right){e}^{−{sx}^{\mathrm{2}} } {dx}=−\frac{\mathrm{2}{s}}{\alpha}\:{I}'\left(\alpha\right) \\ $$$${I}\left(\alpha\right)={Ae}^{−\frac{\mathrm{1}}{\mathrm{4}{s}}\alpha^{\mathrm{2}} } ,\:{I}\left(\mathrm{0}\right)=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{{s}}}\:\:\:\:\Rightarrow{I}\left(\alpha\right)=\frac{\sqrt{\pi}}{\mathrm{2}\sqrt{{s}}}\:\:{e}^{−\frac{\mathrm{1}}{\mathrm{4}{s}}\alpha^{\mathrm{2}} } \\ $$$$\therefore\:\mathscr{L}\left({sin}\left(\sqrt{{t}}\right)\right)=\frac{\sqrt{\pi}}{\mathrm{2}{s}^{\frac{\mathrm{3}}{\mathrm{2}}} }{e}^{−\frac{\mathrm{1}}{\mathrm{4}{s}}} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com