
Question and Answers Forum
Question Number 149113 by bramlexs22 last updated on 03/Aug/21
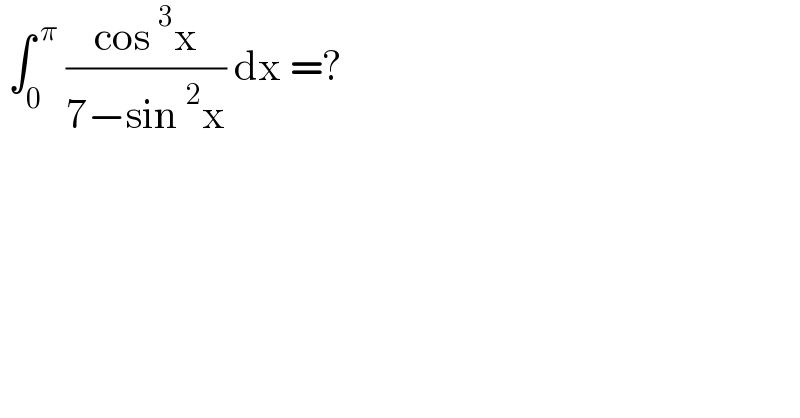
Answered by mathmax by abdo last updated on 03/Aug/21
![Ψ=∫_0 ^(π ) ((cos^3 x)/(7−sin^2 x)) dx ⇒Ψ=∫_0 ^π ((cos^3 x)/(7−(1−cos^2 x)))dx =∫_0 ^π ((cos^3 x)/(6+cos^2 x))dx =∫_0 ^π ((cosx(cos^2 x+6)−6cosx)/(cos^2 x +6))dx =∫_0 ^π cosx dx−6∫_0 ^π ((cosx)/(7−sin^2 x))dx we have ∫_0 ^π cosx dx=[sinx]_0 ^π =0 and ∫_0 ^π ((cosxdx)/(7−sin^2 x))=∫_0 ^(π/2) ((cosx)/(7−sin^2 x))dx +∫_(π/2) ^π ((cosx)/(7−sin^2 x))(→x=(π/2)+t) =_(sinx=y) ∫_0 ^1 (dy/(7−y^2 )) +∫_0 ^(π/2) ((−sint dt)/(7−cos^2 t))(→cost=y) =∫_0 ^1 (dy/(7−y^2 )) −∫_0 ^1 (dy/(7−y^2 )) =0 ⇒Ψ=0](Q149115.png)
Commented by bramlexs22 last updated on 03/Aug/21

Commented by ArielVyny last updated on 04/Aug/21

| ||
Question and Answers Forum | ||
Question Number 149113 by bramlexs22 last updated on 03/Aug/21 | ||
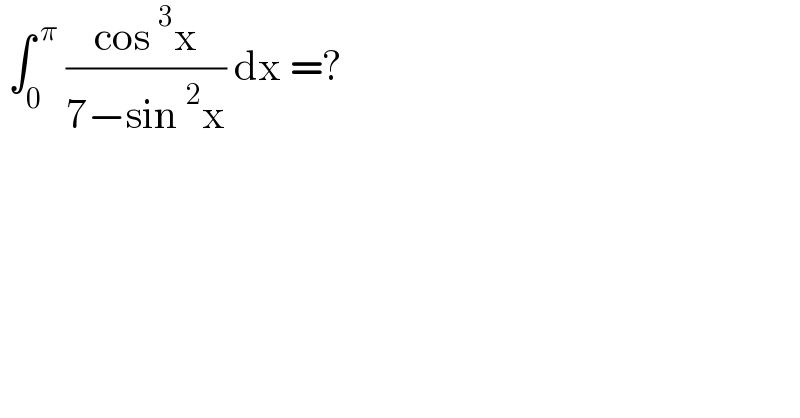 | ||
Answered by mathmax by abdo last updated on 03/Aug/21 | ||
![Ψ=∫_0 ^(π ) ((cos^3 x)/(7−sin^2 x)) dx ⇒Ψ=∫_0 ^π ((cos^3 x)/(7−(1−cos^2 x)))dx =∫_0 ^π ((cos^3 x)/(6+cos^2 x))dx =∫_0 ^π ((cosx(cos^2 x+6)−6cosx)/(cos^2 x +6))dx =∫_0 ^π cosx dx−6∫_0 ^π ((cosx)/(7−sin^2 x))dx we have ∫_0 ^π cosx dx=[sinx]_0 ^π =0 and ∫_0 ^π ((cosxdx)/(7−sin^2 x))=∫_0 ^(π/2) ((cosx)/(7−sin^2 x))dx +∫_(π/2) ^π ((cosx)/(7−sin^2 x))(→x=(π/2)+t) =_(sinx=y) ∫_0 ^1 (dy/(7−y^2 )) +∫_0 ^(π/2) ((−sint dt)/(7−cos^2 t))(→cost=y) =∫_0 ^1 (dy/(7−y^2 )) −∫_0 ^1 (dy/(7−y^2 )) =0 ⇒Ψ=0](Q149115.png) | ||
| ||
Commented by bramlexs22 last updated on 03/Aug/21 | ||
 | ||
Commented by ArielVyny last updated on 04/Aug/21 | ||
 | ||