Question and Answers Forum

All Questions      Topic List

Trigonometry Questions

Previous in All Question      Next in All Question      

Previous in Trigonometry      Next in Trigonometry      

Question Number 14977 by Tinkutara last updated on 06/Jun/17

Find the real roots of the equation cos^4 x + sin^7 x = 1 in the interval [−π, π].

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{real}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\mathrm{cos}^{\mathrm{4}} \:{x}\:+\:\mathrm{sin}^{\mathrm{7}} \:{x}\:=\:\mathrm{1}\:\mathrm{in}\:\mathrm{the}\:\mathrm{interval}\:\left[−\pi,\:\pi\right]. \\ $$

Commented by mrW1 last updated on 06/Jun/17

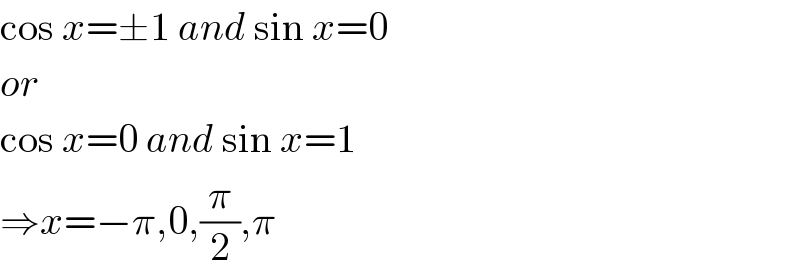
cos x=±1 and sin x=0 or cos x=0 and sin x=1 ⇒x=−π,0,(π/2),π

$$\mathrm{cos}\:{x}=\pm\mathrm{1}\:{and}\:\mathrm{sin}\:{x}=\mathrm{0} \\ $$$${or} \\ $$$$\mathrm{cos}\:{x}=\mathrm{0}\:{and}\:\mathrm{sin}\:{x}=\mathrm{1} \\ $$$$\Rightarrow{x}=−\pi,\mathrm{0},\frac{\pi}{\mathrm{2}},\pi \\ $$

Commented by Tinkutara last updated on 07/Jun/17

Thanks Sir!

$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$

Answered by myintkhaing last updated on 07/Jun/17

sin^7 x = 1 −cos^4 x sin^7 x = sin^2 x (2 − sin^2 x) sin^2 x(sin^5 x+sin^2 x −2) = 0 sin^2 x = 0 or sin^5 x+sin^2 x−2=0 sin x = 0 ⇒ x = 0, ±π sin^5 x+sin^2 x−2=0 , here −1≤sin x≤1 if sin x = −1, L.H.S ≠ R.H.S if sin x = 1, L.H.S = R.H.S x = (π/2) x = 0,±π,(π/2)

$${sin}^{\mathrm{7}} \:{x}\:=\:\mathrm{1}\:−{cos}^{\mathrm{4}} \:{x} \\ $$$${sin}^{\mathrm{7}} \:{x}\:=\:{sin}^{\mathrm{2}} \:{x}\:\left(\mathrm{2}\:−\:{sin}^{\mathrm{2}} {x}\right) \\ $$$${sin}^{\mathrm{2}} \:{x}\left({sin}^{\mathrm{5}} \:{x}+{sin}^{\mathrm{2}} \:{x}\:−\mathrm{2}\right)\:=\:\mathrm{0} \\ $$$${sin}^{\mathrm{2}} \:{x}\:=\:\mathrm{0}\:{or}\:{sin}^{\mathrm{5}} {x}+{sin}^{\mathrm{2}} {x}−\mathrm{2}=\mathrm{0} \\ $$$${sin}\:{x}\:=\:\mathrm{0}\:\Rightarrow\:{x}\:=\:\mathrm{0},\:\pm\pi \\ $$$${sin}^{\mathrm{5}} {x}+{sin}^{\mathrm{2}} {x}−\mathrm{2}=\mathrm{0}\:,\:{here}\:−\mathrm{1}\leqslant{sin}\:{x}\leqslant\mathrm{1} \\ $$$${if}\:{sin}\:{x}\:=\:−\mathrm{1},\:{L}.{H}.{S}\:\neq\:{R}.{H}.{S} \\ $$$${if}\:{sin}\:{x}\:=\:\mathrm{1},\:{L}.{H}.{S}\:=\:{R}.{H}.{S} \\ $$$${x}\:=\:\frac{\pi}{\mathrm{2}} \\ $$$${x}\:=\:\mathrm{0},\pm\pi,\frac{\pi}{\mathrm{2}} \\ $$$$ \\ $$

Commented by Tinkutara last updated on 07/Jun/17

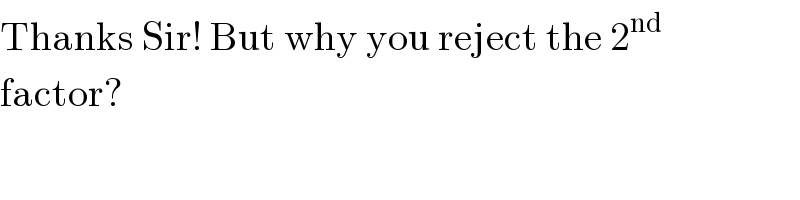
Thanks Sir! But why you reject the 2^(nd) factor?

$$\mathrm{Thanks}\:\mathrm{Sir}!\:\mathrm{But}\:\mathrm{why}\:\mathrm{you}\:\mathrm{reject}\:\mathrm{the}\:\mathrm{2}^{\mathrm{nd}} \\ $$$$\mathrm{factor}? \\ $$

Commented by myintkhaing last updated on 07/Jun/17

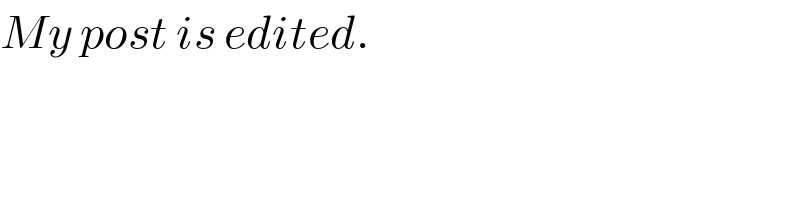
My post is edited.

$${My}\:{post}\:{is}\:{edited}. \\ $$

Commented by Tinkutara last updated on 07/Jun/17

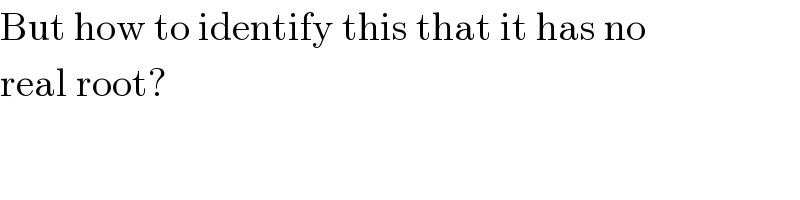
But how to identify this that it has no real root?

$$\mathrm{But}\:\mathrm{how}\:\mathrm{to}\:\mathrm{identify}\:\mathrm{this}\:\mathrm{that}\:\mathrm{it}\:\mathrm{has}\:\mathrm{no} \\ $$$$\mathrm{real}\:\mathrm{root}? \\ $$

Commented by Tinkutara last updated on 07/Jun/17

Thanks Sir!

$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$

Answered by mrW1 last updated on 07/Jun/17

This question can be generalised to cos^(2m) x + sin^(2n+1) x = 1 with m,n∈N^+ the answer is the same, independent from m and n. sin^(2n+1) x=1−cos^(2m) x≥0 and ≤1 ⇒0≤sin x≤1 for sin x=0 we get cos^(2m) x=1 or cos x=±1, this is possible. for sin x=1 we get cos^(2m) x=0 or cos x=0, this is possible. for 0<sin x<1 we get cos^(2m) x+sin^(2n+1) x=1 (cos^2 x)^m +sin^(2n+1) x=1 (1−sin^2 x)^m +sin^(2n+1) x=1 let t=sin x, 0<t<1 ⇒(1−t^2 )^m +t^(2n+1) =1 (1−t^2 )^m =1−t^(2n+1) =(1−t)(1+t+t^2 +...+t^(2n) ) ⇒(((1−t^2 )^m )/(1−t))=1+t+t^2 +...+t^(2n) >1+t ⇒(((1−t^2 )^m )/((1−t)(1+t)))>1 ⇒(1−t^2 )^(m−1) >1 but 0<1−t^2 <1 ⇒ (1−t^2 )^(m−1) <1 this is a contradiction, which results from the assumption that 0<sin x<1. i.e. the assumption is wrong. therefore the solution of the equation cos^(2m) x + sin^(2n+1) x = 1 with m,n∈N^+ is only sin x=0 and cos x=±1 ⇒x=kπ, k∈Z or sin x=1 and cos x=0 ⇒x=kπ+(−1)^k (π/2), k∈Z

$$\mathrm{This}\:\mathrm{question}\:\mathrm{can}\:\mathrm{be}\:\mathrm{generalised}\:\mathrm{to} \\ $$$$\mathrm{cos}^{\mathrm{2m}} \:\mathrm{x}\:+\:\mathrm{sin}^{\mathrm{2n}+\mathrm{1}} \:\mathrm{x}\:=\:\mathrm{1}\:\mathrm{with}\:\mathrm{m},\mathrm{n}\in\mathbb{N}^{+} \\ $$$$\mathrm{the}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{the}\:\mathrm{same},\:\mathrm{independent} \\ $$$$\mathrm{from}\:\mathrm{m}\:\mathrm{and}\:\mathrm{n}. \\ $$$$\mathrm{sin}^{\mathrm{2n}+\mathrm{1}} \:\mathrm{x}=\mathrm{1}−\mathrm{cos}^{\mathrm{2m}} \:\mathrm{x}\geqslant\mathrm{0}\:\mathrm{and}\:\leqslant\mathrm{1} \\ $$$$\Rightarrow\mathrm{0}\leqslant\mathrm{sin}\:\mathrm{x}\leqslant\mathrm{1} \\ $$$$ \\ $$$$\mathrm{for}\:\mathrm{sin}\:\mathrm{x}=\mathrm{0}\:\mathrm{we}\:\mathrm{get}\:\mathrm{cos}^{\mathrm{2m}} \:\mathrm{x}=\mathrm{1}\:\mathrm{or} \\ $$$$\mathrm{cos}\:\mathrm{x}=\pm\mathrm{1},\:\mathrm{this}\:\mathrm{is}\:\mathrm{possible}. \\ $$$$ \\ $$$$\mathrm{for}\:\mathrm{sin}\:\mathrm{x}=\mathrm{1}\:\mathrm{we}\:\mathrm{get}\:\mathrm{cos}^{\mathrm{2m}} \:\mathrm{x}=\mathrm{0}\:\mathrm{or} \\ $$$$\mathrm{cos}\:\mathrm{x}=\mathrm{0},\:\mathrm{this}\:\mathrm{is}\:\mathrm{possible}. \\ $$$$ \\ $$$$\mathrm{for}\:\mathrm{0}<\mathrm{sin}\:\mathrm{x}<\mathrm{1}\:\mathrm{we}\:\mathrm{get} \\ $$$$\mathrm{cos}^{\mathrm{2m}} \:\mathrm{x}+\mathrm{sin}^{\mathrm{2n}+\mathrm{1}} \:\mathrm{x}=\mathrm{1} \\ $$$$\left(\mathrm{cos}^{\mathrm{2}} \:\mathrm{x}\right)^{\mathrm{m}} +\mathrm{sin}^{\mathrm{2n}+\mathrm{1}} \:\mathrm{x}=\mathrm{1} \\ $$$$\left(\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:\mathrm{x}\right)^{\mathrm{m}} +\mathrm{sin}^{\mathrm{2n}+\mathrm{1}} \:\mathrm{x}=\mathrm{1} \\ $$$$\mathrm{let}\:\mathrm{t}=\mathrm{sin}\:\mathrm{x},\:\mathrm{0}<\mathrm{t}<\mathrm{1} \\ $$$$\Rightarrow\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{m}} +\mathrm{t}^{\mathrm{2n}+\mathrm{1}} =\mathrm{1} \\ $$$$\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{m}} =\mathrm{1}−\mathrm{t}^{\mathrm{2n}+\mathrm{1}} =\left(\mathrm{1}−\mathrm{t}\right)\left(\mathrm{1}+\mathrm{t}+\mathrm{t}^{\mathrm{2}} +...+\mathrm{t}^{\mathrm{2n}} \right) \\ $$$$\Rightarrow\frac{\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{m}} }{\mathrm{1}−\mathrm{t}}=\mathrm{1}+\mathrm{t}+\mathrm{t}^{\mathrm{2}} +...+\mathrm{t}^{\mathrm{2n}} >\mathrm{1}+\mathrm{t} \\ $$$$\Rightarrow\frac{\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{m}} }{\left(\mathrm{1}−\mathrm{t}\right)\left(\mathrm{1}+\mathrm{t}\right)}>\mathrm{1} \\ $$$$\Rightarrow\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{m}−\mathrm{1}} >\mathrm{1} \\ $$$$\mathrm{but}\:\mathrm{0}<\mathrm{1}−\mathrm{t}^{\mathrm{2}} <\mathrm{1}\:\Rightarrow\:\left(\mathrm{1}−\mathrm{t}^{\mathrm{2}} \right)^{\mathrm{m}−\mathrm{1}} <\mathrm{1} \\ $$$$\mathrm{this}\:\mathrm{is}\:\mathrm{a}\:\mathrm{contradiction},\:\mathrm{which}\:\mathrm{results} \\ $$$$\mathrm{from}\:\mathrm{the}\:\mathrm{assumption}\:\mathrm{that}\:\mathrm{0}<\mathrm{sin}\:\mathrm{x}<\mathrm{1}. \\ $$$$\mathrm{i}.\mathrm{e}.\:\mathrm{the}\:\mathrm{assumption}\:\mathrm{is}\:\mathrm{wrong}. \\ $$$$ \\ $$$$\mathrm{therefore}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\mathrm{cos}^{\mathrm{2m}} \:\mathrm{x}\:+\:\mathrm{sin}^{\mathrm{2n}+\mathrm{1}} \:\mathrm{x}\:=\:\mathrm{1}\:\mathrm{with}\:\mathrm{m},\mathrm{n}\in\mathbb{N}^{+} \\ $$$$\mathrm{is}\:\mathrm{only} \\ $$$$\mathrm{sin}\:\mathrm{x}=\mathrm{0}\:\mathrm{and}\:\mathrm{cos}\:\mathrm{x}=\pm\mathrm{1}\: \\ $$$$\Rightarrow\mathrm{x}=\mathrm{k}\pi,\:\mathrm{k}\in\mathbb{Z} \\ $$$$\mathrm{or} \\ $$$$\mathrm{sin}\:\mathrm{x}=\mathrm{1}\:\mathrm{and}\:\mathrm{cos}\:\mathrm{x}=\mathrm{0}\: \\ $$$$\Rightarrow\mathrm{x}=\mathrm{k}\pi+\left(−\mathrm{1}\right)^{\mathrm{k}} \frac{\pi}{\mathrm{2}},\:\mathrm{k}\in\mathbb{Z} \\ $$

Commented by Tinkutara last updated on 07/Jun/17

Thanks Sir!

$$\mathrm{Thanks}\:\mathrm{Sir}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com