Question and Answers Forum

All Questions      Topic List

Coordinate Geometry Questions

Previous in All Question      Next in All Question      

Previous in Coordinate Geometry      Next in Coordinate Geometry      

Question Number 150596 by mr W last updated on 14/Aug/21

Commented by mr W last updated on 14/Aug/21

a more challenging case: 3D case three vertex of a tetrahedron lie on the coordinate axes. the fourth one lies on the sphere with radius R and center at G(p,q,r). find the minimum and maximum volume of the tetrahedron.

$${a}\:{more}\:{challenging}\:{case}:\:\mathrm{3}{D}\:{case} \\ $$$${three}\:{vertex}\:{of}\:{a}\:{tetrahedron}\:{lie}\:{on} \\ $$$${the}\:{coordinate}\:{axes}.\:{the}\:{fourth}\:{one} \\ $$$${lies}\:{on}\:{the}\:{sphere}\:{with}\:{radius}\:{R}\:{and} \\ $$$${center}\:{at}\:{G}\left({p},{q},{r}\right). \\ $$$${find}\:{the}\:{minimum}\:{and}\:{maximum} \\ $$$${volume}\:{of}\:{the}\:{tetrahedron}. \\ $$

Answered by ajfour last updated on 14/Aug/21

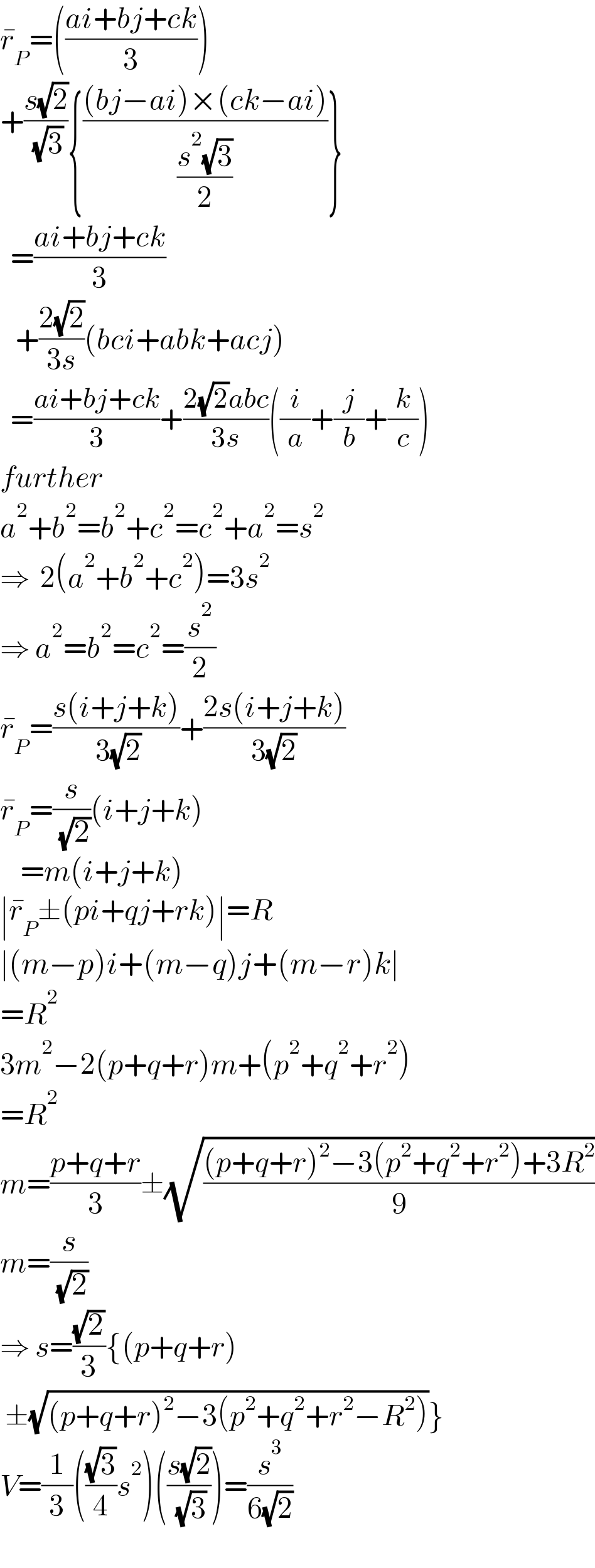
r_P ^� =(((ai+bj+ck)/3)) +((s(√2))/( (√3))){(((bj−ai)×(ck−ai))/((s^2 (√3))/2))} =((ai+bj+ck)/3) +((2(√2))/(3s))(bci+abk+acj) =((ai+bj+ck)/3)+((2(√2)abc)/(3s))((i/a)+(j/b)+(k/c)) further a^2 +b^2 =b^2 +c^2 =c^2 +a^2 =s^2 ⇒ 2(a^2 +b^2 +c^2 )=3s^2 ⇒ a^2 =b^2 =c^2 =(s^2 /2) r_P ^� =((s(i+j+k))/( 3(√2)))+((2s(i+j+k))/(3(√2))) r_P ^� =(s/( (√2)))(i+j+k) =m(i+j+k) ∣r_P ^� ±(pi+qj+rk)∣=R ∣(m−p)i+(m−q)j+(m−r)k∣ =R^2 3m^2 −2(p+q+r)m+(p^2 +q^2 +r^2 ) =R^2 m=((p+q+r)/3)±(√(((p+q+r)^2 −3(p^2 +q^2 +r^2 )+3R^2 )/9)) m=(s/( (√2))) ⇒ s=((√2)/3){(p+q+r) ±(√((p+q+r)^2 −3(p^2 +q^2 +r^2 −R^2 )))} V=(1/3)(((√3)/4)s^2 )(((s(√2))/( (√3))))=(s^3 /(6(√2)))

$$\bar {{r}}_{{P}} =\left(\frac{{ai}+{bj}+{ck}}{\mathrm{3}}\right) \\ $$$$+\frac{{s}\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{3}}}\left\{\frac{\left({bj}−{ai}\right)×\left({ck}−{ai}\right)}{\frac{{s}^{\mathrm{2}} \sqrt{\mathrm{3}}}{\mathrm{2}}}\right\} \\ $$$$\:\:=\frac{{ai}+{bj}+{ck}}{\mathrm{3}} \\ $$$$\:\:\:+\frac{\mathrm{2}\sqrt{\mathrm{2}}}{\mathrm{3}{s}}\left({bci}+{abk}+{acj}\right) \\ $$$$\:\:=\frac{{ai}+{bj}+{ck}}{\mathrm{3}}+\frac{\mathrm{2}\sqrt{\mathrm{2}}{abc}}{\mathrm{3}{s}}\left(\frac{{i}}{{a}}+\frac{{j}}{{b}}+\frac{{k}}{{c}}\right) \\ $$$${further} \\ $$$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} ={b}^{\mathrm{2}} +{c}^{\mathrm{2}} ={c}^{\mathrm{2}} +{a}^{\mathrm{2}} ={s}^{\mathrm{2}} \\ $$$$\Rightarrow\:\:\mathrm{2}\left({a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} \right)=\mathrm{3}{s}^{\mathrm{2}} \\ $$$$\Rightarrow\:{a}^{\mathrm{2}} ={b}^{\mathrm{2}} ={c}^{\mathrm{2}} =\frac{{s}^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\bar {{r}}_{{P}} =\frac{{s}\left({i}+{j}+{k}\right)}{\:\mathrm{3}\sqrt{\mathrm{2}}}+\frac{\mathrm{2}{s}\left({i}+{j}+{k}\right)}{\mathrm{3}\sqrt{\mathrm{2}}} \\ $$$$\bar {{r}}_{{P}} =\frac{{s}}{\:\sqrt{\mathrm{2}}}\left({i}+{j}+{k}\right) \\ $$$$\:\:\:\:={m}\left({i}+{j}+{k}\right) \\ $$$$\mid\bar {{r}}_{{P}} \pm\left({pi}+{qj}+{rk}\right)\mid={R} \\ $$$$\mid\left({m}−{p}\right){i}+\left({m}−{q}\right){j}+\left({m}−{r}\right){k}\mid \\ $$$$={R}^{\mathrm{2}} \\ $$$$\mathrm{3}{m}^{\mathrm{2}} −\mathrm{2}\left({p}+{q}+{r}\right){m}+\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} \right) \\ $$$$={R}^{\mathrm{2}} \\ $$$${m}=\frac{{p}+{q}+{r}}{\mathrm{3}}\pm\sqrt{\frac{\left({p}+{q}+{r}\right)^{\mathrm{2}} −\mathrm{3}\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} \right)+\mathrm{3}{R}^{\mathrm{2}} }{\mathrm{9}}} \\ $$$${m}=\frac{{s}}{\:\sqrt{\mathrm{2}}} \\ $$$$\Rightarrow\:{s}=\frac{\sqrt{\mathrm{2}}}{\mathrm{3}}\left\{\left({p}+{q}+{r}\right)\right. \\ $$$$\left.\:\pm\sqrt{\left({p}+{q}+{r}\right)^{\mathrm{2}} −\mathrm{3}\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} −{R}^{\mathrm{2}} \right)}\right\} \\ $$$${V}=\frac{\mathrm{1}}{\mathrm{3}}\left(\frac{\sqrt{\mathrm{3}}}{\mathrm{4}}{s}^{\mathrm{2}} \right)\left(\frac{{s}\sqrt{\mathrm{2}}}{\:\sqrt{\mathrm{3}}}\right)=\frac{{s}^{\mathrm{3}} }{\mathrm{6}\sqrt{\mathrm{2}}} \\ $$$$ \\ $$

Answered by mr W last updated on 14/Aug/21

say edge length of tetrahedron is s. ΔABC is an equilateral triangle with side length s. A(a,0,0) B(0,b,0) C(0,0,c) since AB=BC=CA=s, ⇒a=b=c=(s/( (√2))) eqn. of plane ABC is x+y+z=(s/( (√2))) the fourth vertex lies on the line x=y=z=k, say since it lies also on the sphere (x−p)^2 +(y−q)^2 +(z−r)^2 =R^2 (k−p)^2 +(k−q)^2 +(k−r)^2 =R^2 3k^2 −2(p+q+r)k+p^2 +q^2 +r^2 −R^2 =0 ⇒k=(1/3){p+q+r±(√((p+q+r)^2 −3(p^2 +q^2 +r^2 −R^2 )))} solution exists only if (p+q+r)^2 −3(p^2 +q^2 +r^2 )≥3R^2 the distance from intersection point P(k,k,k) to the plane ABC is the heigth of the tetrahedron, which is (((√6)s)/3). ((3k−(s/( (√2))))/( (√3)))=±(((√6)s)/3) 3k=(((√2)(1±2)s)/2) ⇒s=((3(√2)k)/(1±2))>0 ⇒s=((√2)/(1±2)){p+q+r±(√((p+q+r)^2 −3(p^2 +q^2 +r^2 −R^2 )))} the volume of tetrahedron is V=(s^3 /(6(√2))) in general there exist 2 tetrahedrons.

$${say}\:{edge}\:{length}\:{of}\:{tetrahedron}\:{is}\:{s}. \\ $$$$\Delta{ABC}\:{is}\:{an}\:{equilateral}\:{triangle}\:{with} \\ $$$${side}\:{length}\:{s}. \\ $$$${A}\left({a},\mathrm{0},\mathrm{0}\right) \\ $$$${B}\left(\mathrm{0},{b},\mathrm{0}\right) \\ $$$${C}\left(\mathrm{0},\mathrm{0},{c}\right) \\ $$$${since}\:{AB}={BC}={CA}={s}, \\ $$$$\Rightarrow{a}={b}={c}=\frac{{s}}{\:\sqrt{\mathrm{2}}} \\ $$$${eqn}.\:{of}\:{plane}\:{ABC}\:{is} \\ $$$${x}+{y}+{z}=\frac{{s}}{\:\sqrt{\mathrm{2}}} \\ $$$${the}\:{fourth}\:{vertex}\:{lies}\:{on}\:{the}\:{line} \\ $$$${x}={y}={z}={k},\:{say} \\ $$$${since}\:{it}\:{lies}\:{also}\:{on}\:{the}\:{sphere} \\ $$$$\left({x}−{p}\right)^{\mathrm{2}} +\left({y}−{q}\right)^{\mathrm{2}} +\left({z}−{r}\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$$\left({k}−{p}\right)^{\mathrm{2}} +\left({k}−{q}\right)^{\mathrm{2}} +\left({k}−{r}\right)^{\mathrm{2}} ={R}^{\mathrm{2}} \\ $$$$\mathrm{3}{k}^{\mathrm{2}} −\mathrm{2}\left({p}+{q}+{r}\right){k}+{p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} −{R}^{\mathrm{2}} =\mathrm{0} \\ $$$$\Rightarrow{k}=\frac{\mathrm{1}}{\mathrm{3}}\left\{{p}+{q}+{r}\pm\sqrt{\left({p}+{q}+{r}\right)^{\mathrm{2}} −\mathrm{3}\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} −{R}^{\mathrm{2}} \right)}\right\} \\ $$$${solution}\:{exists}\:{only}\:{if}\: \\ $$$$\left({p}+{q}+{r}\right)^{\mathrm{2}} −\mathrm{3}\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} \right)\geqslant\mathrm{3}{R}^{\mathrm{2}} \\ $$$$ \\ $$$${the}\:{distance}\:{from}\:{intersection}\:{point} \\ $$$${P}\left({k},{k},{k}\right)\:{to}\:{the}\:{plane}\:{ABC}\:{is}\:{the} \\ $$$${heigth}\:{of}\:{the}\:{tetrahedron},\:{which}\:{is} \\ $$$$\frac{\sqrt{\mathrm{6}}{s}}{\mathrm{3}}. \\ $$$$\frac{\mathrm{3}{k}−\frac{{s}}{\:\sqrt{\mathrm{2}}}}{\:\sqrt{\mathrm{3}}}=\pm\frac{\sqrt{\mathrm{6}}{s}}{\mathrm{3}} \\ $$$$\mathrm{3}{k}=\frac{\sqrt{\mathrm{2}}\left(\mathrm{1}\pm\mathrm{2}\right){s}}{\mathrm{2}} \\ $$$$\Rightarrow{s}=\frac{\mathrm{3}\sqrt{\mathrm{2}}{k}}{\mathrm{1}\pm\mathrm{2}}>\mathrm{0} \\ $$$$\Rightarrow{s}=\frac{\sqrt{\mathrm{2}}}{\mathrm{1}\pm\mathrm{2}}\left\{{p}+{q}+{r}\pm\sqrt{\left({p}+{q}+{r}\right)^{\mathrm{2}} −\mathrm{3}\left({p}^{\mathrm{2}} +{q}^{\mathrm{2}} +{r}^{\mathrm{2}} −{R}^{\mathrm{2}} \right)}\right\} \\ $$$${the}\:{volume}\:{of}\:{tetrahedron}\:{is} \\ $$$${V}=\frac{{s}^{\mathrm{3}} }{\mathrm{6}\sqrt{\mathrm{2}}} \\ $$$${in}\:{general}\:{there}\:{exist}\:\mathrm{2}\:{tetrahedrons}. \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com