
Question and Answers Forum
Question Number 52550 by Tawa1 last updated on 09/Jan/19
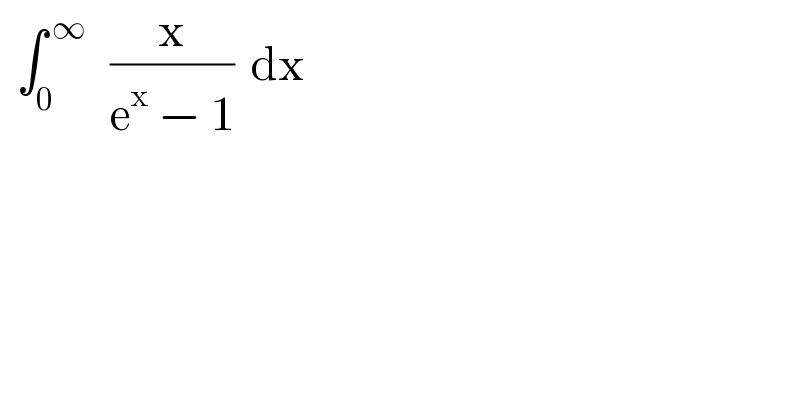
Commented by maxmathsup by imad last updated on 11/Jan/19
![let I =∫_0 ^∞ (x/(e^x −1))dx ⇒ I =∫_0 ^∞ ((xe^(−x) )/(1−e^(−x) ))dx =∫_0 ^∞ xe^(−x) (Σ_(n=0) ^∞ e^(−nx) )dx =Σ_(n=0) ^∞ (∫_0 ^∞ x e^(−(n+1)x) dx)=Σ_(n=0) ^∞ A_n A_n =∫_0 ^∞ x e^(−(n+1x) dx =_((n+1)x=t ) ∫_0 ^∞ (t/(n+1)) e^(−t) (dt/(n+1)) =(1/((n+1)^2 ))∫_0 ^∞ t e^(−t) dt but by parts ∫_0 ^∞ t e^(−t) dt =[−t e^(−t) ]_0 ^(+∞) +∫ e^(−t) dt =[−e^(−t) ]_0 ^(+∞) =1 ⇒A_n =(1/((n+1)^2 )) ⇒ I =Σ_(n=0) ^∞ (1/((n+1)^2 )) =Σ_(n=1) ^∞ (1/n^2 ) =(π^2 /6) .](Q52668.png)
Answered by Smail last updated on 09/Jan/19
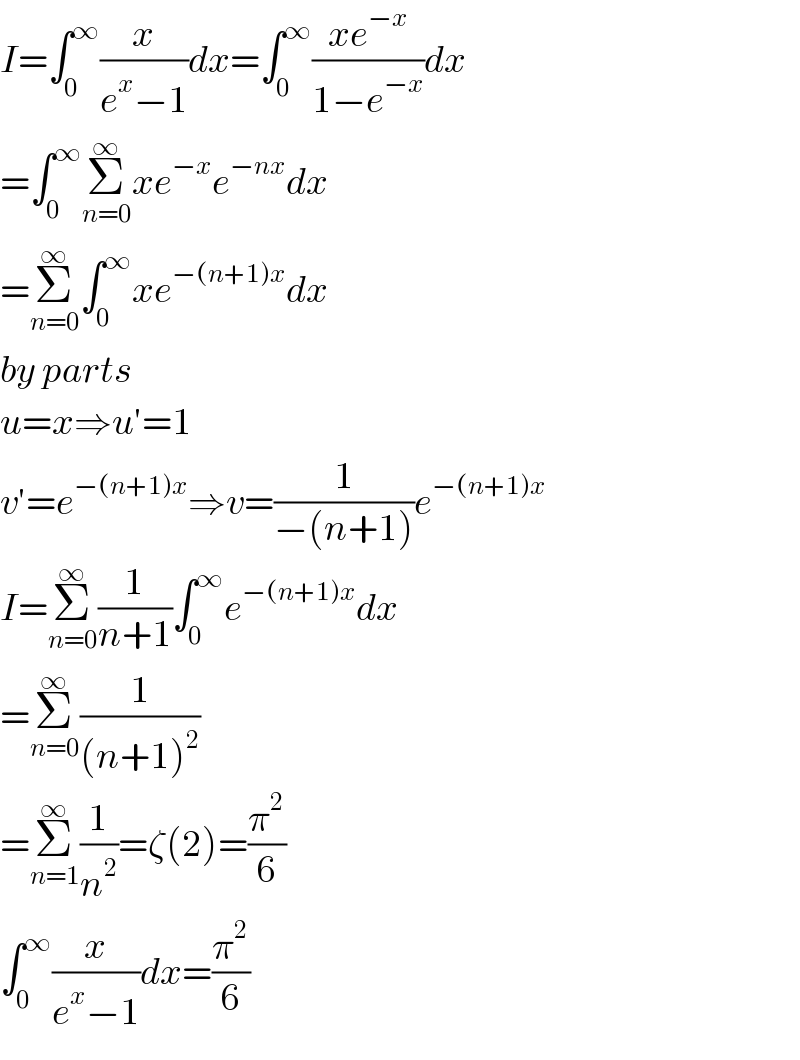
Commented by Tawa1 last updated on 09/Jan/19

| ||
Question and Answers Forum | ||
Question Number 52550 by Tawa1 last updated on 09/Jan/19 | ||
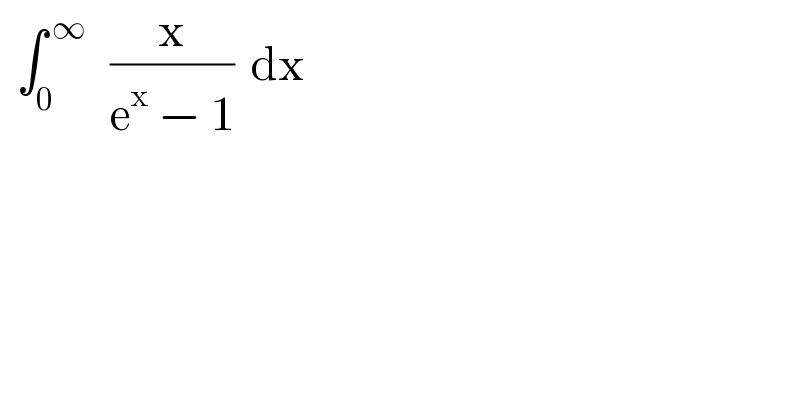 | ||
Commented by maxmathsup by imad last updated on 11/Jan/19 | ||
![let I =∫_0 ^∞ (x/(e^x −1))dx ⇒ I =∫_0 ^∞ ((xe^(−x) )/(1−e^(−x) ))dx =∫_0 ^∞ xe^(−x) (Σ_(n=0) ^∞ e^(−nx) )dx =Σ_(n=0) ^∞ (∫_0 ^∞ x e^(−(n+1)x) dx)=Σ_(n=0) ^∞ A_n A_n =∫_0 ^∞ x e^(−(n+1x) dx =_((n+1)x=t ) ∫_0 ^∞ (t/(n+1)) e^(−t) (dt/(n+1)) =(1/((n+1)^2 ))∫_0 ^∞ t e^(−t) dt but by parts ∫_0 ^∞ t e^(−t) dt =[−t e^(−t) ]_0 ^(+∞) +∫ e^(−t) dt =[−e^(−t) ]_0 ^(+∞) =1 ⇒A_n =(1/((n+1)^2 )) ⇒ I =Σ_(n=0) ^∞ (1/((n+1)^2 )) =Σ_(n=1) ^∞ (1/n^2 ) =(π^2 /6) .](Q52668.png) | ||
Answered by Smail last updated on 09/Jan/19 | ||
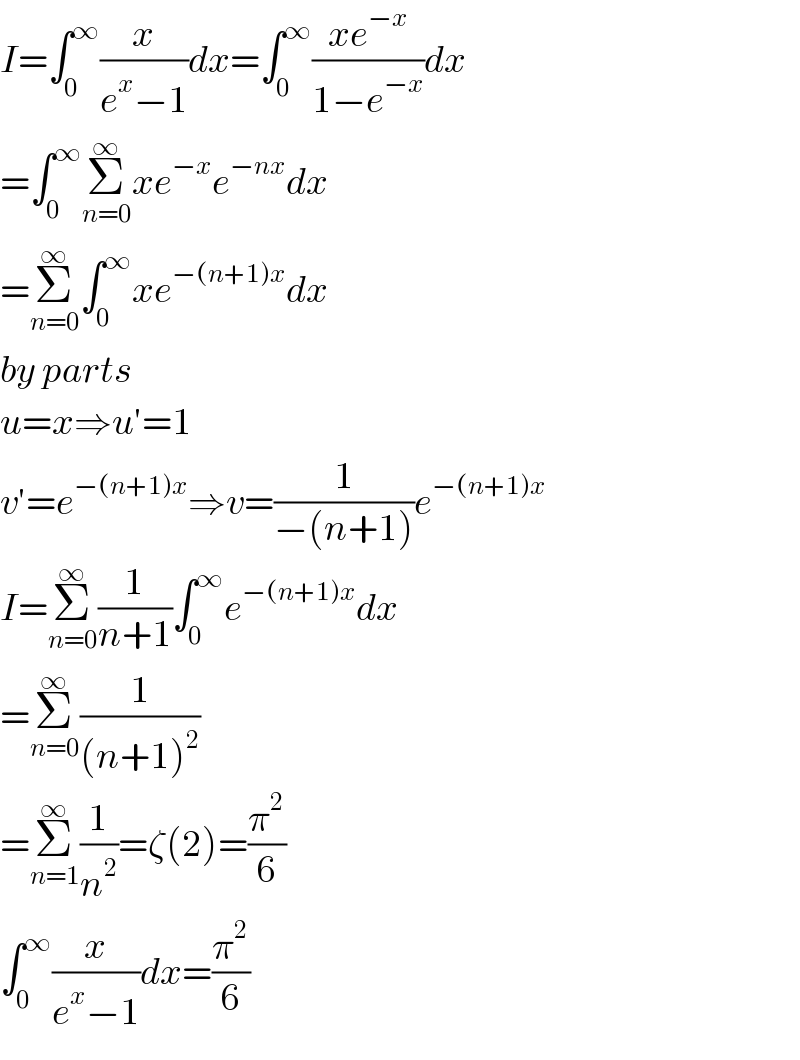 | ||
| ||
Commented by Tawa1 last updated on 09/Jan/19 | ||
 | ||