
Question and Answers Forum
Question Number 151230 by mnjuly1970 last updated on 19/Aug/21
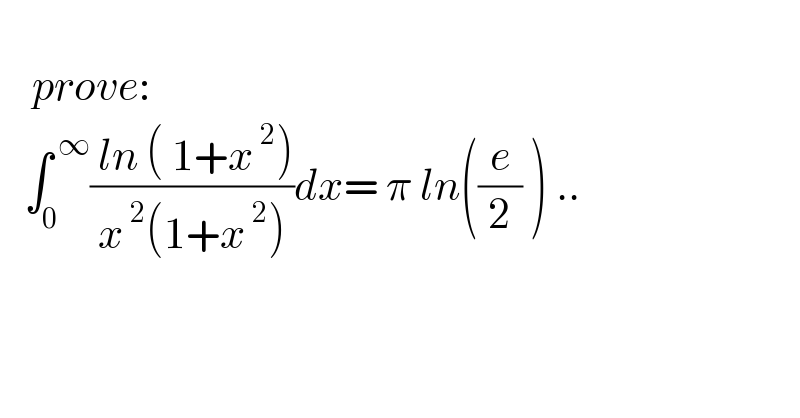
Commented by Lordose last updated on 19/Aug/21

Commented by Lordose last updated on 19/Aug/21

Answered by Lordose last updated on 19/Aug/21

Commented by mnjuly1970 last updated on 19/Aug/21
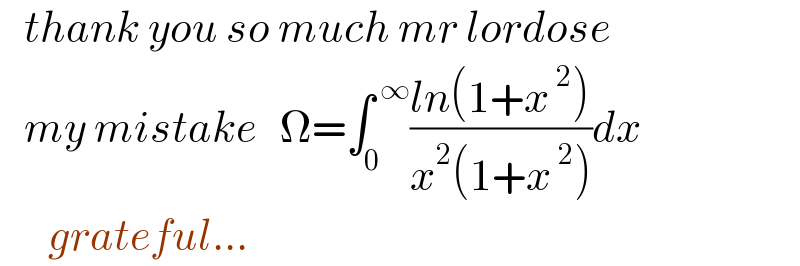
Answered by qaz last updated on 19/Aug/21

Answered by Lordose last updated on 19/Aug/21
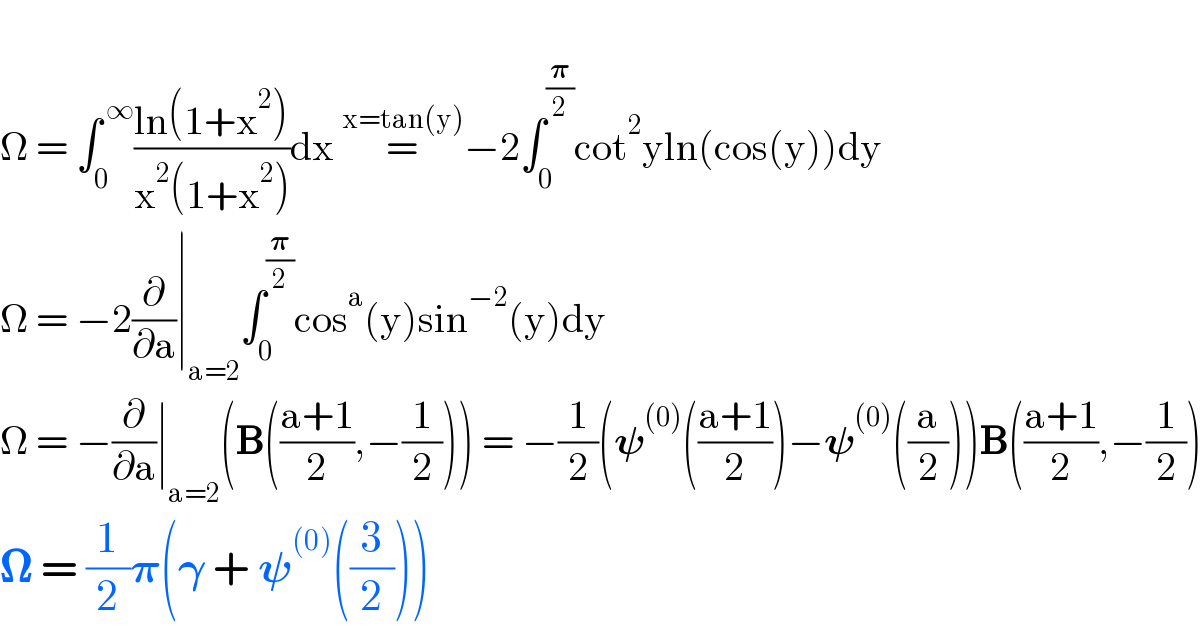
Answered by qaz last updated on 19/Aug/21
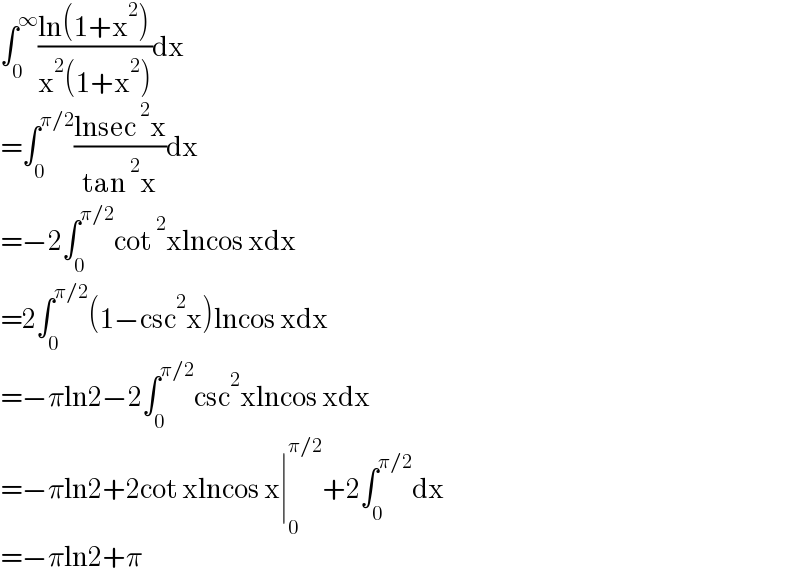
Commented by mnjuly1970 last updated on 19/Aug/21

Commented by peter frank last updated on 19/Aug/21

