Question and Answers Forum

All Questions      Topic List

Number Theory Questions

Previous in All Question      Next in All Question      

Previous in Number Theory      Next in Number Theory      

Question Number 15292 by RasheedSoomro last updated on 09/Jun/17

Light version of Q#13724 Expansion of 100! has 24, 0′s at the end. Find the first non-zero digit from right. 100!=.....d000...00 What is the value of d?

$$\mathrm{Light}\:\mathrm{version}\:\mathrm{of}\:\mathcal{Q}#\mathrm{13724} \\ $$$$\mathcal{E}\mathrm{xpansion}\:\mathrm{of}\:\mathrm{100}!\:\mathrm{has}\:\mathrm{24},\:\mathrm{0}'\mathrm{s}\:\:\mathrm{at}\:\mathrm{the}\:\mathrm{end}. \\ $$$$\mathrm{Find}\:\mathrm{the}\:\mathrm{first}\:\mathrm{non}-\mathrm{zero}\:\mathrm{digit}\:\mathrm{from}\:\mathrm{right}. \\ $$$$\mathrm{100}!=.....\mathrm{d000}...\mathrm{00} \\ $$$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{d}? \\ $$

Answered by RasheedSoomro last updated on 11/Jun/17

100! has 24 0′s from right. p=((100!)/(10^(24) )) has no zero at the end. ∴ First non-zero digit of 100! =unit digit of p ∴ p≡d(mod 10) Simplifying p=((100!)/(10^(24) ))=((100!)/(2^(24) ×5^(24) )) 10^(24) =2^(24) ×5^(24) =2^(6•) (5.10.15...100) All the 24 5′s have come in 5.10...100 ^• Also some 2′s have come: One 2 in each multiple of 10 10 2′s One 2 extra in each multiple of 20 5 2′s One 2 extra in each multiple of 40 2 2′s One 2 extra in each multiple of 80 1 2′s _(−) Total(included in 5.10...100) 18 2′s Remaining 24−18=6 So now p=((100!)/(10^(24) ))=((100!)/(2^(24) ×5^(24) ))=((100!)/(2^6 (5.10....100))) p=(1/(2.2^5 )){(1.2.3.4)(6.7.8.9)...(96.97.98.99} p={(((1.2.3.4)/2))(6.7.8.9).(11.12.13.14)(((16.17.18.19)/2))} ×{(21.22.23.24)(26.27.28.29).(31.32.33.34)(((36.37.38.39)/2))} ...×{(81.82.83.84)(86.87.88.89).(91.92.93.94)(((96.97.98.99)/2))} Account of 2^6 : One 2 comes in read bracket 5 2′s come in five blue brackets (one blue bracket is per each 20) Now we can verify/prove the following congruences: ((1.2.3.4)/2)≡2(mod 10) {: ((6.7.8.9)),(⋮),((91.92.93.94)) }≡4(mod 10) [14 congruencs] (5k+1)(5k+2)(5k+3)(5k+4)≡4(mod 10) { ((^• See Answer of mrW1 ⌉)),((to Q#14614 or an)),(( alternative proof in)),((the comment below.)) :} {: (((16.17.18.19)/2)),(⋮),(((96.97.98.99)/2)) }≡2 [5 congruences] {(5k+1)(5k+2)(5k+3)(5k+4)}/2≡4(mod 10) { ((^∗ Fo proof see the)),((comment below.)) :} Multiplying all the above congruences p≡2.4^(14) .2^5 (mod 10) 4^(14) ≡6(mod 10) [4^(2k) ≡6(mod 10)] 2^5 ≡32≡2(mod 10) p≡2.6.2(mod 10) p≡4(mod 10) Hence the require non-zero digit is 4 It′s only accedent that the answer is correct! Actually there′s a fault in the logic. I′ll try to correct my logic. Please stay with me!

$$\mathrm{100}!\:\mathrm{has}\:\mathrm{24}\:\:\:\mathrm{0}'\mathrm{s}\:\mathrm{from}\:\mathrm{right}. \\ $$$$\mathrm{p}=\frac{\mathrm{100}!}{\mathrm{10}^{\mathrm{24}} }\:\mathrm{has}\:\mathrm{no}\:\mathrm{zero}\:\mathrm{at}\:\mathrm{the}\:\mathrm{end}. \\ $$$$\therefore\:\:\mathrm{First}\:\mathrm{non}-\mathrm{zero}\:\mathrm{digit}\:\mathrm{of}\:\mathrm{100}! \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{unit}\:\mathrm{digit}\:\mathrm{of}\:\:\mathrm{p} \\ $$$$\therefore\:\:\mathrm{p}\equiv\mathrm{d}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathcal{S}{implifying}\:\:\:\mathrm{p}=\frac{\mathrm{100}!}{\mathrm{10}^{\mathrm{24}} }=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{24}} ×\mathrm{5}^{\mathrm{24}} } \\ $$$$\:\:\:\:\:\:\:\:\mathrm{10}^{\mathrm{24}} =\mathrm{2}^{\mathrm{24}} ×\mathrm{5}^{\mathrm{24}} =\mathrm{2}^{\mathrm{6}\bullet} \left(\mathrm{5}.\mathrm{10}.\mathrm{15}...\mathrm{100}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{All}\:\mathrm{the}\:\mathrm{24}\:\:\:\mathrm{5}'\mathrm{s}\:\mathrm{have}\:\mathrm{come}\:\mathrm{in}\:\mathrm{5}.\mathrm{10}...\mathrm{100} \\ $$$$\:\:\:\:\:\:\:\:\:\:^{\bullet} \mathrm{Also}\:\mathrm{some}\:\mathrm{2}'\mathrm{s}\:\mathrm{have}\:\mathrm{come}: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{One}\:\mathrm{2}\:\mathrm{in}\:\mathrm{each}\:\mathrm{multiple}\:\mathrm{of}\:\mathrm{10}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{10}\:\:\mathrm{2}'\mathrm{s} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{One}\:\mathrm{2}\:\mathrm{extra}\:\:\mathrm{in}\:\mathrm{each}\:\mathrm{multiple}\:\mathrm{of}\:\mathrm{20}\:\:\:\mathrm{5}\:\:\:\mathrm{2}'\mathrm{s} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{One}\:\mathrm{2}\:\mathrm{extra}\:\:\mathrm{in}\:\mathrm{each}\:\mathrm{multiple}\:\mathrm{of}\:\mathrm{40}\:\:\:\mathrm{2}\:\:\:\mathrm{2}'\mathrm{s} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{One}\:\mathrm{2}\:\mathrm{extra}\:\:\mathrm{in}\:\mathrm{each}\:\mathrm{mult}\underset{−} {\mathrm{iple}\:\mathrm{of}\:\mathrm{80}\:\:\:\mathrm{1}\:\:\:\mathrm{2}'\mathrm{s}\:\:\:\:\:\:\:\:\:} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{Total}\left(\mathrm{included}\:\mathrm{in}\:\mathrm{5}.\mathrm{10}...\mathrm{100}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{18}\:\:\mathrm{2}'\mathrm{s}\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{Remaining}\:\:\mathrm{24}−\mathrm{18}=\mathrm{6} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{So}\:\mathrm{now}\:\mathrm{p}=\frac{\mathrm{100}!}{\mathrm{10}^{\mathrm{24}} }=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{24}} ×\mathrm{5}^{\mathrm{24}} }=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{6}} \left(\mathrm{5}.\mathrm{10}....\mathrm{100}\right)} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{p}=\frac{\mathrm{1}}{\mathrm{2}.\mathrm{2}^{\mathrm{5}} }\left\{\left(\mathrm{1}.\mathrm{2}.\mathrm{3}.\mathrm{4}\right)\left(\mathrm{6}.\mathrm{7}.\mathrm{8}.\mathrm{9}\right)...\left(\mathrm{96}.\mathrm{97}.\mathrm{98}.\mathrm{99}\right\}\right. \\ $$$$\:\:\:\:\:\:\:\:\mathrm{p}=\left\{\left(\frac{\mathrm{1}.\mathrm{2}.\mathrm{3}.\mathrm{4}}{\mathrm{2}}\right)\left(\mathrm{6}.\mathrm{7}.\mathrm{8}.\mathrm{9}\right).\left(\mathrm{11}.\mathrm{12}.\mathrm{13}.\mathrm{14}\right)\left(\frac{\mathrm{16}.\mathrm{17}.\mathrm{18}.\mathrm{19}}{\mathrm{2}}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:×\left\{\left(\mathrm{21}.\mathrm{22}.\mathrm{23}.\mathrm{24}\right)\left(\mathrm{26}.\mathrm{27}.\mathrm{28}.\mathrm{29}\right).\left(\mathrm{31}.\mathrm{32}.\mathrm{33}.\mathrm{34}\right)\left(\frac{\mathrm{36}.\mathrm{37}.\mathrm{38}.\mathrm{39}}{\mathrm{2}}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:...×\left\{\left(\mathrm{81}.\mathrm{82}.\mathrm{83}.\mathrm{84}\right)\left(\mathrm{86}.\mathrm{87}.\mathrm{88}.\mathrm{89}\right).\left(\mathrm{91}.\mathrm{92}.\mathrm{93}.\mathrm{94}\right)\left(\frac{\mathrm{96}.\mathrm{97}.\mathrm{98}.\mathrm{99}}{\mathrm{2}}\right)\right\} \\ $$$$\:\:\:\:\:\mathrm{Account}\:\mathrm{of}\:\mathrm{2}^{\mathrm{6}} :\:\mathrm{One}\:\mathrm{2}\:\mathrm{comes}\:\mathrm{in}\:\mathrm{read}\:\mathrm{bracket}\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{5}\:\:\:\:\mathrm{2}'\mathrm{s}\:\:\:\mathrm{come}\:\mathrm{in}\:\mathrm{five}\:\mathrm{blue}\:\mathrm{brackets} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{one}\:\mathrm{blue}\:\mathrm{bracket}\:\mathrm{is}\:\:\mathrm{per}\:\mathrm{each}\:\mathrm{20}\right) \\ $$$$\mathrm{Now}\:\mathrm{we}\:\mathrm{can}\:\mathrm{verify}/\mathrm{prove}\:\mathrm{the}\:\mathrm{following}\:\mathrm{congruences}: \\ $$$$\frac{\mathrm{1}.\mathrm{2}.\mathrm{3}.\mathrm{4}}{\mathrm{2}}\equiv\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\left.\begin{matrix}{\mathrm{6}.\mathrm{7}.\mathrm{8}.\mathrm{9}}\\{\vdots}\\{\mathrm{91}.\mathrm{92}.\mathrm{93}.\mathrm{94}}\end{matrix}\right\}\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right)\:\:\:\left[\mathrm{14}\:\mathrm{congruencs}\right] \\ $$$$\left(\mathrm{5k}+\mathrm{1}\right)\left(\mathrm{5k}+\mathrm{2}\right)\left(\mathrm{5k}+\mathrm{3}\right)\left(\mathrm{5k}+\mathrm{4}\right)\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right)\:\begin{cases}{\:^{\bullet} \mathrm{See}\:\mathrm{Answer}\:\mathrm{of}\:\mathrm{mrW1}\:\rceil}\\{\mathrm{to}\:\mathrm{Q}#\mathrm{14614}\:\mathrm{or}\:\mathrm{an}}\\{\:\mathrm{alternative}\:\mathrm{proof}\:\mathrm{in}}\\{\mathrm{the}\:\mathrm{comment}\:\mathrm{below}.}\end{cases} \\ $$$$\left.\begin{matrix}{\left(\mathrm{16}.\mathrm{17}.\mathrm{18}.\mathrm{19}\right)/\mathrm{2}}\\{\vdots}\\{\left(\mathrm{96}.\mathrm{97}.\mathrm{98}.\mathrm{99}\right)/\mathrm{2}}\end{matrix}\right\}\equiv\mathrm{2}\:\:\:\left[\mathrm{5}\:\mathrm{congruences}\right] \\ $$$$\left\{\left(\mathrm{5k}+\mathrm{1}\right)\left(\mathrm{5k}+\mathrm{2}\right)\left(\mathrm{5k}+\mathrm{3}\right)\left(\mathrm{5k}+\mathrm{4}\right)\right\}/\mathrm{2}\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right)\begin{cases}{\:^{\ast} \mathrm{Fo}\:\mathrm{proof}\:\mathrm{see}\:\mathrm{the}}\\{\mathrm{comment}\:\mathrm{below}.}\end{cases} \\ $$$$\mathrm{Multiplying}\:\mathrm{all}\:\mathrm{the}\:\mathrm{above}\:\mathrm{congruences} \\ $$$$\mathrm{p}\equiv\mathrm{2}.\mathrm{4}^{\mathrm{14}} .\mathrm{2}^{\mathrm{5}} \left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\:\:\:\:\mathrm{4}^{\mathrm{14}} \equiv\mathrm{6}\left(\mathrm{mod}\:\mathrm{10}\right)\:\:\left[\mathrm{4}^{\mathrm{2k}} \equiv\mathrm{6}\left(\mathrm{mod}\:\mathrm{10}\right)\right]\: \\ $$$$\:\:\:\:\mathrm{2}^{\mathrm{5}} \equiv\mathrm{32}\equiv\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{p}\equiv\mathrm{2}.\mathrm{6}.\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{p}\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{Hence}\:\mathrm{the}\:\mathrm{require}\:\mathrm{non}-\mathrm{zero}\:\mathrm{digit}\:\mathrm{is}\:\mathrm{4} \\ $$$$\:\boldsymbol{\mathrm{It}}'\boldsymbol{\mathrm{s}}\:\:\boldsymbol{\mathrm{only}}\:\boldsymbol{\mathrm{accedent}}\:\boldsymbol{\mathrm{that}}\:\boldsymbol{\mathrm{the}}\:\boldsymbol{\mathrm{answer}}\:\:\:\:\:\:\:\:\: \\ $$$$\boldsymbol{\mathrm{is}}\:\boldsymbol{\mathrm{correct}}!\:\boldsymbol{\mathrm{Actually}}\:\boldsymbol{\mathrm{there}}'\boldsymbol{\mathrm{s}}\:\boldsymbol{\mathrm{a}}\:\boldsymbol{\mathrm{fault}}\:\boldsymbol{\mathrm{in}} \\ $$$$\boldsymbol{\mathrm{the}}\:\:\boldsymbol{\mathrm{logic}}.\:\boldsymbol{\mathrm{I}}'\boldsymbol{\mathrm{ll}}\:\boldsymbol{\mathrm{try}}\:\boldsymbol{\mathrm{to}}\:\boldsymbol{\mathrm{correct}}\:\boldsymbol{\mathrm{my}}\:\boldsymbol{\mathrm{logic}}. \\ $$$$\boldsymbol{\mathrm{Please}}\:\boldsymbol{\mathrm{stay}}\:\boldsymbol{\mathrm{with}}\:\boldsymbol{\mathrm{me}}! \\ $$

Commented by RasheedSoomro last updated on 09/Jun/17

•(5k+1)(5k+2)(5k+3)(5k+4)(mod 10)=4 Case-1: k is even.let it′s equal to 2m ∀m∈Z =(5(2m)+1)(5(2m)+2)(5(2m)+3)(5(2m)+4)(mod 10) =10000m^4 +10000m^3 +3500m^2 +500m+24(mod 10) =(10000m^4 +10000m^3 +3500m^2 +500m+20)+4(mod 10) =10(1000m^4 +1000m^3 +350m^2 +50m+2)+4(mod 10) =4(mod 10) Case-2:k is odd.Let it′s equal to 2m+1 (5(2m+1)+1)(5(2m+1)+2)(5(2m+1)+3)(5(2m+1)+4)(mod 10) (10m+6)(10m+7)(10m+8)(10m+9)(mod 10) 10000m^4 +30000m^3 +33500m^2 +16500m+3024(mod 10) (10000m^4 +30000m^3 +33500m^2 +16500m+3020)+4(mod 10) 10(1000m^4 +3000m^3 +3350m^2 +1650m+302)+4(mod 10) =4(mod 10 Hence for all k∈Z ,the proposition itrue. ∗{(5k+1)(5k+2)(5k+3)(5k+4)/2} (mod 10)=2 Case-1:k ieven.Let it′s equal to 2m ={(5(2m)+1)(5(2m)+2)(5(2m)+3)(5(2m)+4)/2}(mod 10) =5000m^4 +5000m^3 +1750m^2 +250m+12(mod 10) =10(500m^4 +500m^3 +175m^2 +25m+1)+2(mod 10) =2(mod 10) Case-2:k is odd.Let it′s equal to 2m+1 =(5(2m+1)+1)(5(2m+1)+2)(5(2m+1)+3)(5(2m+1)+4)/2(mod 10) =(10m+6)(10m+7)(10m+8)(10m+9)/2(mod 10) =(10000m^4 +30000m^3 +33500m^2 +16500m+3024)/2(mod 10) =5000m^4 +15000m^3 +16750m^2 +8250m+1512(mod 10) =(5000m^4 +15000m^3 +16750m^2 +8250m+1510)+2(mod 2) =2(mod 2)

$$\:\bullet\left(\mathrm{5k}+\mathrm{1}\right)\left(\mathrm{5k}+\mathrm{2}\right)\left(\mathrm{5k}+\mathrm{3}\right)\left(\mathrm{5k}+\mathrm{4}\right)\left(\mathrm{mod}\:\mathrm{10}\right)=\mathrm{4} \\ $$$$\mathrm{Case}-\mathrm{1}:\:\mathrm{k}\:\mathrm{is}\:\mathrm{even}.\mathrm{let}\:\mathrm{it}'\mathrm{s}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{2m}\:\:\forall\mathrm{m}\in\mathbb{Z} \\ $$$$=\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{1}\right)\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{2}\right)\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{3}\right)\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{4}\right)\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{10000m}^{\mathrm{4}} +\mathrm{10000m}^{\mathrm{3}} +\mathrm{3500m}^{\mathrm{2}} +\mathrm{500m}+\mathrm{24}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\left(\mathrm{10000m}^{\mathrm{4}} +\mathrm{10000m}^{\mathrm{3}} +\mathrm{3500m}^{\mathrm{2}} +\mathrm{500m}+\mathrm{20}\right)+\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{10}\left(\mathrm{1000m}^{\mathrm{4}} +\mathrm{1000m}^{\mathrm{3}} +\mathrm{350m}^{\mathrm{2}} +\mathrm{50m}+\mathrm{2}\right)+\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right)\:\: \\ $$$$\mathrm{Case}-\mathrm{2}:\mathrm{k}\:\mathrm{is}\:\mathrm{odd}.\mathrm{Let}\:\mathrm{it}'\mathrm{s}\:\mathrm{equal}\:\mathrm{to}\:\mathrm{2m}+\mathrm{1} \\ $$$$\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{1}\right)\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{2}\right)\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{3}\right)\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{4}\right)\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\left(\mathrm{10m}+\mathrm{6}\right)\left(\mathrm{10m}+\mathrm{7}\right)\left(\mathrm{10m}+\mathrm{8}\right)\left(\mathrm{10m}+\mathrm{9}\right)\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{10000m}^{\mathrm{4}} +\mathrm{30000m}^{\mathrm{3}} +\mathrm{33500m}^{\mathrm{2}} +\mathrm{16500m}+\mathrm{3024}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\left(\mathrm{10000m}^{\mathrm{4}} +\mathrm{30000m}^{\mathrm{3}} +\mathrm{33500m}^{\mathrm{2}} +\mathrm{16500m}+\mathrm{3020}\right)+\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{10}\left(\mathrm{1000m}^{\mathrm{4}} +\mathrm{3000m}^{\mathrm{3}} +\mathrm{3350m}^{\mathrm{2}} +\mathrm{1650m}+\mathrm{302}\right)+\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\:\:\:\right. \\ $$$$\mathrm{Hence}\:\mathrm{for}\:\mathrm{all}\:\mathrm{k}\in\mathbb{Z}\:,\mathrm{the}\:\mathrm{proposition}\:\mathrm{itrue}. \\ $$$$ \\ $$$$\:\ast\left\{\left(\mathrm{5k}+\mathrm{1}\right)\left(\mathrm{5k}+\mathrm{2}\right)\left(\mathrm{5k}+\mathrm{3}\right)\left(\mathrm{5k}+\mathrm{4}\right)/\mathrm{2}\right\}\:\:\left(\mathrm{mod}\:\mathrm{10}\right)=\mathrm{2} \\ $$$$\:\mathrm{Case}-\mathrm{1}:\mathrm{k}\:\mathrm{ieven}.\mathrm{Let}\:\mathrm{it}'\mathrm{s}\:\mathrm{equal}\:\mathrm{to}\:\:\mathrm{2m} \\ $$$$=\left\{\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{1}\right)\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{2}\right)\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{3}\right)\left(\mathrm{5}\left(\mathrm{2m}\right)+\mathrm{4}\right)/\mathrm{2}\right\}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{5000m}^{\mathrm{4}} +\mathrm{5000m}^{\mathrm{3}} +\mathrm{1750m}^{\mathrm{2}} +\mathrm{250m}+\mathrm{12}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{10}\left(\mathrm{500m}^{\mathrm{4}} +\mathrm{500m}^{\mathrm{3}} +\mathrm{175m}^{\mathrm{2}} +\mathrm{25m}+\mathrm{1}\right)+\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\:\mathrm{Case}-\mathrm{2}:\mathrm{k}\:\mathrm{is}\:\mathrm{odd}.\mathrm{Let}\:\mathrm{it}'\mathrm{s}\:\mathrm{equal}\:\mathrm{to}\:\:\mathrm{2m}+\mathrm{1} \\ $$$$=\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{1}\right)\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{2}\right)\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{3}\right)\left(\mathrm{5}\left(\mathrm{2m}+\mathrm{1}\right)+\mathrm{4}\right)/\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\left(\mathrm{10m}+\mathrm{6}\right)\left(\mathrm{10m}+\mathrm{7}\right)\left(\mathrm{10m}+\mathrm{8}\right)\left(\mathrm{10m}+\mathrm{9}\right)/\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\left(\mathrm{10000m}^{\mathrm{4}} +\mathrm{30000m}^{\mathrm{3}} +\mathrm{33500m}^{\mathrm{2}} +\mathrm{16500m}+\mathrm{3024}\right)/\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\mathrm{5000m}^{\mathrm{4}} +\mathrm{15000m}^{\mathrm{3}} +\mathrm{16750m}^{\mathrm{2}} +\mathrm{8250m}+\mathrm{1512}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$=\left(\mathrm{5000m}^{\mathrm{4}} +\mathrm{15000m}^{\mathrm{3}} +\mathrm{16750m}^{\mathrm{2}} +\mathrm{8250m}+\mathrm{1510}\right)+\mathrm{2}\left(\mathrm{mod}\:\mathrm{2}\right) \\ $$$$=\mathrm{2}\left(\mathrm{mod}\:\mathrm{2}\right) \\ $$

Commented by mrW1 last updated on 09/Jun/17

(5k+1)(5k+2)(5k+3)(5k+4) =(5k+1)(5k+4)(5k+3)(5k+2) = [(5k)^2 +5(5k)+4][(5k)^2 +5(5k)+6] =[(5k)^2 +5(5k)]^2 +10[(5k)^2 +5(5k)]+24 =625[k(k+1)]^2 +250[k(k+1)]+24 =2500[((k(k+1))/2)]^2 +500[((k(k+1))/2)]+24 since one of k and k+1 is even, ((k(k+1))/2) is integer ⇒4 (mod 10)

$$\left(\mathrm{5k}+\mathrm{1}\right)\left(\mathrm{5k}+\mathrm{2}\right)\left(\mathrm{5k}+\mathrm{3}\right)\left(\mathrm{5k}+\mathrm{4}\right) \\ $$$$=\left(\mathrm{5k}+\mathrm{1}\right)\left(\mathrm{5k}+\mathrm{4}\right)\left(\mathrm{5k}+\mathrm{3}\right)\left(\mathrm{5k}+\mathrm{2}\right) \\ $$$$=\:\left[\left(\mathrm{5k}\right)^{\mathrm{2}} +\mathrm{5}\left(\mathrm{5k}\right)+\mathrm{4}\right]\left[\left(\mathrm{5k}\right)^{\mathrm{2}} +\mathrm{5}\left(\mathrm{5k}\right)+\mathrm{6}\right] \\ $$$$=\left[\left(\mathrm{5k}\right)^{\mathrm{2}} +\mathrm{5}\left(\mathrm{5k}\right)\right]^{\mathrm{2}} +\mathrm{10}\left[\left(\mathrm{5k}\right)^{\mathrm{2}} +\mathrm{5}\left(\mathrm{5k}\right)\right]+\mathrm{24} \\ $$$$=\mathrm{625}\left[\mathrm{k}\left(\mathrm{k}+\mathrm{1}\right)\right]^{\mathrm{2}} +\mathrm{250}\left[\mathrm{k}\left(\mathrm{k}+\mathrm{1}\right)\right]+\mathrm{24} \\ $$$$=\mathrm{2500}\left[\frac{\mathrm{k}\left(\mathrm{k}+\mathrm{1}\right)}{\mathrm{2}}\right]^{\mathrm{2}} +\mathrm{500}\left[\frac{\mathrm{k}\left(\mathrm{k}+\mathrm{1}\right)}{\mathrm{2}}\right]+\mathrm{24} \\ $$$$\mathrm{since}\:\mathrm{one}\:\mathrm{of}\:\mathrm{k}\:\mathrm{and}\:\mathrm{k}+\mathrm{1}\:\mathrm{is}\:\mathrm{even}, \\ $$$$\frac{\mathrm{k}\left(\mathrm{k}+\mathrm{1}\right)}{\mathrm{2}}\:\mathrm{is}\:\mathrm{integer} \\ $$$$\Rightarrow\mathrm{4}\:\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$

Commented by RasheedSoomro last updated on 09/Jun/17

Your method is better Sir! It was also used in your answer of Q#14614. I merely tried to use different approach!

$$\mathrm{Your}\:\mathrm{method}\:\mathrm{is}\:\mathrm{better}\:\mathrm{Sir}!\:\mathrm{It}\:\mathrm{was}\:\mathrm{also}\:\mathrm{used} \\ $$$$\:\mathrm{in}\:\mathrm{your}\:\mathrm{answer}\:\mathrm{of}\:\mathrm{Q}#\mathrm{14614}. \\ $$$$\mathrm{I}\:\mathrm{merely}\:\mathrm{tried}\:\mathrm{to}\:\mathrm{use}\:\mathrm{different}\:\mathrm{approach}! \\ $$

Commented by mrW1 last updated on 09/Jun/17

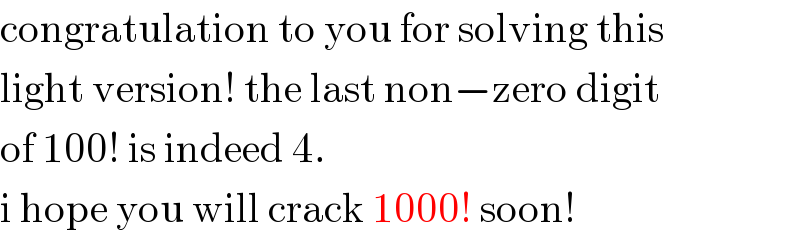
congratulation to you for solving this light version! the last non−zero digit of 100! is indeed 4. i hope you will crack 1000! soon!

$$\mathrm{congratulation}\:\mathrm{to}\:\mathrm{you}\:\mathrm{for}\:\mathrm{solving}\:\mathrm{this} \\ $$$$\mathrm{light}\:\mathrm{version}!\:\mathrm{the}\:\mathrm{last}\:\mathrm{non}−\mathrm{zero}\:\mathrm{digit} \\ $$$$\mathrm{of}\:\mathrm{100}!\:\mathrm{is}\:\mathrm{indeed}\:\mathrm{4}. \\ $$$$\mathrm{i}\:\mathrm{hope}\:\mathrm{you}\:\mathrm{will}\:\mathrm{crack}\:\mathrm{1000}!\:\mathrm{soon}! \\ $$

Commented by RasheedSoomro last updated on 09/Jun/17

Please help me in finding error in answer of Q#13724. In one of its answers I have used same logic. Bu the answer is wrong! Mr prakash jain & mrW1 Do you know any standard method of such questions? If you do please do share.

$$\:\mathrm{Please}\:\mathrm{help}\:\mathrm{me}\:\mathrm{in}\:\mathrm{finding}\:\mathrm{error}\:\mathrm{in}\:\mathrm{answer}\:\mathrm{of}\:\mathrm{Q}#\mathrm{13724}. \\ $$$$\mathrm{In}\:\mathrm{one}\:\mathrm{of}\:\mathrm{its}\:\mathrm{answers}\:\mathrm{I}\:\mathrm{have}\:\mathrm{used}\:\mathrm{same}\:\mathrm{logic}. \\ $$$$\mathrm{Bu}\:\mathrm{the}\:\mathrm{answer}\:\mathrm{is}\:\mathrm{wrong}! \\ $$$$\mathrm{Mr}\:\mathrm{prakash}\:\mathrm{jain}\:\&\:\mathrm{mrW1} \\ $$$$\mathrm{Do}\:\mathrm{you}\:\mathrm{know}\:\mathrm{any}\:\mathrm{standard}\:\mathrm{method}\:\mathrm{of}\:\mathrm{such}\:\mathrm{questions}? \\ $$$$\mathrm{If}\:\mathrm{you}\:\mathrm{do}\:\mathrm{please}\:\mathrm{do}\:\mathrm{share}. \\ $$

Commented by mrW1 last updated on 09/Jun/17

I definitively don′t know any standard methods for solving such questions. I am learning, also from posts such like yours.

$$\mathrm{I}\:\mathrm{definitively}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{any}\:\mathrm{standard} \\ $$$$\mathrm{methods}\:\mathrm{for}\:\mathrm{solving}\:\mathrm{such}\:\mathrm{questions}. \\ $$$$\mathrm{I}\:\mathrm{am}\:\mathrm{learning},\:\mathrm{also}\:\mathrm{from}\:\mathrm{posts}\:\mathrm{such}\: \\ $$$$\mathrm{like}\:\mathrm{yours}. \\ $$

Commented by prakash jain last updated on 10/Jun/17

Actually I don′t know a short cut method to this question. I posted this question to get some ideas.

$$\mathrm{Actually}\:\mathrm{I}\:\mathrm{don}'\mathrm{t}\:\mathrm{know}\:\mathrm{a}\:\mathrm{short}\:\mathrm{cut} \\ $$$$\mathrm{method}\:\mathrm{to}\:\mathrm{this}\:\mathrm{question}. \\ $$$$\mathrm{I}\:\mathrm{posted}\:\mathrm{this}\:\mathrm{question}\:\mathrm{to}\:\mathrm{get}\:\mathrm{some} \\ $$$$\mathrm{ideas}. \\ $$

Commented by RasheedSoomro last updated on 10/Jun/17

Thanks of you both!

$$\mathcal{T}{hanks}\:\mathrm{of}\:\mathrm{you}\:\mathrm{both}! \\ $$

Answered by RasheedSoomro last updated on 11/Jun/17

Easy way 100! has 24 0′s from right. p=((100!)/(10^(24) )) has no zeo from right ∴ first non-zero digit in 100! from right = unit digit in p ∴ p≡d(mod 10) p=((100!)/(10^(24) ))=((100!)/(2^(24) ×5^(24) ))=((100!)/(2^6 (5.10....100))) [18 2′s from 2^(24) have come in 5.10....100] =(1/2^6 ){(1.2.3.4)(6.7.8.9)...(96.97.98.99} p≡d(mod 10)⇒ (1/2^6 ){(1.2.3.4)(6.7.8.9)...(96.97.98.99}≡d(mod 10) (1/2^6 )(4)^(20) ≡d(mod 10) [∵ Each bracket≡4(mod 10) Total brackets=20] (2^(40) /2^6 )≡d(mod 10) d≡2^(34) (mod 10) Verify that: 2^4 ≡6(mod 10 (2^4 )^8 ≡6^8 ≡6(mod 10) 2^(32) .2^2 ≡6.2^2 ≡4(mod 10) 2^(34) ≡4(mod 10) Hence d≡2^(34) ≡4(mod 10) d≡4(mod 10) Wrong logic! Accedently correct answer! I′ll try to correct my logic. Please stay with me!

$$\mathrm{Easy}\:\mathrm{way} \\ $$$$\mathrm{100}!\:\mathrm{has}\:\mathrm{24}\:\:\mathrm{0}'\mathrm{s}\:\mathrm{from}\:\mathrm{right}.\: \\ $$$$\mathrm{p}=\frac{\mathrm{100}!}{\mathrm{10}^{\mathrm{24}} }\:\:\mathrm{has}\:\mathrm{no}\:\mathrm{zeo}\:\mathrm{from}\:\mathrm{right} \\ $$$$\therefore\:\:\mathrm{first}\:\mathrm{non}-\mathrm{zero}\:\mathrm{digit}\:\mathrm{in}\:\mathrm{100}!\:\mathrm{from}\:\mathrm{right} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\:\mathrm{unit}\:\mathrm{digit}\:\mathrm{in}\:\mathrm{p} \\ $$$$\therefore\:\:\mathrm{p}\equiv\mathrm{d}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{p}=\frac{\mathrm{100}!}{\mathrm{10}^{\mathrm{24}} }=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{24}} ×\mathrm{5}^{\mathrm{24}} }=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{6}} \left(\mathrm{5}.\mathrm{10}....\mathrm{100}\right)} \\ $$$$\left[\mathrm{18}\:\:\:\mathrm{2}'\mathrm{s}\:\mathrm{from}\:\mathrm{2}^{\mathrm{24}} \:\mathrm{have}\:\mathrm{come}\:\mathrm{in}\:\mathrm{5}.\mathrm{10}....\mathrm{100}\right] \\ $$$$\:\:\:=\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{6}} }\left\{\left(\mathrm{1}.\mathrm{2}.\mathrm{3}.\mathrm{4}\right)\left(\mathrm{6}.\mathrm{7}.\mathrm{8}.\mathrm{9}\right)...\left(\mathrm{96}.\mathrm{97}.\mathrm{98}.\mathrm{99}\right\}\right. \\ $$$$\:\:\:\:\mathrm{p}\equiv\mathrm{d}\left(\mathrm{mod}\:\mathrm{10}\right)\Rightarrow \\ $$$$\:\:\:\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{6}} }\left\{\left(\mathrm{1}.\mathrm{2}.\mathrm{3}.\mathrm{4}\right)\left(\mathrm{6}.\mathrm{7}.\mathrm{8}.\mathrm{9}\right)...\left(\mathrm{96}.\mathrm{97}.\mathrm{98}.\mathrm{99}\right\}\equiv\mathrm{d}\left(\mathrm{mod}\:\mathrm{10}\right)\right. \\ $$$$\:\:\:\:\frac{\mathrm{1}}{\mathrm{2}^{\mathrm{6}} }\left(\mathrm{4}\right)^{\mathrm{20}} \equiv\mathrm{d}\left(\mathrm{mod}\:\mathrm{10}\right)\:\:\:\:\left[\because\:\mathrm{Each}\:\mathrm{bracket}\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right)\:\mathrm{Total}\:\mathrm{brackets}=\mathrm{20}\right] \\ $$$$\:\:\:\frac{\mathrm{2}^{\mathrm{40}} }{\mathrm{2}^{\mathrm{6}} }\equiv\mathrm{d}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\:\:\:\:\:\mathrm{d}\equiv\mathrm{2}^{\mathrm{34}} \left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{Verify}\:\mathrm{that}: \\ $$$$\:\:\:\:\:\:\:\mathrm{2}^{\mathrm{4}} \equiv\mathrm{6}\left(\mathrm{mod}\:\mathrm{10}\right. \\ $$$$\:\:\:\:\:\:\:\left(\mathrm{2}^{\mathrm{4}} \right)^{\mathrm{8}} \equiv\mathrm{6}^{\mathrm{8}} \equiv\mathrm{6}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{2}^{\mathrm{32}} .\mathrm{2}^{\mathrm{2}} \equiv\mathrm{6}.\mathrm{2}^{\mathrm{2}} \equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\:\:\:\:\:\:\:\:\mathrm{2}^{\mathrm{34}} \equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{Hence} \\ $$$$\:\:\:\:\:\:\:\:\mathrm{d}\equiv\mathrm{2}^{\mathrm{34}} \equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\:\:\:\:\:\:\:\:\mathrm{d}\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$ \\ $$$$\:\boldsymbol{\mathrm{Wrong}}\:\boldsymbol{\mathrm{logic}}!\:\boldsymbol{\mathrm{Accedently}}\:\boldsymbol{\mathrm{correct}}\:\boldsymbol{\mathrm{answer}}!\:\:\:\:\:\:\: \\ $$$$\boldsymbol{\mathrm{I}}'\boldsymbol{\mathrm{ll}}\:\boldsymbol{\mathrm{try}}\:\boldsymbol{\mathrm{to}}\:\boldsymbol{\mathrm{correct}}\:\boldsymbol{\mathrm{my}}\:\boldsymbol{\mathrm{logic}}. \\ $$$$\boldsymbol{\mathrm{Please}}\:\boldsymbol{\mathrm{stay}}\:\boldsymbol{\mathrm{with}}\:\boldsymbol{\mathrm{me}}! \\ $$

Answered by RasheedSoomro last updated on 11/Jun/17

According to Corrected Logic. Right logic & Right answer Expansion of 100! have 24 0′s in the right. Let p=((100!)/(10^(24) )) p has no zero from right. First non-zero digit in 100! =unit digit in p d≡p(mod 10) p=((100!)/(10^(24) ))=((100!)/(2^(24) ×5^(24) )) =((100!)/(2^(24) ×(5×((10)/2)×((15)/3)×((20)/2^2 )×5^2 ×...×((100)/2^2 )))) =((100!)/(2^(24) ×(((5.10.15.....100)/(2^(18) ×3^8 ×7^2 ×11×13×17×19))))) =3^8 ×7^2 ×11×13×17×19×((100!)/(2^6 (5.10....100))) =3^8 ×7^2 ×11×13×17×19{(((1...4)/2))(6...9)(11...14)(((16....19)/2))} ×...×{(81....84)(86...89)(91...94)(((96...99)/2))} Number of red bracket= 1 Number of black brackets= 14 3 per each 20 except first. Number of blue brackets= 5 1 per each 20 We can verify/prove the following congruences 3^8 ≡1(mod 10) 7^2 ≡9(mod 10) 11≡1(mod 10) 13≡3(mod 10) 17≡7(mod 10) 19≡9(mod 10) ((1.2.3.4)/2)≡2 Any of Black-bracket≡4(mod 10) [14 congruences] Any of Blue-bracket≡2(mod 10) [ 5 congruences] Multiplying all above congruences p≡1.9.1.3.7.9.2.4^(14) .2^5 (mod 10) p≡1.9.1.3.7.9.2.6.2(mod 10) [∵4^(even) ≡6(mod 10] p≡40824≡4(mod 10) [∵4^(even) ≡6(mod 10]

$$\mathrm{According}\:\mathrm{to}\:\mathrm{Corrected}\:\mathrm{Logic}. \\ $$$$\mathrm{Right}\:\mathrm{logic}\:\&\:\:\mathrm{Right}\:\mathrm{answer} \\ $$$$\:\:\mathcal{E}\mathrm{xpansion}\:\mathrm{of}\:\mathrm{100}!\:\mathrm{have}\:\mathrm{24}\:\:\:\mathrm{0}'\mathrm{s}\:\mathrm{in}\:\mathrm{the}\:\mathrm{right}. \\ $$$$\mathrm{Let}\:\mathrm{p}=\frac{\mathrm{100}!}{\mathrm{10}^{\mathrm{24}} } \\ $$$$\mathrm{p}\:\mathrm{has}\:\mathrm{no}\:\mathrm{zero}\:\mathrm{from}\:\mathrm{right}. \\ $$$$\mathrm{First}\:\mathrm{non}-\mathrm{zero}\:\mathrm{digit}\:\mathrm{in}\:\mathrm{100}! \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{unit}\:\mathrm{digit}\:\mathrm{in}\:\mathrm{p} \\ $$$$\:\:\:\:\:\:\:\mathrm{d}\equiv\mathrm{p}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{p}=\frac{\mathrm{100}!}{\mathrm{10}^{\mathrm{24}} }=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{24}} ×\mathrm{5}^{\mathrm{24}} } \\ $$$$\:\:\:\:=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{24}} ×\left(\mathrm{5}×\frac{\mathrm{10}}{\mathrm{2}}×\frac{\mathrm{15}}{\mathrm{3}}×\frac{\mathrm{20}}{\mathrm{2}^{\mathrm{2}} }×\mathrm{5}^{\mathrm{2}} ×...×\frac{\mathrm{100}}{\mathrm{2}^{\mathrm{2}} }\right)} \\ $$$$\:\:\:\:=\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{24}} ×\left(\frac{\mathrm{5}.\mathrm{10}.\mathrm{15}.....\mathrm{100}}{\mathrm{2}^{\mathrm{18}} ×\mathrm{3}^{\mathrm{8}} ×\mathrm{7}^{\mathrm{2}} ×\mathrm{11}×\mathrm{13}×\mathrm{17}×\mathrm{19}}\right)} \\ $$$$\:\:\:=\mathrm{3}^{\mathrm{8}} ×\mathrm{7}^{\mathrm{2}} ×\mathrm{11}×\mathrm{13}×\mathrm{17}×\mathrm{19}×\frac{\mathrm{100}!}{\mathrm{2}^{\mathrm{6}} \left(\mathrm{5}.\mathrm{10}....\mathrm{100}\right)} \\ $$$$\:=\mathrm{3}^{\mathrm{8}} ×\mathrm{7}^{\mathrm{2}} ×\mathrm{11}×\mathrm{13}×\mathrm{17}×\mathrm{19}\left\{\left(\frac{\mathrm{1}...\mathrm{4}}{\mathrm{2}}\right)\left(\mathrm{6}...\mathrm{9}\right)\left(\mathrm{11}...\mathrm{14}\right)\left(\frac{\mathrm{16}....\mathrm{19}}{\mathrm{2}}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:×...×\left\{\left(\mathrm{81}....\mathrm{84}\right)\left(\mathrm{86}...\mathrm{89}\right)\left(\mathrm{91}...\mathrm{94}\right)\left(\frac{\mathrm{96}...\mathrm{99}}{\mathrm{2}}\right)\right\} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{Number}\:\mathrm{of}\:\mathrm{red}\:\mathrm{bracket}=\:\:\:\mathrm{1} \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{Number}\:\mathrm{of}\:\mathrm{black}\:\mathrm{brackets}=\:\:\:\mathrm{14}\:\:\:\mathrm{3}\:\mathrm{per}\:\mathrm{each}\:\:\mathrm{20}\:\mathrm{except}\:\mathrm{first}. \\ $$$$\:\:\:\:\:\:\:\:\:\mathrm{Number}\:\mathrm{of}\:\mathrm{blue}\:\mathrm{brackets}=\:\:\:\mathrm{5}\:\:\:\:\mathrm{1}\:\mathrm{per}\:\mathrm{each}\:\:\mathrm{20} \\ $$$$\mathrm{We}\:\mathrm{can}\:\mathrm{verify}/\mathrm{prove}\:\mathrm{the}\:\mathrm{following}\:\mathrm{congruences} \\ $$$$\mathrm{3}^{\mathrm{8}} \equiv\mathrm{1}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{7}^{\mathrm{2}} \equiv\mathrm{9}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{11}\equiv\mathrm{1}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{13}\equiv\mathrm{3}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{17}\equiv\mathrm{7}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{19}\equiv\mathrm{9}\left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\frac{\mathrm{1}.\mathrm{2}.\mathrm{3}.\mathrm{4}}{\mathrm{2}}\equiv\mathrm{2} \\ $$$$\mathrm{Any}\:\mathrm{of}\:\boldsymbol{\mathrm{Black}}-\boldsymbol{\mathrm{bracket}}\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right)\:\:\:\:\left[\mathrm{14}\:\mathrm{congruences}\right] \\ $$$$\mathrm{Any}\:\mathrm{of}\:\boldsymbol{\mathrm{Blue}}-\boldsymbol{\mathrm{bracket}}\equiv\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right)\:\:\:\:\left[\:\mathrm{5}\:\mathrm{congruences}\right] \\ $$$$ \\ $$$$\mathrm{Multiplying}\:\mathrm{all}\:\mathrm{above}\:\mathrm{congruences} \\ $$$$\mathrm{p}\equiv\mathrm{1}.\mathrm{9}.\mathrm{1}.\mathrm{3}.\mathrm{7}.\mathrm{9}.\mathrm{2}.\mathrm{4}^{\mathrm{14}} .\mathrm{2}^{\mathrm{5}} \left(\mathrm{mod}\:\mathrm{10}\right) \\ $$$$\mathrm{p}\equiv\mathrm{1}.\mathrm{9}.\mathrm{1}.\mathrm{3}.\mathrm{7}.\mathrm{9}.\mathrm{2}.\mathrm{6}.\mathrm{2}\left(\mathrm{mod}\:\mathrm{10}\right)\:\:\:\left[\because\mathrm{4}^{\mathrm{even}} \equiv\mathrm{6}\left(\mathrm{mod}\:\mathrm{10}\right]\right. \\ $$$$\mathrm{p}\equiv\mathrm{40824}\equiv\mathrm{4}\left(\mathrm{mod}\:\mathrm{10}\right)\:\:\:\left[\because\mathrm{4}^{\mathrm{even}} \equiv\mathrm{6}\left(\mathrm{mod}\:\mathrm{10}\right]\right. \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com