Question and Answers Forum

All Questions      Topic List

None Questions

Previous in All Question      Next in All Question      

Previous in None      Next in None      

Question Number 1539 by 314159 last updated on 17/Aug/15

$$\mathbf{Find}\underset{\mathbf{n}=1}{\sum ^{\mathrm{\infty }}}\frac{1}{{(2\mathbf{n}-1)}^4}.$$

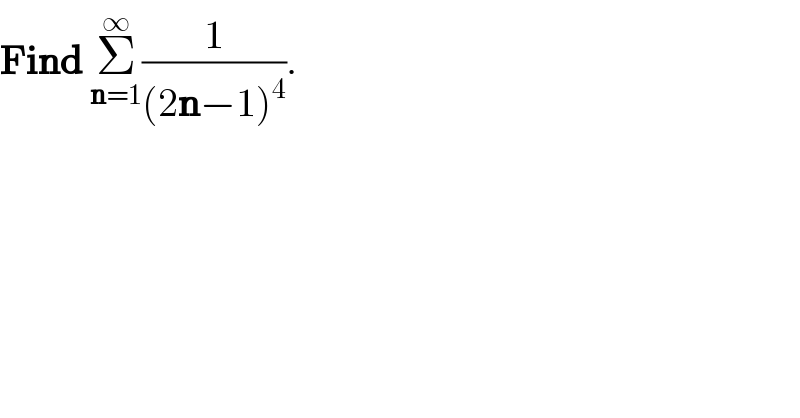
Answered by 123456 last updated on 17/Aug/15

$$\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{n^4}=\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{{(2n-1)}^4}+\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{{\left(2n\right)}^4}$$$$\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{n^4}=\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{{(2n-1)}^4}+\frac{1}{16}\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{n^4}$$$$\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{{(2n-1)}^4}=\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{n^4}-\frac{1}{16}\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{n^4}$$$$\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{{(2n-1)}^4}=\frac{15}{16}\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{n^4}$$$$\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{{(2n-1)}^4}=\frac{15}{16}\times \frac{{\pi }^4}{90}=\frac{15{\pi }^4}{16\times 90}=\frac{5{\pi }^4}{16\times 30}=\frac{{\pi }^4}{16\times 6}=\frac{{\pi }^4}{96}$$$$\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{{(2n-1)}^4}=\frac{{\pi }^4}{96}$$

Commented by 314159 last updated on 17/Aug/15

$$\mathbf{Thank}\mathbf{a}\mathbf{lot}...$$

Commented by 112358 last updated on 17/Aug/15

$$Outofcuriosity,howis$$$$\underset{n=1}{\sum ^{+\mathrm{\infty }}}\frac{1}{n^4}=\frac{{\pi }^4}{90}?$$$$$$

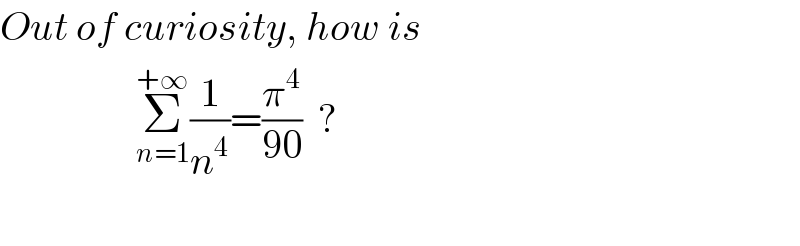
Commented by 123456 last updated on 17/Aug/15

$$\mathrm{rieman}\mathrm{zeta}\mathrm{function},\mathrm{later}\mathrm{i}\mathrm{answer}\mathrm{you}$$

Commented by 112358 last updated on 17/Aug/15

$$Thanks$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com