Question and Answers Forum

All Questions      Topic List

Mechanics Questions

Previous in All Question      Next in All Question      

Previous in Mechanics      Next in Mechanics      

Question Number 154549 by peter frank last updated on 19/Sep/21

A particle is projected with velocity 2(√(gh)) so that it just clears two walls of equal heigh(h) in the t_1 and t_(2 ) respectively.The two walls are at a distance of 2h from each other.If time passing between the two walls is 2(√(h/g)) show that (i) angle projected 60^° (ii)t_1 +t_2 =2(√((3h)/g))

$$\mathrm{A}\:\mathrm{particle}\:\mathrm{is}\:\mathrm{projected}\:\:\mathrm{with}\:\mathrm{velocity} \\ $$$$\mathrm{2}\sqrt{\mathrm{gh}}\:\:\:\mathrm{so}\:\mathrm{that}\:\:\mathrm{it}\:\mathrm{just}\:\mathrm{clears}\:\mathrm{two} \\ $$$$\mathrm{walls}\:\mathrm{of}\:\mathrm{equal}\:\mathrm{heigh}\left(\mathrm{h}\right)\:\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{t}_{\mathrm{1}} \:\mathrm{and}\:\mathrm{t}_{\mathrm{2}\:} \:\mathrm{respectively}.\mathrm{The}\:\mathrm{two} \\ $$$$\mathrm{walls}\:\mathrm{are}\:\mathrm{at}\:\mathrm{a}\:\mathrm{distance}\:\mathrm{of}\:\:\mathrm{2h}\:\:\mathrm{from} \\ $$$$\mathrm{each}\:\mathrm{other}.\mathrm{If}\:\mathrm{time}\:\mathrm{passing}\: \\ $$$$\mathrm{between}\:\mathrm{the}\:\mathrm{two}\:\mathrm{walls}\:\mathrm{is}\:\mathrm{2}\sqrt{\frac{\mathrm{h}}{\mathrm{g}}} \\ $$$$\mathrm{show}\:\mathrm{that}\:\left(\mathrm{i}\right)\:\mathrm{angle}\:\mathrm{projected}\:\mathrm{60}^{°} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{ii}\right)\mathrm{t}_{\mathrm{1}} +\mathrm{t}_{\mathrm{2}} =\mathrm{2}\sqrt{\frac{\mathrm{3h}}{\mathrm{g}}} \\ $$$$ \\ $$

Commented by Tawa11 last updated on 21/Sep/21

nice sir

$$\mathrm{nice}\:\mathrm{sir} \\ $$

Answered by ARUNG_Brandon_MBU last updated on 19/Sep/21

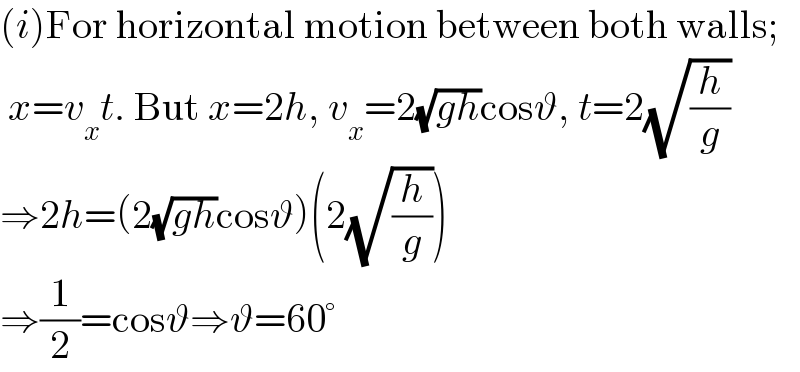
(i)For horizontal motion between both walls; x=v_x t. But x=2h, v_x =2(√(gh))cosϑ, t=2(√(h/g)) ⇒2h=(2(√(gh))cosϑ)(2(√(h/g))) ⇒(1/2)=cosϑ⇒ϑ=60°

$$\left({i}\right)\mathrm{For}\:\mathrm{horizontal}\:\mathrm{motion}\:\mathrm{between}\:\mathrm{both}\:\mathrm{walls}; \\ $$$$\:{x}={v}_{{x}} {t}.\:\mathrm{But}\:{x}=\mathrm{2}{h},\:{v}_{{x}} =\mathrm{2}\sqrt{{gh}}\mathrm{cos}\vartheta,\:{t}=\mathrm{2}\sqrt{\frac{{h}}{{g}}} \\ $$$$\Rightarrow\mathrm{2}{h}=\left(\mathrm{2}\sqrt{{gh}}\mathrm{cos}\vartheta\right)\left(\mathrm{2}\sqrt{\frac{{h}}{{g}}}\right) \\ $$$$\Rightarrow\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{cos}\vartheta\Rightarrow\vartheta=\mathrm{60}° \\ $$

Commented by peter frank last updated on 20/Sep/21

thanks

$$\mathrm{thanks} \\ $$

Answered by ARUNG_Brandon_MBU last updated on 19/Sep/21

(ii) For vertical motion: y=ut+(1/2)at^2 , y=h, u=2(√(gh))sin60°=(√(3gh)), a=−g ⇒h=(√(3gh))t−(1/2)gt^2 ⇒gt^2 −2(√(3gh))t+h=0 t=((2(√(3gh))±(√(12gh−4gh)))/(2g))=((2(√(3gh))±2(√(2gh)))/(2g)) t_1 =(√((3h)/g))+(√((2h)/g)), t_2 =(√((3h)/g))−(√((2h)/g)) t_1 +t_2 =2(√((3h)/g))

$$\left({ii}\right)\:\mathrm{For}\:\mathrm{vertical}\:\mathrm{motion}: \\ $$$${y}={ut}+\frac{\mathrm{1}}{\mathrm{2}}{at}^{\mathrm{2}} ,\:{y}={h},\:{u}=\mathrm{2}\sqrt{{gh}}\mathrm{sin60}°=\sqrt{\mathrm{3}{gh}},\:{a}=−{g} \\ $$$$\Rightarrow{h}=\sqrt{\mathrm{3}{gh}}{t}−\frac{\mathrm{1}}{\mathrm{2}}{gt}^{\mathrm{2}} \Rightarrow{gt}^{\mathrm{2}} −\mathrm{2}\sqrt{\mathrm{3}{gh}}{t}+{h}=\mathrm{0} \\ $$$${t}=\frac{\mathrm{2}\sqrt{\mathrm{3}{gh}}\pm\sqrt{\mathrm{12}{gh}−\mathrm{4}{gh}}}{\mathrm{2}{g}}=\frac{\mathrm{2}\sqrt{\mathrm{3}{gh}}\pm\mathrm{2}\sqrt{\mathrm{2}{gh}}}{\mathrm{2}{g}} \\ $$$${t}_{\mathrm{1}} =\sqrt{\frac{\mathrm{3}{h}}{{g}}}+\sqrt{\frac{\mathrm{2}{h}}{{g}}},\:{t}_{\mathrm{2}} =\sqrt{\frac{\mathrm{3}{h}}{{g}}}−\sqrt{\frac{\mathrm{2}{h}}{{g}}} \\ $$$${t}_{\mathrm{1}} +{t}_{\mathrm{2}} =\mathrm{2}\sqrt{\frac{\mathrm{3}{h}}{{g}}} \\ $$

Answered by peter frank last updated on 20/Sep/21

Answered by peter frank last updated on 20/Sep/21

x−direction x_1 =v_o cos θt_1 x_2 =v_o cos θt_2 v_o =2(√(gh)) x_2 −x_1 =2h (t_2 −t_1 )=2(√(h/g)) v_o cos θ(t_2 −t_1 )=2h cosθ=((2h)/(v_o (t_2 −t_1 ))) =(1/2) θ= cos^(−1) (1/2)=60^° also y−direction y=v_o sin θt_1 −(1/2)gt_1 ^2 y=v_o sin θt_2 −(1/2)gt_2 ^2 v_o sin θt_1 −(1/2)gt_1 ^2 =v_o sin θt_2 −(1/2)gt_2 ^2 2(√(gh)) sin θ(t_2 −t_1 )=(1/2)g(t_2 ^2 −t_1 ^2 ) 2(√(gh)) sin θ=(1/2)g(t_2 +t_1 ) [θ=60] t_2 +t_1 =((4(√(gh)))/g).((√3)/2)= t_2 +t_1 =2(√(((3h)/g) ))

$$\mathrm{x}−\mathrm{direction} \\ $$$$\mathrm{x}_{\mathrm{1}} =\mathrm{v}_{\mathrm{o}} \mathrm{cos}\:\theta\mathrm{t}_{\mathrm{1}} \\ $$$$\mathrm{x}_{\mathrm{2}} =\mathrm{v}_{\mathrm{o}} \mathrm{cos}\:\theta\mathrm{t}_{\mathrm{2}} \\ $$$$\mathrm{v}_{\mathrm{o}} =\mathrm{2}\sqrt{\mathrm{gh}} \\ $$$$\mathrm{x}_{\mathrm{2}} −\mathrm{x}_{\mathrm{1}} =\mathrm{2h}\:\:\:\:\:\:\left(\mathrm{t}_{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} \right)=\mathrm{2}\sqrt{\frac{\mathrm{h}}{\mathrm{g}}} \\ $$$$\mathrm{v}_{\mathrm{o}} \mathrm{cos}\:\theta\left(\mathrm{t}_{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} \right)=\mathrm{2h} \\ $$$$\mathrm{cos}\theta=\frac{\mathrm{2h}}{\mathrm{v}_{\mathrm{o}} \left(\mathrm{t}_{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} \right)}\:\:=\frac{\mathrm{1}}{\mathrm{2}}\:\:\: \\ $$$$\theta=\:\:\mathrm{cos}^{−\mathrm{1}} \frac{\mathrm{1}}{\mathrm{2}}=\mathrm{60}^{°} \\ $$$$\mathrm{also}\:\:\mathrm{y}−\mathrm{direction} \\ $$$$\mathrm{y}=\mathrm{v}_{\mathrm{o}} \mathrm{sin}\:\theta\mathrm{t}_{\mathrm{1}} −\frac{\mathrm{1}}{\mathrm{2}}\mathrm{gt}_{\mathrm{1}} ^{\mathrm{2}} \\ $$$$\mathrm{y}=\mathrm{v}_{\mathrm{o}} \mathrm{sin}\:\theta\mathrm{t}_{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}\mathrm{gt}_{\mathrm{2}} ^{\mathrm{2}} \\ $$$$\mathrm{v}_{\mathrm{o}} \mathrm{sin}\:\theta\mathrm{t}_{\mathrm{1}} −\frac{\mathrm{1}}{\mathrm{2}}\mathrm{gt}_{\mathrm{1}} ^{\mathrm{2}} =\mathrm{v}_{\mathrm{o}} \mathrm{sin}\:\theta\mathrm{t}_{\mathrm{2}} −\frac{\mathrm{1}}{\mathrm{2}}\mathrm{gt}_{\mathrm{2}} ^{\mathrm{2}} \\ $$$$\mathrm{2}\sqrt{\mathrm{gh}}\:\mathrm{sin}\:\theta\left(\mathrm{t}_{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} \right)=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{g}\left(\mathrm{t}_{\mathrm{2}} ^{\mathrm{2}} −\mathrm{t}_{\mathrm{1}} ^{\mathrm{2}} \right) \\ $$$$\mathrm{2}\sqrt{\mathrm{gh}}\:\mathrm{sin}\:\theta=\frac{\mathrm{1}}{\mathrm{2}}\mathrm{g}\left(\mathrm{t}_{\mathrm{2}} +\mathrm{t}_{\mathrm{1}} \right)\:\:\:\left[\theta=\mathrm{60}\right] \\ $$$$\mathrm{t}_{\mathrm{2}} +\mathrm{t}_{\mathrm{1}} =\frac{\mathrm{4}\sqrt{\mathrm{gh}}}{\mathrm{g}}.\frac{\sqrt{\mathrm{3}}}{\mathrm{2}}= \\ $$$$\mathrm{t}_{\mathrm{2}} +\mathrm{t}_{\mathrm{1}} =\mathrm{2}\sqrt{\frac{\mathrm{3h}}{\mathrm{g}}\:}\: \\ $$$$ \\ $$$$ \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com