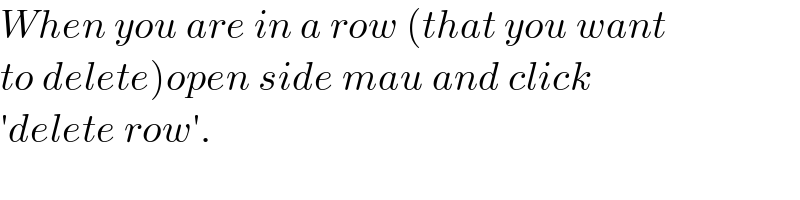Determine all triangle with: 1.The lengths of sides positive integers and at least one is prime number. 2.The semiperimetr is positive integer and area is equal with perimetr.


Question and Answers Forum

All Questions Topic List
Algebra Questions
Previous in All Question Next in All Question
Previous in Algebra Next in Algebra
Question Number 155100 by mathdanisur last updated on 25/Sep/21

$Determine all triangle with :$ $1 . The lengths of sides positive integers$ $and at least one is prime number .$ $2 . The semiperimetr is positive integer$ $and area is equal with perimetr .$
Commented by MJS_new last updated on 25/Sep/21

$if a < b < c there are only 4 I think$ $5 / 12 / 13$ $6 / 25 / 29$ $7 / 15 / 20$ $9 / 10 / 17$ $[{there}^{'} s a 5^{th} one without primes : 6 / 8 / 10]$
Commented by mathdanisur last updated on 25/Sep/21

$perfect my dear thankyou$
	Answered by Rasheed.Sindhi last updated on 26/Sep/21
	$•a,b,c,s=((a+b+c)/2)∈Z^+ ∧ a(say)∈P •(√(s(s−a)(s−b)(s−c))) =a+b+c_(where s=(a+b+c)/2) ((a+b+c)/2)∈Z^+ ⇒a+b+c∈E ⇒ { ((a,b,c all are eve even⇒prime is 2)),((Two of a,b,c are odd,the third is even.)) :} C-1: a,b,c∈E⇒a(prime)=2 b=2m,c=2n ; m,n∈Z^+ s=(2+2m+2n)/2=m+n+1 s−a=1+m+n−2=m+n−1 s−b=1+m+n−2m=−m+n+1 s−c=1+m+n−2n=m−n+1 (√(s(s−a)(s−b)(s−c)))=a+b+c ▶(√((m+n+1)(m+n−1)(−m+n+1)(m−n+1))) =2m+2n+2 ▶ (m+n+1)(m+n−1)(−m+n+1)(m−n+1) =4(m+n+1)^2 ▶ (m+n−1)(−m+n+1)(m−n+1) =4(m+n+1) (o,o)-case:m,n∈O determinant ((((o,o)-case:m,n∈O))) RHS is clearly even. LHS:(o+o−o)(−o+o+o)(o−o+o) =(e−o)(e+o)(e+o) =(o)(o)(o)=o LHS is odd. Contradiction. determinant ((((e,e)-case:m,n∈E))) RHS=even LHS:(e+e−o)(−e+e+o)(e−e+o) (e−o)(e+o)(e+o) (o)(o)(o)=o Contradiction. determinant ((((e,o) or (o,e)-case:m∈E,n∈O))) Continue$
	$∙ a, b, c, s = \frac{a + b + c}{2} \in Z^{+} \land a (s a y) \in P$ $∙ \underset{w h e r e s = (a + b + c) / 2}{\sqrt{s (s - a) (s - b) (s - c)} = a + b + c}$ $\frac{a + b + c}{2} \in Z^{+} \Rightarrow a + b + c \in E$ $\Rightarrow {\begin{cases} a, b, c a l l a r e e v e e v e n \Rightarrow p r i m e i s 2 \\ T w o o f a, b, c a r e o d d, t h e t h i r d i s e v e n . \end{cases}$ $C - 1 : a, b, c \in E \Rightarrow a (p r i m e) = 2$ $b = 2 m, c = 2 n; m, n \in Z^{+}$ $s = (2 + 2 m + 2 n) / 2 = m + n + 1$ $s - a = 1 + m + n - 2 = m + n - 1$ $s - b = 1 + m + n - 2 m = - m + n + 1$ $s - c = 1 + m + n - 2 n = m - n + 1$ $\sqrt{s (s - a) (s - b) (s - c)} = a + b + c$ $▸ \sqrt{(m + n + 1) (m + n - 1) (- m + n + 1) (m - n + 1)}$ $= 2 m + 2 n + 2$ $▸ (m + n + 1) (m + n - 1) (- m + n + 1) (m - n + 1)$ $= 4 {(m + n + 1)}^{2}$ $▸ (m + n - 1) (- m + n + 1) (m - n + 1)$ $= 4 (m + n + 1)$ $(o, o) - c a s e : m, n \in O$ $\begin{matrix} (o, o) - c a s e : m, n \in O \end{matrix}$ $RHS i s c l e a r l y e v e n .$ $LHS : (o + o - o) (- o + o + o) (o - o + o)$ $= (e - o) (e + o) (e + o)$ $= (o) (o) (o) = o$ $LHS i s o d d .$ $C o n t r a d i c t i o n .$ $\begin{matrix} (e, e) - c a s e : m, n \in E \end{matrix}$ $RHS = e v e n$ $LHS : (e + e - o) (- e + e + o) (e - e + o)$ $(e - o) (e + o) (e + o)$ $(o) (o) (o) = o$ $C o n t r a d i c t i o n .$ $\begin{matrix} (e, o) o r (o, e) - c a s e : m \in E, n \in O \end{matrix}$ $C o n t i n u e$
	Commented by talminator2856791 last updated on 26/Sep/21

	$how did you put it in a box ?$
	Commented by Rasheed.Sindhi last updated on 26/Sep/21

	$T h e m a n u t h a t a p p e a r s o n c l i c k$ $o f m a t r i x - b u t t o n c o n t a i n s t a b l e$ $w i t h b o r d e r s . I^{'} v e d e l e t e d i t s r o w / s$ $e x c e p t o n e w h i c h I^{'} v e u s e d a s b o x .$
	Commented by talminator2856791 last updated on 05/Oct/21

	$how to delete the rows ?$
	Commented by Rasheed.Sindhi last updated on 05/Oct/21

	$W h e n y o u a r e i n a r o w (t h a t y o u w a n t$ $t o d e l e t e) o p e n s i d e m a u a n d c l i c k$ $^{'} d e l e t e {r o w}^{'} .$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum