Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 155244 by mathdanisur last updated on 27/Sep/21

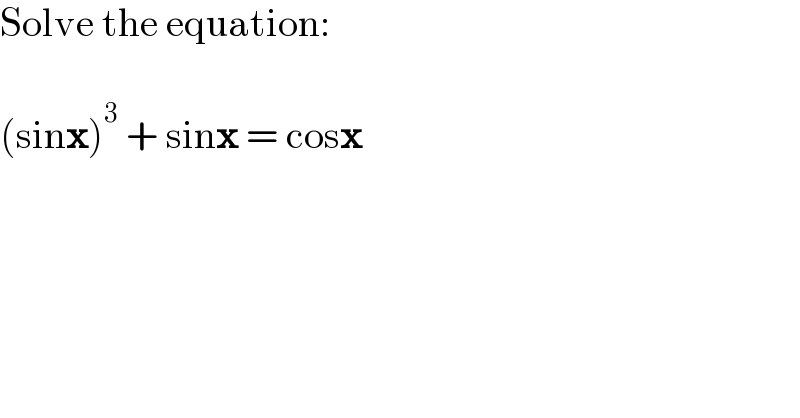
Solve the equation: (sinx)^3 + sinx = cosx

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{equation}: \\ $$$$ \\ $$$$\left(\mathrm{sin}\boldsymbol{\mathrm{x}}\right)^{\mathrm{3}} \:+\:\mathrm{sin}\boldsymbol{\mathrm{x}}\:=\:\mathrm{cos}\boldsymbol{\mathrm{x}} \\ $$

Answered by ajfour last updated on 27/Sep/21

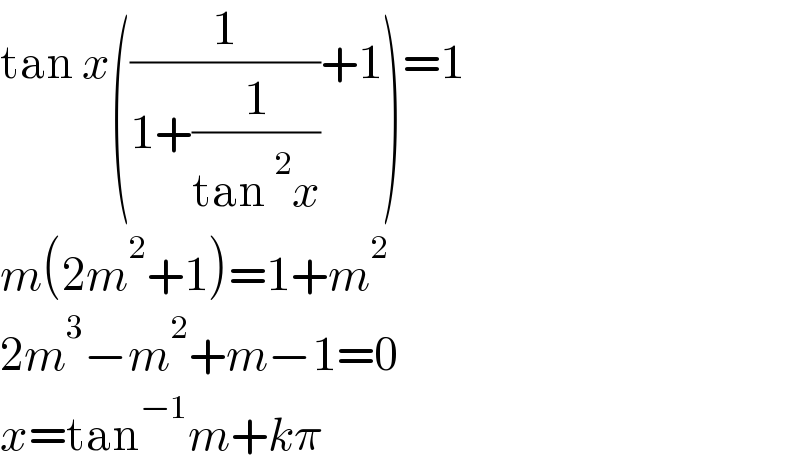
tan x((1/(1+(1/(tan^2 x))))+1)=1 m(2m^2 +1)=1+m^2 2m^3 −m^2 +m−1=0 x=tan^(−1) m+kπ

$$\mathrm{tan}\:{x}\left(\frac{\mathrm{1}}{\mathrm{1}+\frac{\mathrm{1}}{\mathrm{tan}\:^{\mathrm{2}} {x}}}+\mathrm{1}\right)=\mathrm{1} \\ $$$${m}\left(\mathrm{2}{m}^{\mathrm{2}} +\mathrm{1}\right)=\mathrm{1}+{m}^{\mathrm{2}} \\ $$$$\mathrm{2}{m}^{\mathrm{3}} −{m}^{\mathrm{2}} +{m}−\mathrm{1}=\mathrm{0} \\ $$$${x}=\mathrm{tan}^{−\mathrm{1}} {m}+{k}\pi \\ $$

Commented by mathdanisur last updated on 27/Sep/21

very nice Ser, thank you

$$\mathrm{very}\:\mathrm{nice}\:\boldsymbol{\mathrm{S}}\mathrm{er},\:\mathrm{thank}\:\mathrm{you} \\ $$

Commented by mathdanisur last updated on 27/Sep/21

Thanks Ser, but m=?

$$\mathrm{Thanks}\:\boldsymbol{\mathrm{S}}\mathrm{er},\:\mathrm{but}\:\mathrm{m}=? \\ $$

Commented by mr W last updated on 27/Sep/21

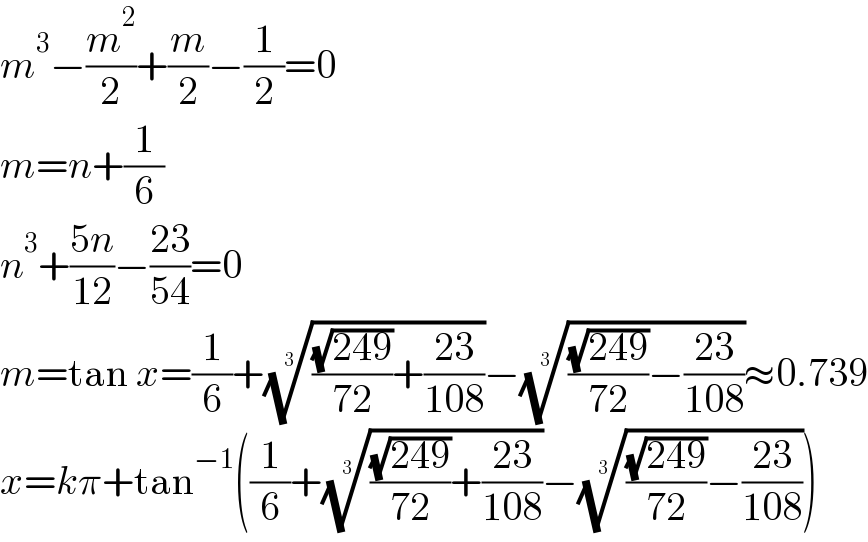
m^3 −(m^2 /2)+(m/2)−(1/2)=0 m=n+(1/6) n^3 +((5n)/(12))−((23)/(54))=0 m=tan x=(1/6)+((((√(249))/(72))+((23)/(108))))^(1/3) −((((√(249))/(72))−((23)/(108))))^(1/3) ≈0.739 x=kπ+tan^(−1) ((1/6)+((((√(249))/(72))+((23)/(108))))^(1/3) −((((√(249))/(72))−((23)/(108))))^(1/3) )

$${m}^{\mathrm{3}} −\frac{{m}^{\mathrm{2}} }{\mathrm{2}}+\frac{{m}}{\mathrm{2}}−\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{0} \\ $$$${m}={n}+\frac{\mathrm{1}}{\mathrm{6}} \\ $$$${n}^{\mathrm{3}} +\frac{\mathrm{5}{n}}{\mathrm{12}}−\frac{\mathrm{23}}{\mathrm{54}}=\mathrm{0} \\ $$$${m}=\mathrm{tan}\:{x}=\frac{\mathrm{1}}{\mathrm{6}}+\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{249}}}{\mathrm{72}}+\frac{\mathrm{23}}{\mathrm{108}}}−\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{249}}}{\mathrm{72}}−\frac{\mathrm{23}}{\mathrm{108}}}\approx\mathrm{0}.\mathrm{739} \\ $$$${x}={k}\pi+\mathrm{tan}^{−\mathrm{1}} \left(\frac{\mathrm{1}}{\mathrm{6}}+\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{249}}}{\mathrm{72}}+\frac{\mathrm{23}}{\mathrm{108}}}−\sqrt[{\mathrm{3}}]{\frac{\sqrt{\mathrm{249}}}{\mathrm{72}}−\frac{\mathrm{23}}{\mathrm{108}}}\right) \\ $$

Answered by MJS_new last updated on 27/Sep/21

sin^3 x +sin x =cos x sin^3 x +sin x =±(√(1−sin^2 x)) sin x =s s^3 +s=±(√(1+s^2 )) squaring [might introduce false solutions] and transforming s^6 +2s^4 +2s^2 −1=0 (s^2 )^3 +2(s^2 )^2 +2(s^2 )=0 s^2 =u−(2/3) u^3 +(2/3)u−((47)/(27))=0 Cardano′s solution u=((((47)/(54))+((√(249))/(18))))^(1/3) −((−((47)/(54))+((√(249))/(18))))^(1/3) it makes no sense to go on with this u≈1.01987663 ⇒ s^2 =.353209964 ⇒ sin x ≈±.594314701 testing leads to sin x ≈.594314701 ⇒ x≈2nπ+2.50517939∨x≈2nπ+.636413265

$$\mathrm{sin}^{\mathrm{3}} \:{x}\:+\mathrm{sin}\:{x}\:=\mathrm{cos}\:{x} \\ $$$$\mathrm{sin}^{\mathrm{3}} \:{x}\:+\mathrm{sin}\:{x}\:=\pm\sqrt{\mathrm{1}−\mathrm{sin}^{\mathrm{2}} \:{x}} \\ $$$$\mathrm{sin}\:{x}\:={s} \\ $$$${s}^{\mathrm{3}} +{s}=\pm\sqrt{\mathrm{1}+{s}^{\mathrm{2}} } \\ $$$$\mathrm{squaring}\:\left[\mathrm{might}\:\mathrm{introduce}\:\mathrm{false}\:\mathrm{solutions}\right] \\ $$$$\mathrm{and}\:\mathrm{transforming} \\ $$$${s}^{\mathrm{6}} +\mathrm{2}{s}^{\mathrm{4}} +\mathrm{2}{s}^{\mathrm{2}} −\mathrm{1}=\mathrm{0} \\ $$$$\left({s}^{\mathrm{2}} \right)^{\mathrm{3}} +\mathrm{2}\left({s}^{\mathrm{2}} \right)^{\mathrm{2}} +\mathrm{2}\left({s}^{\mathrm{2}} \right)=\mathrm{0} \\ $$$${s}^{\mathrm{2}} ={u}−\frac{\mathrm{2}}{\mathrm{3}} \\ $$$${u}^{\mathrm{3}} +\frac{\mathrm{2}}{\mathrm{3}}{u}−\frac{\mathrm{47}}{\mathrm{27}}=\mathrm{0} \\ $$$$\mathrm{Cardano}'\mathrm{s}\:\mathrm{solution} \\ $$$${u}=\sqrt[{\mathrm{3}}]{\frac{\mathrm{47}}{\mathrm{54}}+\frac{\sqrt{\mathrm{249}}}{\mathrm{18}}}−\sqrt[{\mathrm{3}}]{−\frac{\mathrm{47}}{\mathrm{54}}+\frac{\sqrt{\mathrm{249}}}{\mathrm{18}}} \\ $$$$\mathrm{it}\:\mathrm{makes}\:\mathrm{no}\:\mathrm{sense}\:\mathrm{to}\:\mathrm{go}\:\mathrm{on}\:\mathrm{with}\:\mathrm{this} \\ $$$${u}\approx\mathrm{1}.\mathrm{01987663} \\ $$$$\Rightarrow \\ $$$${s}^{\mathrm{2}} =.\mathrm{353209964} \\ $$$$\Rightarrow \\ $$$$\mathrm{sin}\:{x}\:\approx\pm.\mathrm{594314701} \\ $$$$\mathrm{testing}\:\mathrm{leads}\:\mathrm{to} \\ $$$$\mathrm{sin}\:{x}\:\approx.\mathrm{594314701} \\ $$$$\Rightarrow \\ $$$${x}\approx\mathrm{2}{n}\pi+\mathrm{2}.\mathrm{50517939}\vee{x}\approx\mathrm{2}{n}\pi+.\mathrm{636413265} \\ $$

Commented by mathdanisur last updated on 27/Sep/21

Very nice Ser, thank you

$$\mathrm{Very}\:\mathrm{nice}\:\boldsymbol{\mathrm{S}}\mathrm{er},\:\mathrm{thank}\:\mathrm{you} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com