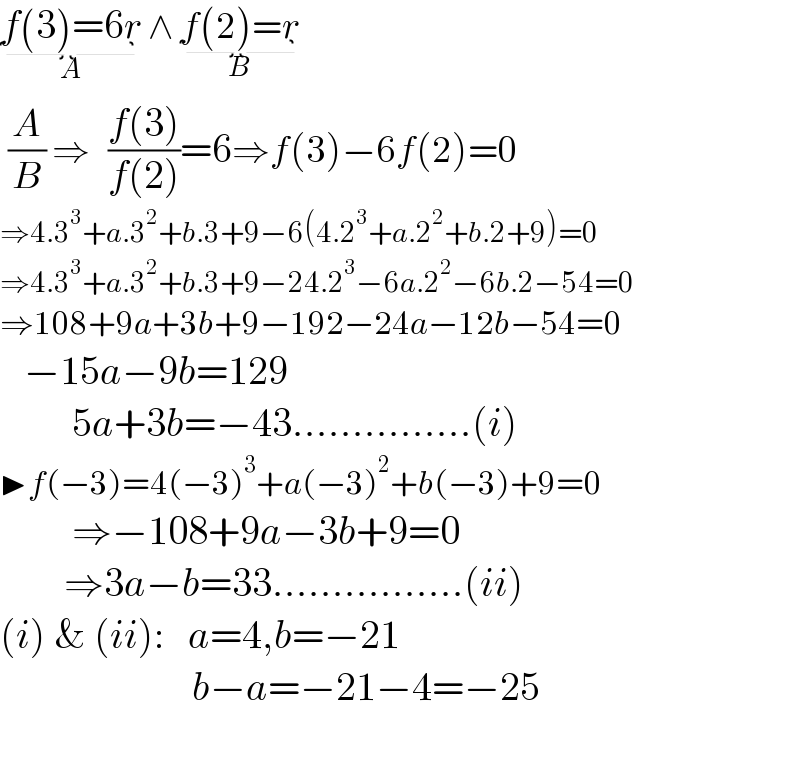Question and Answers Forum
Question Number 155576 by MathsFan last updated on 02/Oct/21
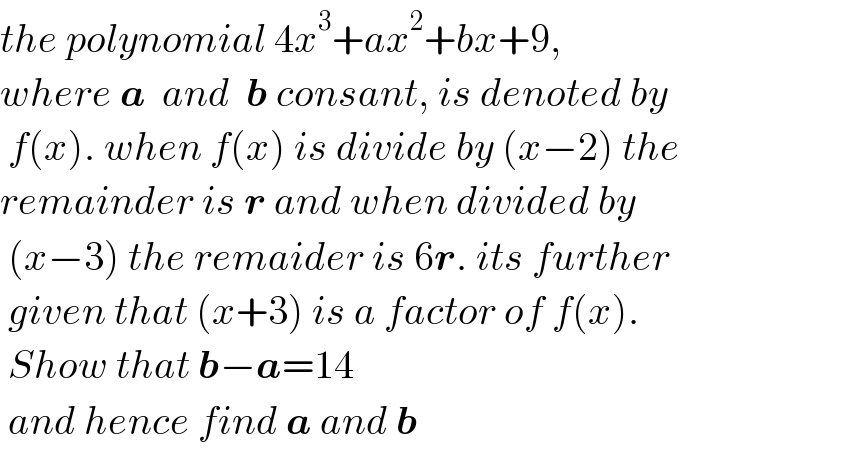
Commented by Rasheed.Sindhi last updated on 02/Oct/21
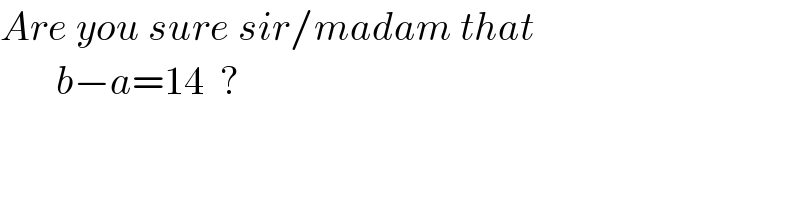
Commented by MathsFan last updated on 02/Oct/21

Commented by Rasheed.Sindhi last updated on 03/Oct/21
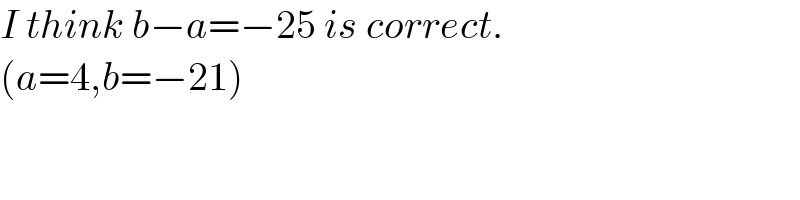
Answered by Rasheed.Sindhi last updated on 02/Oct/21
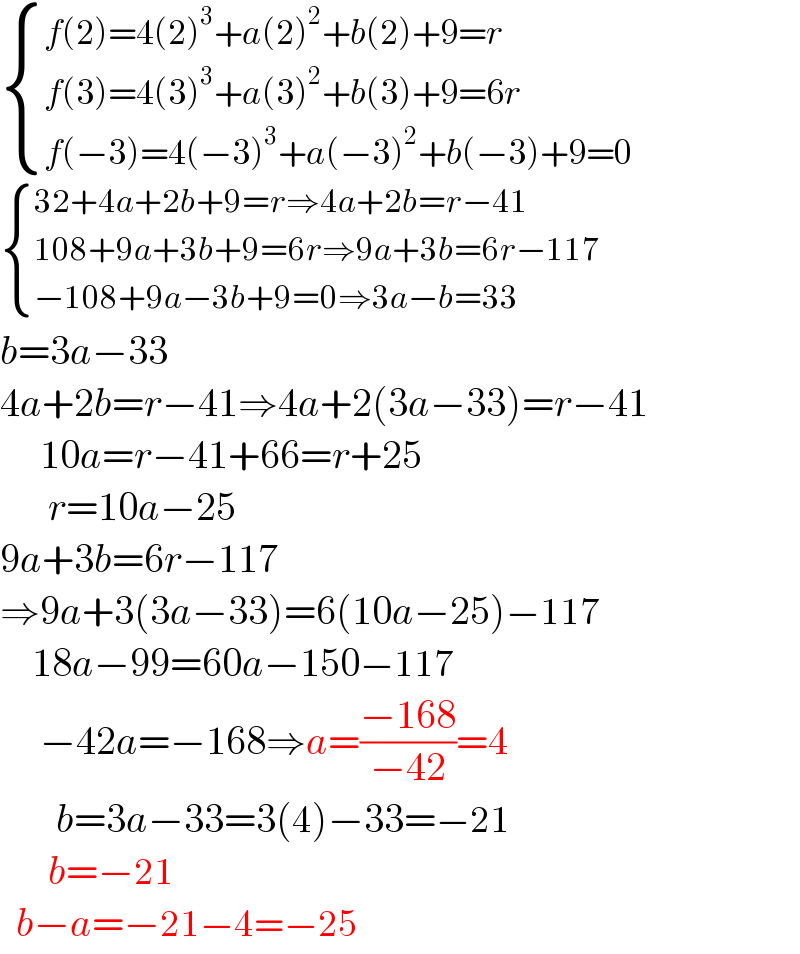
Commented by MathsFan last updated on 02/Oct/21

Answered by Rasheed.Sindhi last updated on 02/Oct/21

Commented by MathsFan last updated on 02/Oct/21
Commented by Rasheed.Sindhi last updated on 02/Oct/21
Commented by peter frank last updated on 02/Oct/21

Commented by Tawa11 last updated on 03/Oct/21

Commented by Rasheed.Sindhi last updated on 03/Oct/21
Answered by Rasheed.Sindhi last updated on 03/Oct/21

Answered by Rasheed.Sindhi last updated on 04/Oct/21

Answered by ajfour last updated on 03/Oct/21
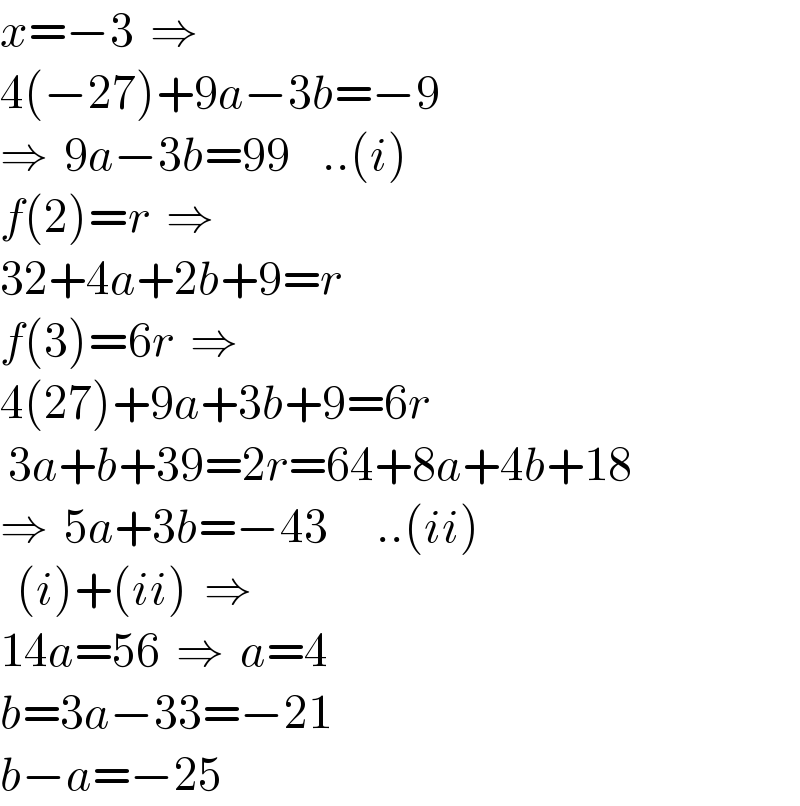
Answered by Rasheed.Sindhi last updated on 04/Oct/21
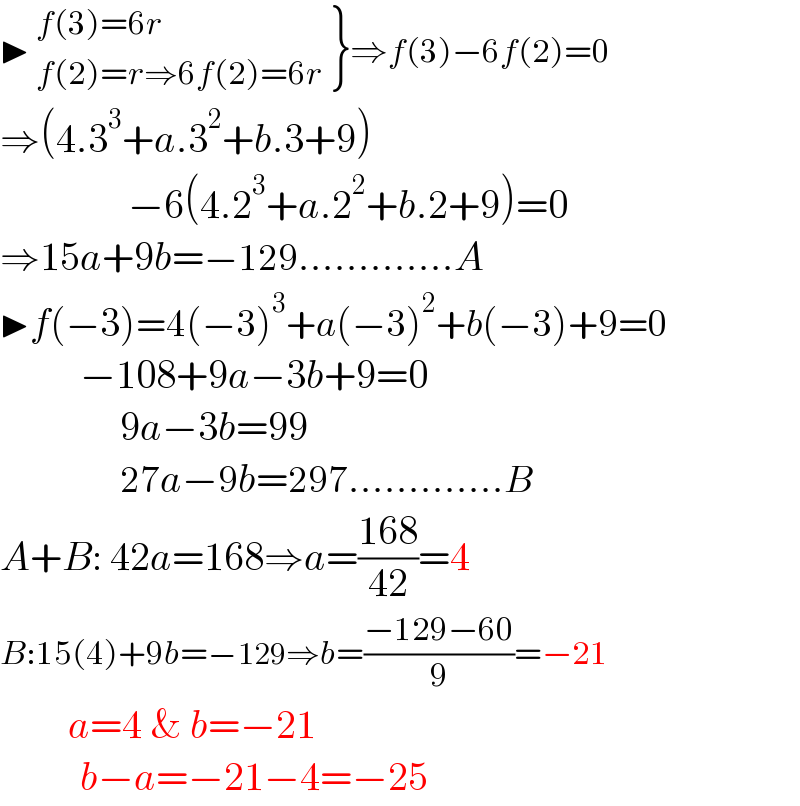
Answered by Rasheed.Sindhi last updated on 04/Oct/21