if the line px+qy=r tangents the ellipse (x^2 /a^2 )+(y^2 /b^2 )=1, then 1) prove a^2 p^2 +b^2 q^2 =r^2 2) find the coordinates of the touching point.


Question and Answers Forum

All Questions Topic List
Coordinate Geometry Questions
Previous in All Question Next in All Question
Previous in Coordinate Geometry Next in Coordinate Geometry
Question Number 157926 by mr W last updated on 29/Oct/21

$i f t h e l i n e p x + q y = r t a n g e n t s t h e$ $e l l i p s e \frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1, t h e n$ $1) p r o v e a^{2} p^{2} + b^{2} q^{2} = r^{2}$ $2) f i n d t h e c o o r d i n a t e s o f t h e$ $t o u c h i n g p o i n t .$
	Answered by mindispower last updated on 29/Oct/21
	$.... paramatric of elips{(acos(t),bsin(t))),t∈[0,2π[ M′(t)=(−asin(t),bcos(t)) M(t)∈ Line pacos(t)+qbsin(t)=r...(1) M′(t) director vector and (−q,p) line director⇒ −qbcos(t)+pasin(t)=0... (1)^2 +(2)^2 ⇔p^2 a^2 cos^2 (t)+q^2 b^2 sin^2 (t)+2paqbsin(t)cos(t) +q^2 b^2 cos^2 (t)+p^2 a^2 sin^2 (t)−2abpqsin(t)cos(t)=r^2 +0 ⇔a^2 p^2 +b^2 q^2 =r^2 { ((−qbcos(t)+pasin(t)=0....∗sin(t)..1)),((pacos(t)+qbsin(t)=r......∗cos(t)....2)) :} (1) sin(t)cos(t)≠0 ⇒(1)+(2) pa=rcos(t)⇒cos(t)=((pa)/r) M=(((a^2 p)/r),((b^2 q)/r)) sin(t)=((qb)/r) sin(t)=0⇒−qbcos(t)=0,⇒q=0 pacos(t)=r⇒cos(t)=(r/(pa))⇒∣(r/(pa))∣=1 in this case the line is x=(r/p), vertical lign M((r/p),0) cos(t)=0⇒p=0 sin(t)=(r/(qb))......The lign is y=(r/q)=^ +_− 1 vertical line M(0,(r/q))$
	$. . . . p a r a m a t r i c o f e l i p s {(a c o s (t), b s i n (t))), t \in [0, 2 π [$ $M^{'} (t) = (- a s i n (t), b c o s (t))$ $M (t) \in L i n e p a c o s (t) + q b s i n (t) = r . . . (1)$ $M^{'} (t) d i r e c t o r v e c t o r a n d (- q, p) l i n e d i r e c t o r \Rightarrow$ $- q b c o s (t) + p a s i n (t) = 0 . . .$ ${(1)}^{2} + {(2)}^{2} \Leftrightarrow p^{2} a^{2} {c o s}^{2} (t) + q^{2} b^{2} {s i n}^{2} (t) + 2 p a q b s i n (t) c o s (t)$ $+ q^{2} b^{2} {c o s}^{2} (t) + p^{2} a^{2} {s i n}^{2} (t) - 2 a b p q s i n (t) c o s (t) = r^{2} + 0$ $\Leftrightarrow a^{2} p^{2} + b^{2} q^{2} = r^{2}$ ${\begin{cases} - q b c o s (t) + p a s i n (t) = 0 . . . . * s i n (t) . . 1 \\ p a c o s (t) + q b s i n (t) = r . . . . . . * c o s (t) . . . . 2 \end{cases}$ $(1) s i n (t) c o s (t) \neq 0$ $\Rightarrow (1) + (2) p a = r c o s (t) \Rightarrow c o s (t) = \frac{p a}{r}$ $M = (\frac{a^{2} p}{r}, \frac{b^{2} q}{r})$ $s i n (t) = \frac{q b}{r}$ $s i n (t) = 0 \Rightarrow - q b c o s (t) = 0, \Rightarrow q = 0$ $p a c o s (t) = r \Rightarrow c o s (t) = \frac{r}{p a} \Rightarrow∣ \frac{r}{p a} ∣= 1$ $i n t h i s c a s e t h e l i n e i s x = \frac{r}{p}, v e r t i c a l l i g n$ $M (\frac{r}{p}, 0)$ $c o s (t) = 0 \Rightarrow p = 0$ $s i n (t) = \frac{r}{q b} . . . . . . T h e l i g n i s y = \frac{r}{q} \overset{}{=} \underline{+} 1 v e r t i c a l l i n e$ $M (0, \frac{r}{q})$
	Commented by mr W last updated on 30/Oct/21

	$t h a n k s a l o t!$
	Commented by mindispower last updated on 02/Nov/21

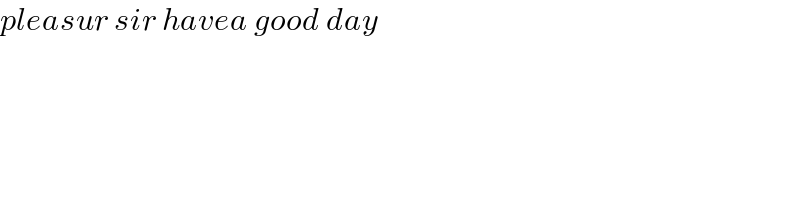
	$p l e a s u r s i r h a v e a g o o d d a y$
	Answered by som(math1967) last updated on 30/Oct/21

	$p x + q y = r \Rightarrow y = \frac{r - p x}{q}$ $\frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ $o r . \frac{x^{2}}{a^{2}} + \frac{{(r - p x)}^{2}}{b^{2} q^{2}} = 1$ $o r . x^{2} b^{2} q^{2} + a^{2} p^{2} x^{2} - 2 a^{2} p x r + r^{2} a^{2} = a^{2} b^{2} q^{2}$ $o r . (b^{2} q^{2} + a^{2} p^{2}) x^{2} - 2 a^{2} p x r + a^{2} r^{2} - a^{2} b^{2} q^{2} = 0 . . . 1)$ $p x + q y = r i s t a n g e n t s o f \frac{x^{2}}{a^{2}} + \frac{y^{2}}{b^{2}} = 1$ $∴ {(- 2 a^{2} p r)}^{2} - 4 (b^{2} q^{2} + a^{2} p^{2}) (a^{2} r^{2} - a^{2} b^{2} q^{2}) = 0$ $[f o r e q u a l r o o t s d i s c r i m i n a n t i s 0]$ $4 a^{2} [a^{2} p^{2} r^{2} - (b^{2} q^{2} + a^{2} p^{2}) (r^{2} - b^{2} q^{2})] = 0$ $a^{2} p^{2} r^{2} - b^{2} q^{2} r^{2} + b^{4} q^{4} - a^{2} p^{2} r^{2} + a^{2} b^{2} p^{2} q^{2} = 0$ $b^{2} q^{2} (b^{2} q^{2} + a^{2} p^{2} - r^{2}) = 0$ $∴ a^{2} p^{2} + b^{2} q^{2} = r^{2}$ $f r o m e q u n . 1$ $(b^{2} q^{2} + a^{2} p^{2}) x^{2} - 2 a^{2} p x r + a^{2} r^{2} - a^{2} b^{2} q^{2} = 0$ $[(a^{2} p^{2} + b^{2} q^{2} = r^{2}]$ $r^{2} x^{2} - 2 a^{2} p x r + a^{2} (r^{2} - b^{2} q^{2}) = 0$ $r^{2} x^{2} - 2 . r x . a^{2} p + a^{4} . p^{2} = 0$ $x = \frac{a^{2} p}{r}$ $y = \frac{r - p x}{q} = \frac{r - \frac{a^{2} p^{2}}{r}}{q} = \frac{r^{2} - a^{2} p^{2}}{q r}$ $(\frac{a^{2} p}{r}, \frac{r^{2} - a^{2} p^{2}}{q r})$ $(\frac{a^{2} p}{r}, \frac{b^{2} q}{r})$
	Commented by mr W last updated on 30/Oct/21

	$t h a n k s a l o t!$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum