Question and Answers Forum

All Questions      Topic List

Geometry Questions

Previous in All Question      Next in All Question      

Previous in Geometry      Next in Geometry      

Question Number 158619 by amin96 last updated on 06/Nov/21

Commented by amin96 last updated on 06/Nov/21

$$ \\ $$

Answered by Rasheed.Sindhi last updated on 07/Nov/21

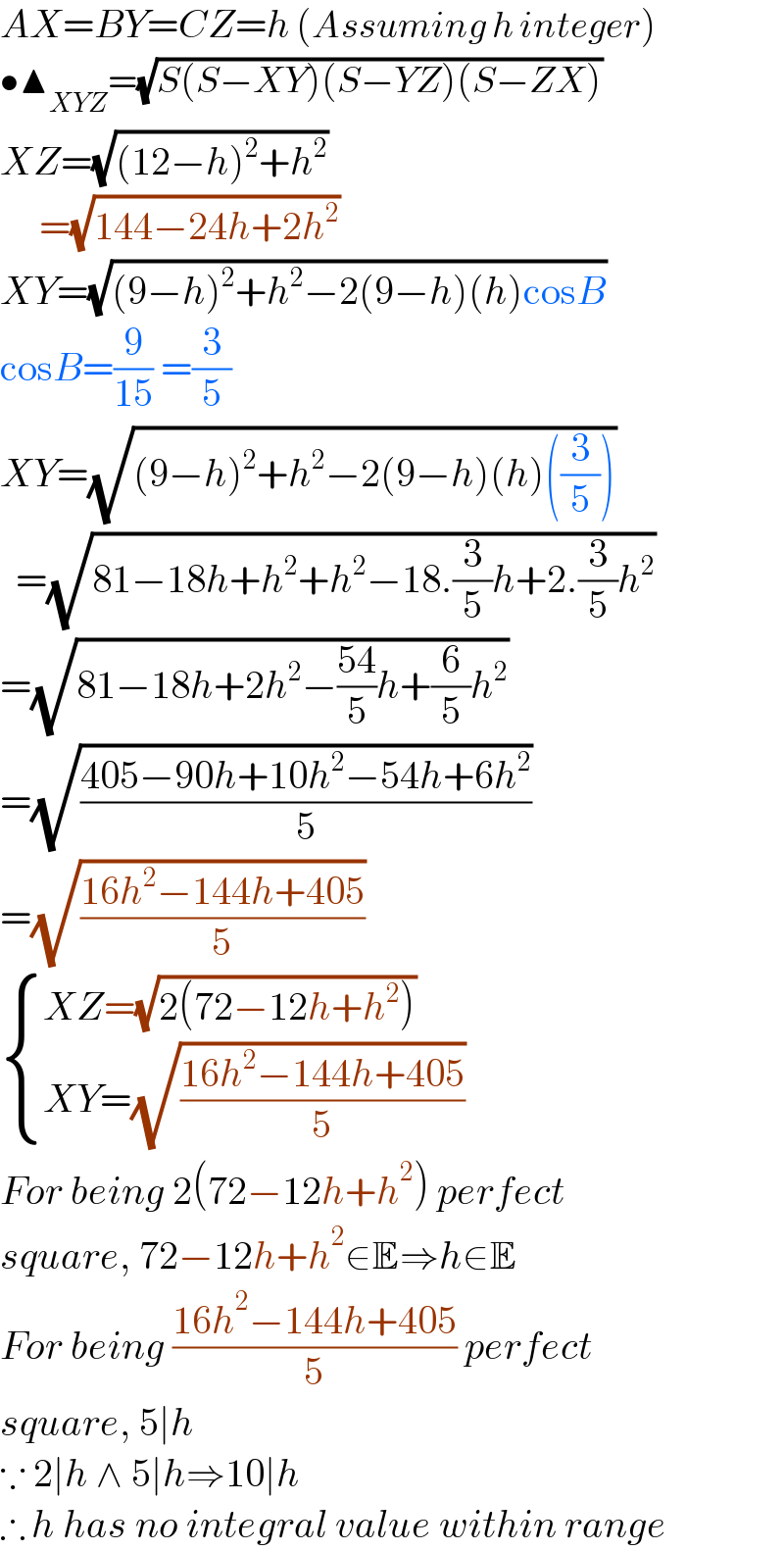
AX=BY=CZ=h (Assuming h integer) •▲_(XYZ) =(√(S(S−XY)(S−YZ)(S−ZX))) XZ=(√((12−h)^2 +h^2 )) =(√(144−24h+2h^2 )) XY=(√((9−h)^2 +h^2 −2(9−h)(h)cosB)) cosB=(9/(15)) =(3/5) XY=(√((9−h)^2 +h^2 −2(9−h)(h)((3/5)))) =(√(81−18h+h^2 +h^2 −18.(3/5)h+2.(3/5)h^2 )) =(√(81−18h+2h^2 −((54)/5)h+(6/5)h^2 )) =(√((405−90h+10h^2 −54h+6h^2 )/5)) =(√((16h^2 −144h+405)/5)) { ((XZ=(√(2(72−12h+h^2 ))))),((XY=(√((16h^2 −144h+405)/5)))) :} For being 2(72−12h+h^2 ) perfect square, 72−12h+h^2 ∈E⇒h∈E For being ((16h^2 −144h+405)/5) perfect square, 5∣h ∵ 2∣h ∧ 5∣h⇒10∣h ∴ h has no integral value within range

$${AX}={BY}={CZ}={h}\:\left({Assuming}\:{h}\:{integer}\right) \\ $$$$\bullet\blacktriangle_{{XYZ}} =\sqrt{{S}\left({S}−{XY}\right)\left({S}−{YZ}\right)\left({S}−{ZX}\right)} \\ $$$${XZ}=\sqrt{\left(\mathrm{12}−{h}\right)^{\mathrm{2}} +{h}^{\mathrm{2}} } \\ $$$$\:\:\:\:\:=\sqrt{\mathrm{144}−\mathrm{24}{h}+\mathrm{2}{h}^{\mathrm{2}} } \\ $$$${XY}=\sqrt{\left(\mathrm{9}−{h}\right)^{\mathrm{2}} +{h}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{9}−{h}\right)\left({h}\right)\mathrm{cos}{B}} \\ $$$$\mathrm{cos}{B}=\frac{\mathrm{9}}{\mathrm{15}}\:=\frac{\mathrm{3}}{\mathrm{5}} \\ $$$${XY}=\sqrt{\left(\mathrm{9}−{h}\right)^{\mathrm{2}} +{h}^{\mathrm{2}} −\mathrm{2}\left(\mathrm{9}−{h}\right)\left({h}\right)\left(\frac{\mathrm{3}}{\mathrm{5}}\right)} \\ $$$$\:\:=\sqrt{\mathrm{81}−\mathrm{18}{h}+{h}^{\mathrm{2}} +{h}^{\mathrm{2}} −\mathrm{18}.\frac{\mathrm{3}}{\mathrm{5}}{h}+\mathrm{2}.\frac{\mathrm{3}}{\mathrm{5}}{h}^{\mathrm{2}} } \\ $$$$=\sqrt{\mathrm{81}−\mathrm{18}{h}+\mathrm{2}{h}^{\mathrm{2}} −\frac{\mathrm{54}}{\mathrm{5}}{h}+\frac{\mathrm{6}}{\mathrm{5}}{h}^{\mathrm{2}} } \\ $$$$=\sqrt{\frac{\mathrm{405}−\mathrm{90}{h}+\mathrm{10}{h}^{\mathrm{2}} −\mathrm{54}{h}+\mathrm{6}{h}^{\mathrm{2}} }{\mathrm{5}}} \\ $$$$=\sqrt{\frac{\mathrm{16}{h}^{\mathrm{2}} −\mathrm{144}{h}+\mathrm{405}}{\mathrm{5}}} \\ $$$$\begin{cases}{{XZ}=\sqrt{\mathrm{2}\left(\mathrm{72}−\mathrm{12}{h}+{h}^{\mathrm{2}} \right)}}\\{{XY}=\sqrt{\frac{\mathrm{16}{h}^{\mathrm{2}} −\mathrm{144}{h}+\mathrm{405}}{\mathrm{5}}}}\end{cases} \\ $$$${For}\:{being}\:\mathrm{2}\left(\mathrm{72}−\mathrm{12}{h}+{h}^{\mathrm{2}} \right)\:{perfect} \\ $$$${square},\:\mathrm{72}−\mathrm{12}{h}+{h}^{\mathrm{2}} \in\mathbb{E}\Rightarrow{h}\in\mathbb{E} \\ $$$${For}\:{being}\:\frac{\mathrm{16}{h}^{\mathrm{2}} −\mathrm{144}{h}+\mathrm{405}}{\mathrm{5}}\:{perfect} \\ $$$${square},\:\mathrm{5}\mid{h} \\ $$$$\because\:\mathrm{2}\mid{h}\:\wedge\:\mathrm{5}\mid{h}\Rightarrow\mathrm{10}\mid{h} \\ $$$$\therefore\:{h}\:{has}\:{no}\:{integral}\:{value}\:{within}\:{range} \\ $$

Commented by amin96 last updated on 07/Nov/21

Is XYZ a right triangle?

$$ \\ $$Is XYZ a right triangle?

Commented by Rasheed.Sindhi last updated on 07/Nov/21

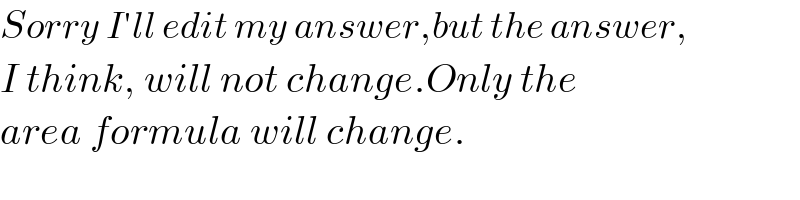
Sorry I′ll edit my answer,but the answer, I think, will not change.Only the area formula will change.

$${Sorry}\:{I}'{ll}\:{edit}\:{my}\:{answer},{but}\:{the}\:{answer}, \\ $$$${I}\:{think},\:{will}\:{not}\:{change}.{Only}\:{the} \\ $$$${area}\:{formula}\:{will}\:{change}. \\ $$

Commented by amin96 last updated on 07/Nov/21

Yes, I think so too. So there is no integer for the area of ​​the yellow triangle?

$$ \\ $$Yes, I think so too. So there is no integer for the area of ​​the yellow triangle?

Commented by mr W last updated on 07/Nov/21

only the value of the area of the yellow triangle should be integer, not the side length of the triangles!

$${only}\:{the}\:{value}\:{of}\:{the}\:{area}\:{of}\:{the}\: \\ $$$${yellow}\:{triangle}\:{should}\:{be}\:{integer},\: \\ $$$${not}\:{the}\:{side}\:{length}\:{of}\:{the}\:{triangles}!\: \\ $$

Answered by mr W last updated on 07/Nov/21

AB=p=9, AC=q=12, BC=r=15 let AX=BY=CZ=t let Δ=area of ΔABC=54 Δ_(AZX) =(t/p)×(((q−t))/q)×Δ Δ_(BXY) =(t/r)×(((p−t))/p)×Δ Δ_(CYZ) =(t/q)×(((r−t))/r)×Δ Δ_(XYZ) =[1−((t(q−t))/(pq))−((t(p−t))/(rp))−((t(r−t))/(qr))]Δ Δ_(XYZ) =(((p+q+r)t^2 −(pq+qr+rp)t+pqr)/(pqr))Δ Δ_(XYZ) =((3(4t^2 −47t+180))/(10)) Δ_(XYZ) =((3t(4t−47))/(10))+54 ((3t(4t−47))/(10)) must be an integer k. Δ_(XYZ) =k+54 12t^2 −141t=10k with k∈Z t=((141±(√(141^2 +480k)))/(24)) 141^2 +480k≥0 ⇒k≥−((141^2 )/(480)) ⇒k≥−41 such that 0<t<9 ⇒−41≤k≤−1 therefore we have following integer area for ΔXYZ: k+54 −41≤k≤−30 for each k two triangles exist. −29≤k≤−1 for each k one triangle exists. that means totally 29+2×12=43 possible yellow triangles exist. sum of all possible integer areas: 41×54+(−41−40−39−...−1) =41×54−((42×41)/2)=1335

$${AB}={p}=\mathrm{9},\:{AC}={q}=\mathrm{12},\:{BC}={r}=\mathrm{15} \\ $$$${let}\:{AX}={BY}={CZ}={t} \\ $$$${let}\:\Delta={area}\:{of}\:\Delta{ABC}=\mathrm{54} \\ $$$$\Delta_{{AZX}} =\frac{{t}}{{p}}×\frac{\left({q}−{t}\right)}{{q}}×\Delta \\ $$$$\Delta_{{BXY}} =\frac{{t}}{{r}}×\frac{\left({p}−{t}\right)}{{p}}×\Delta \\ $$$$\Delta_{{CYZ}} =\frac{{t}}{{q}}×\frac{\left({r}−{t}\right)}{{r}}×\Delta \\ $$$$\Delta_{{XYZ}} =\left[\mathrm{1}−\frac{{t}\left({q}−{t}\right)}{{pq}}−\frac{{t}\left({p}−{t}\right)}{{rp}}−\frac{{t}\left({r}−{t}\right)}{{qr}}\right]\Delta \\ $$$$\Delta_{{XYZ}} =\frac{\left({p}+{q}+{r}\right){t}^{\mathrm{2}} −\left({pq}+{qr}+{rp}\right){t}+{pqr}}{{pqr}}\Delta \\ $$$$\Delta_{{XYZ}} =\frac{\mathrm{3}\left(\mathrm{4}{t}^{\mathrm{2}} −\mathrm{47}{t}+\mathrm{180}\right)}{\mathrm{10}} \\ $$$$\Delta_{{XYZ}} =\frac{\mathrm{3}{t}\left(\mathrm{4}{t}−\mathrm{47}\right)}{\mathrm{10}}+\mathrm{54} \\ $$$$\frac{\mathrm{3}{t}\left(\mathrm{4}{t}−\mathrm{47}\right)}{\mathrm{10}}\:{must}\:{be}\:{an}\:{integer}\:{k}. \\ $$$$\Delta_{{XYZ}} ={k}+\mathrm{54} \\ $$$$\mathrm{12}{t}^{\mathrm{2}} −\mathrm{141}{t}=\mathrm{10}{k}\:{with}\:{k}\in\mathbb{Z} \\ $$$${t}=\frac{\mathrm{141}\pm\sqrt{\mathrm{141}^{\mathrm{2}} +\mathrm{480}{k}}}{\mathrm{24}} \\ $$$$\mathrm{141}^{\mathrm{2}} +\mathrm{480}{k}\geqslant\mathrm{0}\:\Rightarrow{k}\geqslant−\frac{\mathrm{141}^{\mathrm{2}} }{\mathrm{480}}\:\Rightarrow{k}\geqslant−\mathrm{41} \\ $$$${such}\:{that}\:\mathrm{0}<{t}<\mathrm{9}\:\Rightarrow−\mathrm{41}\leqslant{k}\leqslant−\mathrm{1} \\ $$$${therefore}\:{we}\:{have}\:{following} \\ $$$${integer}\:{area}\:{for}\:\Delta{XYZ}:\:{k}+\mathrm{54} \\ $$$$−\mathrm{41}\leqslant{k}\leqslant−\mathrm{30}\:{for}\:{each}\:{k}\:{two}\:{triangles}\:{exist}. \\ $$$$−\mathrm{29}\leqslant{k}\leqslant−\mathrm{1}\:{for}\:{each}\:{k}\:{one}\:{triangle}\:{exists}. \\ $$$${that}\:{means}\:{totally}\:\mathrm{29}+\mathrm{2}×\mathrm{12}=\mathrm{43}\: \\ $$$${possible}\:{yellow}\:{triangles}\:{exist}. \\ $$$${sum}\:{of}\:{all}\:{possible}\:{integer}\:{areas}: \\ $$$$\mathrm{41}×\mathrm{54}+\left(−\mathrm{41}−\mathrm{40}−\mathrm{39}−...−\mathrm{1}\right) \\ $$$$=\mathrm{41}×\mathrm{54}−\frac{\mathrm{42}×\mathrm{41}}{\mathrm{2}}=\mathrm{1335} \\ $$

Commented by mr W last updated on 07/Nov/21

an other way: max. yellow triangle should be smaller than ΔABC=54 min. yellow triangle (see Q158664) =(1−(((9×12+12×15+15×9)^2 )/(4×9×12×15×(9+12+15))))×54 ≈12.6 that means the integer value for the area of the yellow triangle can be from 13 to 53. sum of these values =(((13+53)×41)/2)=1353

$${an}\:{other}\:{way}: \\ $$$${max}.\:{yellow}\:{triangle}\:{should}\:{be}\: \\ $$$${smaller}\:{than}\:\Delta{ABC}=\mathrm{54} \\ $$$${min}.\:{yellow}\:{triangle}\:\left({see}\:{Q}\mathrm{158664}\right) \\ $$$$=\left(\mathrm{1}−\frac{\left(\mathrm{9}×\mathrm{12}+\mathrm{12}×\mathrm{15}+\mathrm{15}×\mathrm{9}\right)^{\mathrm{2}} }{\mathrm{4}×\mathrm{9}×\mathrm{12}×\mathrm{15}×\left(\mathrm{9}+\mathrm{12}+\mathrm{15}\right)}\right)×\mathrm{54} \\ $$$$\approx\mathrm{12}.\mathrm{6} \\ $$$${that}\:{means}\:{the}\:{integer}\:{value}\:{for} \\ $$$${the}\:{area}\:{of}\:{the}\:{yellow}\:{triangle}\:{can} \\ $$$${be}\:{from}\:\mathrm{13}\:{to}\:\mathrm{53}. \\ $$$${sum}\:{of}\:{these}\:{values}\: \\ $$$$=\frac{\left(\mathrm{13}+\mathrm{53}\right)×\mathrm{41}}{\mathrm{2}}=\mathrm{1353} \\ $$

Commented by Tawa11 last updated on 07/Nov/21

Great sir

$$\mathrm{Great}\:\mathrm{sir} \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com