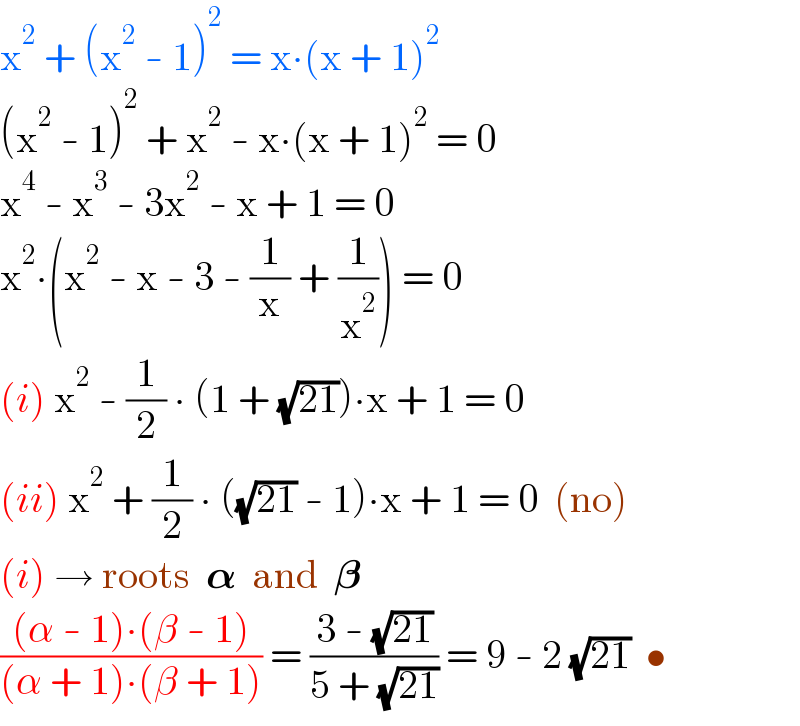Math Question and Answers


Question and Answers Forum

All Questions Topic List
Differentiation Questions
Previous in All Question Next in All Question
Previous in Differentiation Next in Differentiation
Question Number 160837 by mnjuly1970 last updated on 07/Dec/21

Commented by cortano last updated on 07/Dec/21
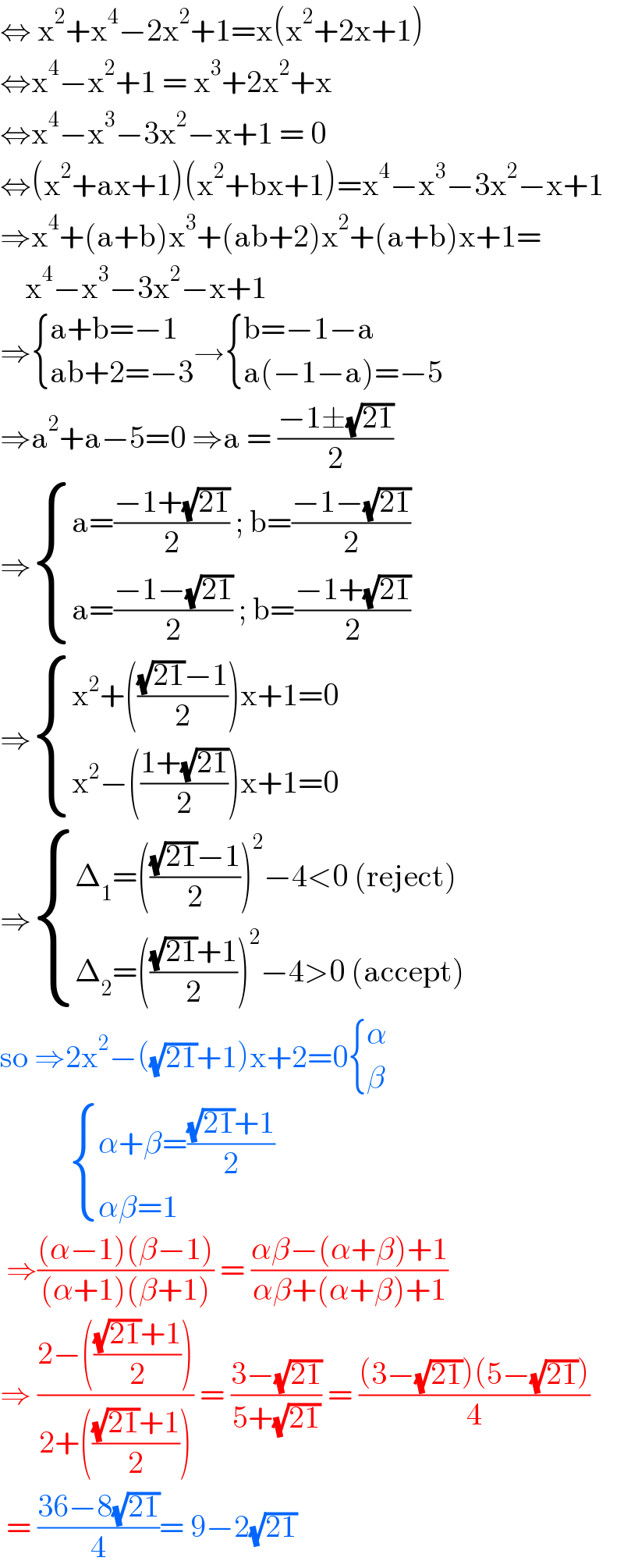
$⇔ x^2 +x^4 −2x^2 +1=x(x^2 +2x+1) ⇔x^4 −x^2 +1 = x^3 +2x^2 +x ⇔x^4 −x^3 −3x^2 −x+1 = 0 ⇔(x^2 +ax+1)(x^2 +bx+1)=x^4 −x^3 −3x^2 −x+1 ⇒x^4 +(a+b)x^3 +(ab+2)x^2 +(a+b)x+1= x^4 −x^3 −3x^2 −x+1 ⇒ { ((a+b=−1)),((ab+2=−3)) :}→ { ((b=−1−a)),((a(−1−a)=−5)) :} ⇒a^2 +a−5=0 ⇒a = ((−1±(√(21)))/2) ⇒ { ((a=((−1+(√(21)))/2) ; b=((−1−(√(21)))/2))),((a=((−1−(√(21)))/2) ; b=((−1+(√(21)))/2))) :} ⇒ { ((x^2 +((((√(21))−1)/2))x+1=0)),((x^2 −(((1+(√(21)))/2))x+1=0)) :} ⇒ { ((Δ_1 =((((√(21))−1)/2))^2 −4<0 (reject))),((Δ_2 =((((√(21))+1)/2))^2 −4>0 (accept))) :} so ⇒2x^2 −((√(21))+1)x+2=0 { (α),(β) :} { ((α+β=(((√(21))+1)/2))),((αβ=1)) :} ⇒(((α−1)(β−1))/((α+1)(β+1))) = ((αβ−(α+β)+1)/(αβ+(α+β)+1)) ⇒ ((2−((((√(21))+1)/2)))/(2+((((√(21))+1)/2)))) = ((3−(√(21)))/(5+(√(21)))) = (((3−(√(21)))(5−(√(21))))/4) = ((36−8(√(21)))/4)= 9−2(√(21))$
$\Leftrightarrow x^{2} + x^{4} - {2 x}^{2} + 1 = x (x^{2} + 2 x + 1)$ $\Leftrightarrow x^{4} - x^{2} + 1 = x^{3} + {2 x}^{2} + x$ $\Leftrightarrow x^{4} - x^{3} - {3 x}^{2} - x + 1 = 0$ $\Leftrightarrow (x^{2} + ax + 1) (x^{2} + bx + 1) = x^{4} - x^{3} - {3 x}^{2} - x + 1$ $\Rightarrow x^{4} + (a + b) x^{3} + (ab + 2) x^{2} + (a + b) x + 1 =$ $x^{4} - x^{3} - {3 x}^{2} - x + 1$ $\Rightarrow {\begin{cases} a + b = - 1 \\ ab + 2 = - 3 \end{cases} \to {\begin{cases} b = - 1 - a \\ a (- 1 - a) = - 5 \end{cases}$ $\Rightarrow a^{2} + a - 5 = 0 \Rightarrow a = \frac{- 1 \pm \sqrt{21}}{2}$ $\Rightarrow {\begin{cases} a = \frac{- 1 + \sqrt{21}}{2}; b = \frac{- 1 - \sqrt{21}}{2} \\ a = \frac{- 1 - \sqrt{21}}{2}; b = \frac{- 1 + \sqrt{21}}{2} \end{cases}$ $\Rightarrow {\begin{cases} x^{2} + (\frac{\sqrt{21} - 1}{2}) x + 1 = 0 \\ x^{2} - (\frac{1 + \sqrt{21}}{2}) x + 1 = 0 \end{cases}$ $\Rightarrow {\begin{cases} Δ_{1} = {(\frac{\sqrt{21} - 1}{2})}^{2} - 4 < 0 (reject) \\ Δ_{2} = {(\frac{\sqrt{21} + 1}{2})}^{2} - 4 > 0 (accept) \end{cases}$ $so \Rightarrow {2 x}^{2} - (\sqrt{21} + 1) x + 2 = 0 {\begin{cases} α \\ β \end{cases}$ ${\begin{cases} α + β = \frac{\sqrt{21} + 1}{2} \\ α β = 1 \end{cases}$ $\Rightarrow \frac{(α - 1) (β - 1)}{(α + 1) (β + 1)} = \frac{α β - (α + β) + 1}{α β + (α + β) + 1}$ $\Rightarrow \frac{2 - (\frac{\sqrt{21} + 1}{2})}{2 + (\frac{\sqrt{21} + 1}{2})} = \frac{3 - \sqrt{21}}{5 + \sqrt{21}} = \frac{(3 - \sqrt{21}) (5 - \sqrt{21})}{4}$ $= \frac{36 - 8 \sqrt{21}}{4} = 9 - 2 \sqrt{21}$
Commented by mnjuly1970 last updated on 07/Dec/21

$t h a n k s a l o t m a s t e r . . .$
Commented by Tawa11 last updated on 07/Dec/21

$Great sir .$
Commented by HongKing last updated on 08/Dec/21

$x^{2} + {(x^{2} - 1)}^{2} = x \cdot {(x + 1)}^{2}$ ${(x^{2} - 1)}^{2} + x^{2} - x \cdot {(x + 1)}^{2} = 0$ $x^{4} - x^{3} - {3 x}^{2} - x + 1 = 0$ $x^{2} \cdot (x^{2} - x - 3 - \frac{1}{x} + \frac{1}{x^{2}}) = 0$ $(i) x^{2} - \frac{1}{2} \cdot (1 + \sqrt{21}) \cdot x + 1 = 0$ $(i i) x^{2} + \frac{1}{2} \cdot (\sqrt{21} - 1) \cdot x + 1 = 0 (no)$ $(i) \to roots α and β$ $\frac{(α - 1) \cdot (β - 1)}{(α + 1) \cdot (β + 1)} = \frac{3 - \sqrt{21}}{5 + \sqrt{21}} = 9 - 2 \sqrt{21} ∙$
Terms of Service
Privacy Policy
Contact: info@tinkutara.com

Question and Answers Forum