
Question Number 161504 by mathocean1 last updated on 18/Dec/21
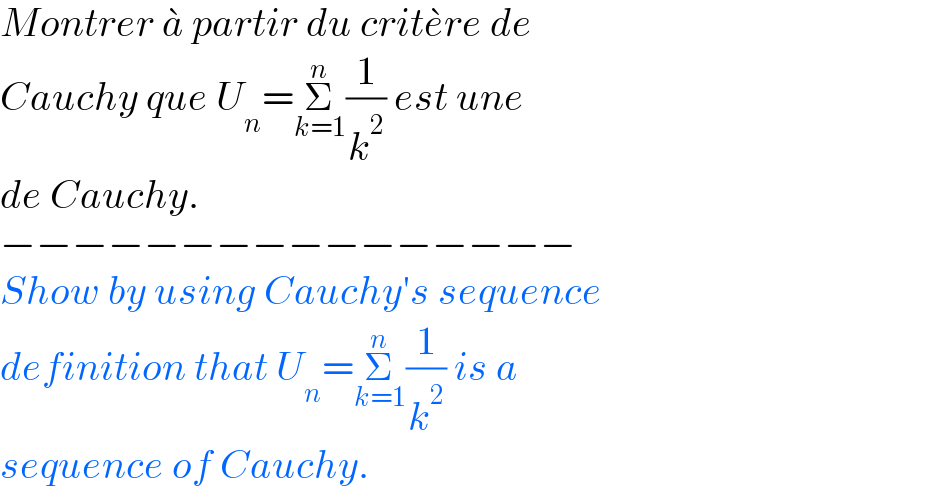
$${Montrer}\:\grave {{a}}\:{partir}\:{du}\:{crit}\grave {{e}re}\:{de}\: \\ $$$${Cauchy}\:{que}\:{U}_{{n}} =\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{k}^{\mathrm{2}} }\:{est}\:{une} \\ $$$${de}\:{Cauchy}. \\ $$$$−−−−−−−−−−−−−−−− \\ $$$${Show}\:{by}\:{using}\:{Cauchy}'{s}\:{sequence} \\ $$$${definition}\:{that}\:{U}_{{n}} =\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{k}^{\mathrm{2}} }\:{is}\:{a}\: \\ $$$${sequence}\:{of}\:{Cauchy}. \\ $$
Answered by mindispower last updated on 20/Dec/21
![⇔∀ε>0 ∃N ∀(n,m)≥N ∣U_n −U_m ∣<ε soit ε>0 U_n −U_m =Σ_(k=m+1) ^n (1/k^2 )=S_(n,m) f(x)=(1/x^2 ) (1/((k+1)^2 ))≤∫_k ^(k+1) (1/x^2 )dx≤(1/k^2 ) ⇒Σ_(k=m) ^(n−1) (1/((k+1)^2 ))<(1/k)−(1/(k+1)) S_(n,m) <(1/m)−(1/n)=((n−m)/(nm)) soit N∈N tell? Que (1/N)<ε ⇒N=[ε]+1 ⇒S_(n,m) =(1/m)−(1/n)<(1/N)<ε,∀(n,m)>N ⇒U_n est de cauchy](Q161616.png)
$$\Leftrightarrow\forall\epsilon>\mathrm{0}\:\exists{N}\:\forall\left({n},{m}\right)\geqslant{N}\:\mid{U}_{{n}} −{U}_{{m}} \mid<\epsilon \\ $$$${soit}\:\epsilon>\mathrm{0} \\ $$$${U}_{{n}} −{U}_{{m}} =\underset{{k}={m}+\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}}{{k}^{\mathrm{2}} }={S}_{{n},{m}} \\ $$$${f}\left({x}\right)=\frac{\mathrm{1}}{{x}^{\mathrm{2}} } \\ $$$$\frac{\mathrm{1}}{\left({k}+\mathrm{1}\right)^{\mathrm{2}} }\leqslant\int_{{k}} ^{{k}+\mathrm{1}} \frac{\mathrm{1}}{{x}^{\mathrm{2}} }{dx}\leqslant\frac{\mathrm{1}}{{k}^{\mathrm{2}} } \\ $$$$\Rightarrow\underset{{k}={m}} {\overset{{n}−\mathrm{1}} {\sum}}\frac{\mathrm{1}}{\left({k}+\mathrm{1}\right)^{\mathrm{2}} }<\frac{\mathrm{1}}{{k}}−\frac{\mathrm{1}}{{k}+\mathrm{1}} \\ $$$${S}_{{n},{m}} <\frac{\mathrm{1}}{{m}}−\frac{\mathrm{1}}{{n}}=\frac{{n}−{m}}{{nm}} \\ $$$${soit}\:{N}\in\mathbb{N}\:{tell}?\:{Que}\:\frac{\mathrm{1}}{{N}}<\epsilon\:\Rightarrow{N}=\left[\epsilon\right]+\mathrm{1} \\ $$$$\Rightarrow{S}_{{n},{m}} =\frac{\mathrm{1}}{{m}}−\frac{\mathrm{1}}{{n}}<\frac{\mathrm{1}}{{N}}<\epsilon,\forall\left({n},{m}\right)>{N} \\ $$$$\Rightarrow{U}_{{n}} {est}\:{de}\:{cauchy} \\ $$$$ \\ $$$$ \\ $$$$ \\ $$
Commented by mathocean1 last updated on 21/Dec/21

$${Thanks}\:{sir}! \\ $$
