Question and Answers Forum

All Questions      Topic List

Algebra Questions

Previous in All Question      Next in All Question      

Previous in Algebra      Next in Algebra      

Question Number 161861 by Rasheed.Sindhi last updated on 23/Dec/21

Prove that ((1^2 ∙2!+2^2 ∙3!+3^2 ∙4!+∙∙∙+n^2 (n+1)!−2)/((n+1)!)) =n^2 +n−2

$$\mathrm{Prove}\:\mathrm{that} \\ $$$$\:\:\:\frac{\mathrm{1}^{\mathrm{2}} \centerdot\mathrm{2}!+\mathrm{2}^{\mathrm{2}} \centerdot\mathrm{3}!+\mathrm{3}^{\mathrm{2}} \centerdot\mathrm{4}!+\centerdot\centerdot\centerdot+{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)!−\mathrm{2}}{\left({n}+\mathrm{1}\right)!} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:={n}^{\mathrm{2}} +{n}−\mathrm{2} \\ $$

Commented by mr W last updated on 23/Dec/21

after some try & try i got it!

$${after}\:{some}\:{try}\:\&\:{try}\:{i}\:{got}\:{it}! \\ $$

Commented by Rasheed.Sindhi last updated on 24/Dec/21

Sir I′ve not got the result by simplification. Actually I made sequence f(1),f(2),f(3),... and my son (Faaiz Soomro) helped me to get general formula for f(n): f(n)=((1^2 ∙2!+2^2 ∙3!+3^2 ∙4!+∙∙∙+n^2 (n+1)!−2)/((n+1)!)) (say) determinant ((n,(f(n))),(1,0),(2,4),(3,(10)),(4,(18)),(5,(28)),(6,(40)),((∙∙∙),(∙∙∙)),(n,(n^2 +n−2)))

$$\mathbb{S}\mathrm{ir}\:\mathrm{I}'\mathrm{ve}\:\mathrm{not}\:\mathrm{got}\:\mathrm{the}\:\mathrm{result}\:\mathrm{by}\:\mathrm{simplification}. \\ $$$$\mathrm{Actually}\:\mathrm{I}\:\mathrm{made}\:\mathrm{sequence}\: \\ $$$$\mathrm{f}\left(\mathrm{1}\right),\mathrm{f}\left(\mathrm{2}\right),\mathrm{f}\left(\mathrm{3}\right),... \\ $$$$\mathrm{and}\:\mathrm{my}\:\mathrm{son}\:\left(\mathrm{Faaiz}\:\mathrm{Soomro}\right)\:\mathrm{helped} \\ $$$$\mathrm{me}\:\mathrm{to}\:\mathrm{get}\:\mathrm{general}\:\mathrm{formula}\:\mathrm{for}\:\mathrm{f}\left(\mathrm{n}\right): \\ $$$${f}\left({n}\right)=\frac{\mathrm{1}^{\mathrm{2}} \centerdot\mathrm{2}!+\mathrm{2}^{\mathrm{2}} \centerdot\mathrm{3}!+\mathrm{3}^{\mathrm{2}} \centerdot\mathrm{4}!+\centerdot\centerdot\centerdot+{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)!−\mathrm{2}}{\left({n}+\mathrm{1}\right)!}\:\left({say}\right) \\ $$$$\begin{array}{|c|c|c|c|c|c|c|c|c|}{{n}}&\hline{{f}\left({n}\right)}\\{\mathrm{1}}&\hline{\mathrm{0}}\\{\mathrm{2}}&\hline{\mathrm{4}}\\{\mathrm{3}}&\hline{\mathrm{10}}\\{\mathrm{4}}&\hline{\mathrm{18}}\\{\mathrm{5}}&\hline{\mathrm{28}}\\{\mathrm{6}}&\hline{\mathrm{40}}\\{\centerdot\centerdot\centerdot}&\hline{\centerdot\centerdot\centerdot}\\{{n}}&\hline{{n}^{\mathrm{2}} +{n}−\mathrm{2}}\\\hline\end{array} \\ $$

Commented by Rasheed.Sindhi last updated on 24/Dec/21

Related Question:Q#161800

$$\mathcal{R}{elated}\:\mathcal{Q}{uestion}:\mathcal{Q}#\mathrm{161800} \\ $$

Commented by mr W last updated on 24/Dec/21

i was wondering how you got that result. to be honest, to prove the result is not a big problem, there are many methods. but i′m basically interested how Σ_(k=1) ^n k^2 (k+1)! can be simplified at all, i.e. i mainly don′t want just to prove the result, but how to obtain the result.

$${i}\:{was}\:{wondering}\:{how}\:{you}\:{got}\:{that} \\ $$$${result}.\:{to}\:{be}\:{honest},\:{to}\:{prove}\:{the} \\ $$$${result}\:{is}\:{not}\:{a}\:{big}\:{problem},\:{there}\:{are} \\ $$$${many}\:{methods}.\:{but}\:{i}'{m}\:{basically} \\ $$$${interested}\:{how}\:\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \left({k}+\mathrm{1}\right)!\:{can}\:{be} \\ $$$${simplified}\:{at}\:{all},\:{i}.{e}.\:{i}\:{mainly}\:{don}'{t}\: \\ $$$${want}\:{just}\:{to}\:{prove}\:{the}\:{result},\:{but}\:{how} \\ $$$${to}\:{obtain}\:{the}\:{result}. \\ $$

Commented by mr W last updated on 24/Dec/21

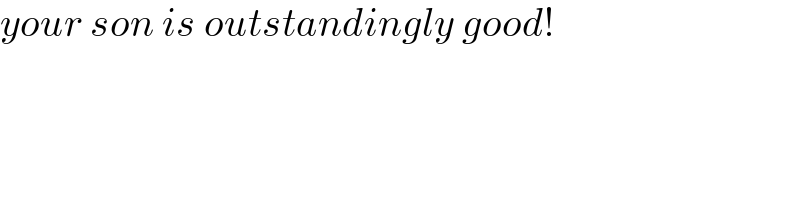
your son is outstandingly good!

$${your}\:{son}\:{is}\:{outstandingly}\:{good}! \\ $$

Commented by Rasheed.Sindhi last updated on 24/Dec/21

I also changed the problem in the following system: { ((a_0 =−2 )),((a_n =a_(n−1) +2n)) :} but have not yet solved.It′s solution will be certainly a_n =n^2 +n−2

$$\mathrm{I}\:\mathrm{also}\:\mathrm{changed}\:\mathrm{the}\:\mathrm{problem}\:\mathrm{in}\:\mathrm{the} \\ $$$$\mathrm{following}\:\mathrm{system}: \\ $$$$\begin{cases}{{a}_{\mathrm{0}} =−\mathrm{2}\:}\\{{a}_{{n}} ={a}_{{n}−\mathrm{1}} +\mathrm{2}{n}}\end{cases} \\ $$$${but}\:{have}\:{not}\:{yet}\:{solved}.{It}'{s}\:{solution} \\ $$$${will}\:{be}\:{certainly}\: \\ $$$$\:\:\:\:\:{a}_{{n}} ={n}^{\mathrm{2}} +{n}−\mathrm{2} \\ $$

Commented by mr W last updated on 24/Dec/21

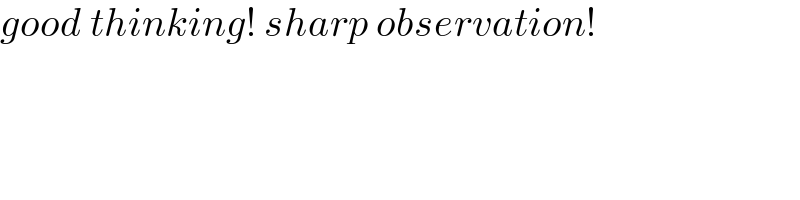
good thinking! sharp observation!

$${good}\:{thinking}!\:{sharp}\:{observation}! \\ $$

Commented by Rasheed.Sindhi last updated on 24/Dec/21

Grateful Sir!

$$\mathbb{G}\mathrm{rateful}\:\mathbb{S}\mathrm{ir}! \\ $$

Answered by mr W last updated on 23/Dec/21

k^2 (k+1)! =(k+1−1)k(k+1)! =(k+1)k(k+1)!−k(k−1)k!−2kk! =(k+1)k(k+1)!−k(k−1)k!−2[(k+1)!−k!] Σ_(k=1) ^n k^2 (k+1)! =Σ_(k=1) ^n (k+1)k(k+1)!−Σ_(k=1) ^n k(k−1)k!−2[Σ_(k=1) ^n (k+1)!−Σ_(k=1) ^n k!] =Σ_(k=2) ^(n+1) k(k−1)k!−Σ_(k=1) ^n k(k−1)k!−2[Σ_(k=2) ^(n+1) k!−Σ_(k=1) ^n k!] =(n+1)n(n+1)!−2[(n+1)!−1!] =(n^2 +n−2)(n+1)!+2 Σ_(k=1) ^n k^2 (k+1)!−2=(n^2 +n−2)(n+1)! ((Σ_(k=1) ^n k^2 (k+1)!−2)/((n+1)!))=n^2 +n−2=(n−1)(n+2) ✓

$${k}^{\mathrm{2}} \left({k}+\mathrm{1}\right)! \\ $$$$=\left({k}+\mathrm{1}−\mathrm{1}\right){k}\left({k}+\mathrm{1}\right)! \\ $$$$=\left({k}+\mathrm{1}\right){k}\left({k}+\mathrm{1}\right)!−{k}\left({k}−\mathrm{1}\right){k}!−\mathrm{2}{kk}! \\ $$$$=\left({k}+\mathrm{1}\right){k}\left({k}+\mathrm{1}\right)!−{k}\left({k}−\mathrm{1}\right){k}!−\mathrm{2}\left[\left({k}+\mathrm{1}\right)!−{k}!\right] \\ $$$$ \\ $$$$\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \left({k}+\mathrm{1}\right)! \\ $$$$=\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\left({k}+\mathrm{1}\right){k}\left({k}+\mathrm{1}\right)!−\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}\left({k}−\mathrm{1}\right){k}!−\mathrm{2}\left[\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\left({k}+\mathrm{1}\right)!−\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}!\right] \\ $$$$=\underset{{k}=\mathrm{2}} {\overset{{n}+\mathrm{1}} {\sum}}{k}\left({k}−\mathrm{1}\right){k}!−\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}\left({k}−\mathrm{1}\right){k}!−\mathrm{2}\left[\underset{{k}=\mathrm{2}} {\overset{{n}+\mathrm{1}} {\sum}}{k}!−\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}!\right] \\ $$$$=\left({n}+\mathrm{1}\right){n}\left({n}+\mathrm{1}\right)!−\mathrm{2}\left[\left({n}+\mathrm{1}\right)!−\mathrm{1}!\right] \\ $$$$=\left({n}^{\mathrm{2}} +{n}−\mathrm{2}\right)\left({n}+\mathrm{1}\right)!+\mathrm{2} \\ $$$$ \\ $$$$\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \left({k}+\mathrm{1}\right)!−\mathrm{2}=\left({n}^{\mathrm{2}} +{n}−\mathrm{2}\right)\left({n}+\mathrm{1}\right)! \\ $$$$\frac{\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}{k}^{\mathrm{2}} \left({k}+\mathrm{1}\right)!−\mathrm{2}}{\left({n}+\mathrm{1}\right)!}={n}^{\mathrm{2}} +{n}−\mathrm{2}=\left({n}−\mathrm{1}\right)\left({n}+\mathrm{2}\right)\:\checkmark \\ $$

Commented by mr W last updated on 23/Dec/21

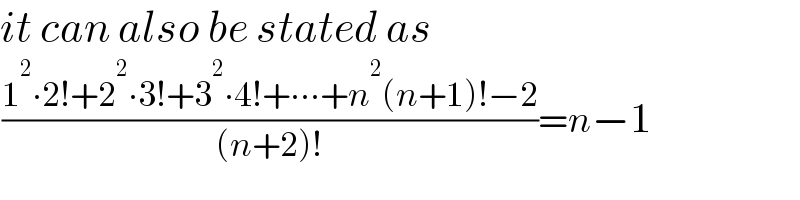
it can also be stated as ((1^2 ∙2!+2^2 ∙3!+3^2 ∙4!+∙∙∙+n^2 (n+1)!−2)/((n+2)!))=n−1

$${it}\:{can}\:{also}\:{be}\:{stated}\:{as} \\ $$$$\:\frac{\mathrm{1}^{\mathrm{2}} \centerdot\mathrm{2}!+\mathrm{2}^{\mathrm{2}} \centerdot\mathrm{3}!+\mathrm{3}^{\mathrm{2}} \centerdot\mathrm{4}!+\centerdot\centerdot\centerdot+{n}^{\mathrm{2}} \left({n}+\mathrm{1}\right)!−\mathrm{2}}{\left({n}+\mathrm{2}\right)!}={n}−\mathrm{1} \\ $$

Commented by Rasheed.Sindhi last updated on 24/Dec/21

ThanX a Lot sir!

$$\mathcal{T}{han}\mathcal{X}\:{a}\:\mathcal{L}{ot}\:\boldsymbol{{sir}}! \\ $$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com