
Question Number 162253 by ZiYangLee last updated on 28/Dec/21
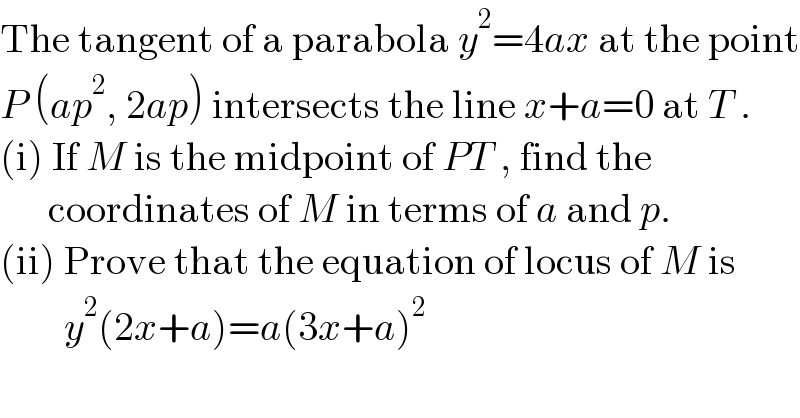
$$\mathrm{The}\:\mathrm{tangent}\:\mathrm{of}\:\mathrm{a}\:\mathrm{parabola}\:{y}^{\mathrm{2}} =\mathrm{4}{ax}\:\mathrm{at}\:\mathrm{the}\:\mathrm{point} \\ $$$${P}\:\left({ap}^{\mathrm{2}} ,\:\mathrm{2}{ap}\right)\:\mathrm{intersects}\:\mathrm{the}\:\mathrm{line}\:{x}+{a}=\mathrm{0}\:\mathrm{at}\:{T}\:. \\ $$$$\left(\mathrm{i}\right)\:\mathrm{If}\:{M}\:\mathrm{is}\:\mathrm{the}\:\mathrm{midpoint}\:\mathrm{of}\:{PT}\:,\:\mathrm{find}\:\mathrm{the}\: \\ $$$$\:\:\:\:\:\:\mathrm{coordinates}\:\mathrm{of}\:{M}\:\mathrm{in}\:\mathrm{terms}\:\mathrm{of}\:{a}\:\mathrm{and}\:{p}. \\ $$$$\left(\mathrm{ii}\right)\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{equation}\:\mathrm{of}\:\mathrm{locus}\:\mathrm{of}\:{M}\:\mathrm{is} \\ $$$$\:\:\:\:\:\:\:\:{y}^{\mathrm{2}} \left(\mathrm{2}{x}+{a}\right)={a}\left(\mathrm{3}{x}+{a}\right)^{\mathrm{2}} \\ $$
Answered by som(math1967) last updated on 28/Dec/21
![y^2 =4ax 2y(dy/dx)=4a [(dy/dx)]_(ap^2 ,2ap) =((4a)/(4ap))=(1/p) ∴slope of tanjent=(1/p) equation of tanjent (y−2ap)=(1/p)(x−ap^2 ) yp−2ap^2 =x−ap^2 x−yp+ap^2 =0 put x=−a [to find pt T] −a+ap^2 =yp y=((ap^2 −a)/p) ∴P(ap^2 ,2ap) T(−a,((ap^2 −a)/p)) ∴mid pt of PT x=((−a+ap^2 )/2) y=((2ap+((ap^2 −a)/p))/2)=((3ap^2 −a)/(2p)) ans i) x=((−a+ap^2 )/2)⇒p^2 =((2x+a)/a) y=((3ap^2 −a)/(2p)) 2yp=3ap^2 −a 4y^2 p^2 =(3ap^2 −a)^2 4y^2 (((2x+a)/a))=(((6ax+3a^2 −a^2 )/a))^2 4y^2 (2x+a)=(6ax+2a^2 )^2 ×(a/a^2 ) 4y^2 (2x+a)=((4a^2 (3x+a)^2 )/a) y^2 (2x+a)=a(3x+a)^2 y^2 (2x+a)=a(3x+a)^2 [proved]](Q162258.png)
$${y}^{\mathrm{2}} =\mathrm{4}{ax} \\ $$$$\mathrm{2}{y}\frac{{dy}}{{dx}}=\mathrm{4}{a} \\ $$$$\left[\frac{{dy}}{{dx}}\right]_{{ap}^{\mathrm{2}} ,\mathrm{2}{ap}} =\frac{\mathrm{4}{a}}{\mathrm{4}{ap}}=\frac{\mathrm{1}}{{p}} \\ $$$$\therefore{slope}\:{of}\:{tanjent}=\frac{\mathrm{1}}{{p}} \\ $$$${equation}\:{of}\:{tanjent}\: \\ $$$$\left({y}−\mathrm{2}{ap}\right)=\frac{\mathrm{1}}{{p}}\left({x}−{ap}^{\mathrm{2}} \right) \\ $$$${yp}−\mathrm{2}{ap}^{\mathrm{2}} ={x}−{ap}^{\mathrm{2}} \\ $$$${x}−{yp}+{ap}^{\mathrm{2}} =\mathrm{0} \\ $$$${put}\:{x}=−{a}\:\left[{to}\:{find}\:{pt}\:{T}\right] \\ $$$$−{a}+{ap}^{\mathrm{2}} ={yp} \\ $$$${y}=\frac{{ap}^{\mathrm{2}} −{a}}{{p}} \\ $$$$\therefore{P}\left({ap}^{\mathrm{2}} ,\mathrm{2}{ap}\right)\:\:\:\:{T}\left(−{a},\frac{{ap}^{\mathrm{2}} −{a}}{{p}}\right) \\ $$$$\therefore{mid}\:{pt}\:{of}\:{PT} \\ $$$$\:{x}=\frac{−{a}+{ap}^{\mathrm{2}} }{\mathrm{2}}\:\:{y}=\frac{\mathrm{2}{ap}+\frac{{ap}^{\mathrm{2}} −{a}}{{p}}}{\mathrm{2}}=\frac{\mathrm{3}{ap}^{\mathrm{2}} −{a}}{\mathrm{2}{p}} \\ $$$$\left.\:\:\:\:\:\:\:\:\:\:{ans}\:{i}\right) \\ $$$${x}=\frac{−{a}+{ap}^{\mathrm{2}} }{\mathrm{2}}\Rightarrow{p}^{\mathrm{2}} =\frac{\mathrm{2}{x}+{a}}{{a}} \\ $$$${y}=\frac{\mathrm{3}{ap}^{\mathrm{2}} −{a}}{\mathrm{2}{p}} \\ $$$$\mathrm{2}{yp}=\mathrm{3}{ap}^{\mathrm{2}} −{a} \\ $$$$\mathrm{4}{y}^{\mathrm{2}} {p}^{\mathrm{2}} =\left(\mathrm{3}{ap}^{\mathrm{2}} −{a}\right)^{\mathrm{2}} \\ $$$$\mathrm{4}{y}^{\mathrm{2}} \left(\frac{\mathrm{2}{x}+{a}}{{a}}\right)=\left(\frac{\mathrm{6}{ax}+\mathrm{3}{a}^{\mathrm{2}} −{a}^{\mathrm{2}} }{{a}}\right)^{\mathrm{2}} \\ $$$$\mathrm{4}{y}^{\mathrm{2}} \left(\mathrm{2}{x}+{a}\right)=\left(\mathrm{6}{ax}+\mathrm{2}{a}^{\mathrm{2}} \right)^{\mathrm{2}} ×\frac{{a}}{{a}^{\mathrm{2}} } \\ $$$$\mathrm{4}{y}^{\mathrm{2}} \left(\mathrm{2}{x}+{a}\right)=\frac{\mathrm{4}{a}^{\mathrm{2}} \left(\mathrm{3}{x}+{a}\right)^{\mathrm{2}} }{{a}} \\ $$$${y}^{\mathrm{2}} \left(\mathrm{2}{x}+{a}\right)={a}\left(\mathrm{3}{x}+{a}\right)^{\mathrm{2}} \\ $$$${y}^{\mathrm{2}} \left(\mathrm{2}{x}+{a}\right)={a}\left(\mathrm{3}{x}+{a}\right)^{\mathrm{2}} \:\:\:\left[{proved}\right] \\ $$
Commented by peter frank last updated on 29/Dec/21

$$\mathrm{great}\:\mathrm{sir} \\ $$
