Question and Answers Forum

All Questions      Topic List

Differentiation Questions

Previous in All Question      Next in All Question      

Previous in Differentiation      Next in Differentiation      

Question Number 162924 by mnjuly1970 last updated on 02/Jan/22

$$$$$$\mathit{\varphi }={\int }_0^{\mathrm{\infty }}\frac{e^{-x^2}.\ln \left(x\right)}{\sqrt{x}}dx=\lambda \mathrm{\Gamma }\left(\frac{1}{4}\right)$$$$$$$$\lambda =?◼$$$$$$

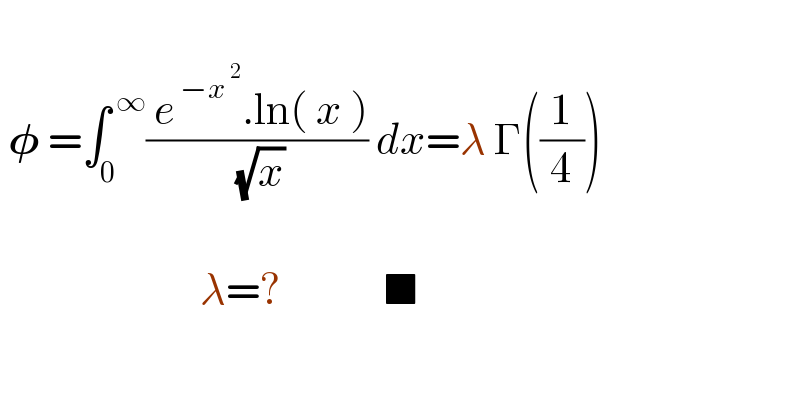
Answered by Lordose last updated on 02/Jan/22

$$\varnothing ={\int }_0^{\mathrm{\infty }}{\mathrm{x}}^{-\frac{1}{2}}{\mathrm{e}}^{-{\mathrm{x}}^2}\ln \left(\mathrm{x}\right)\mathrm{dx}$$$$\varnothing \stackrel{\mathrm{x}={\mathrm{x}}^{\frac{1}{2}}}{=}\frac{1}{4}{\int }_0^{\mathrm{\infty }}{\mathrm{x}}^{-\frac{1}{4}+\frac{1}{2}-1}{\mathrm{e}}^{-\mathrm{x}}\ln \left(\mathrm{x}\right)\mathrm{dx}$$$$\varnothing \left(\mathrm{a}\right)={\int }_0^{\mathrm{\infty }}{\mathrm{x}}^{\mathrm{a}-1}{\mathrm{e}}^{-\mathrm{x}}\mathrm{dx}=\mathbf{\Gamma }\left(\mathrm{a}\right)$$$${\varnothing }^{\prime }\left(\mathrm{a}\right)={\int }_0^{\mathrm{\infty }}{\mathrm{x}}^{\mathrm{a}-1}{\mathrm{e}}^{-\mathrm{x}}\ln \left(\mathrm{x}\right)\mathrm{dx}=\mathbf{\Gamma }\left(\mathrm{a}\right){\mathit{\psi }}^{\left(0\right)}\left(\mathrm{a}\right)$$$$\varnothing ={\varnothing }^{\prime }\left(\frac{1}{4}\right)=\mathbf{\Gamma }\left(\frac{1}{4}\right){\mathit{\psi }}^{\left(0\right)}\left(\frac{1}{4}\right)$$$$\lambda ={\mathit{\psi }}^{\left(0\right)}\left(\frac{1}{4}\right)$$

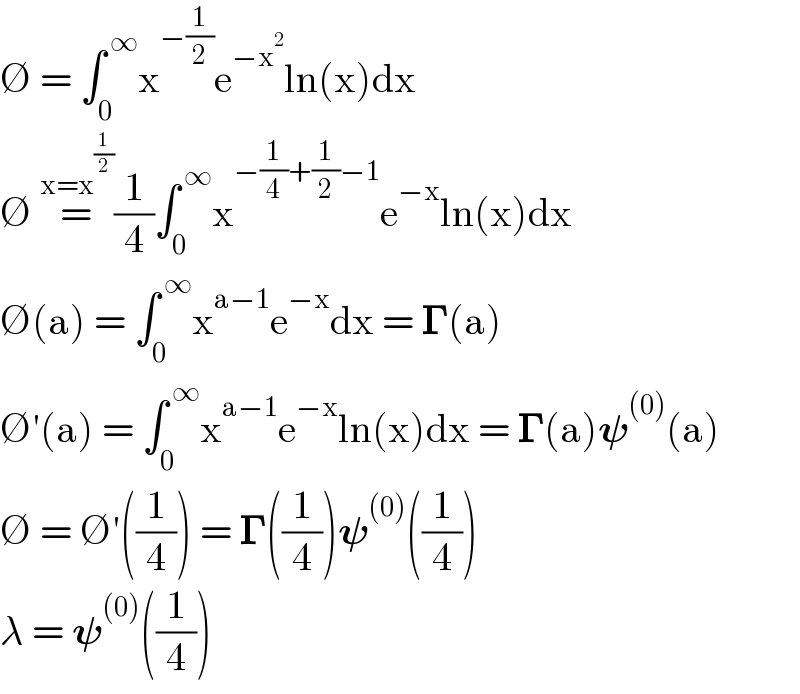
Commented by mnjuly1970 last updated on 02/Jan/22

$$thanksalotsirlordose$$

Answered by mathmax by abdo last updated on 02/Jan/22

$$\mathrm{\Psi }={\int }_0^{\mathrm{\infty }}\frac{{\mathrm{e}}^{-{\mathrm{x}}^2}\mathrm{lnx}}{\sqrt{\mathrm{x}}}\mathrm{dx}\Rightarrow \mathrm{\Psi }{=}_{\mathrm{x}=\sqrt{\mathrm{t}}}{\int }_0^{\mathrm{\infty }}\frac{{\mathrm{e}}^{-\mathrm{t}}\ln \left(\sqrt{\mathrm{t}}\right)}{{\mathrm{t}}^{\frac{1}{4}}}\frac{1}{2}{\mathrm{t}}^{-\frac{1}{2}}\mathrm{dt}$$$$=\frac{1}{4}{\int }_0^{\mathrm{\infty }}{\mathrm{e}}^{-\mathrm{t}}\mathrm{lnt}{\mathrm{t}}^{-\frac{3}{4}}\mathrm{dt}=\frac{1}{4}{\int }_0^{\mathrm{\infty }}{\mathrm{t}}^{\frac{1}{4}-1}{\mathrm{e}}^{-\mathrm{t}}\ln \left(\mathrm{t}\right)\mathrm{dt}$$$$\mathrm{let}\mathrm{\Gamma }\left(\mathrm{a}\right)={\int }_0^{\mathrm{\infty }}{\mathrm{t}}^{\mathrm{a}-1}{\mathrm{e}}^{-\mathrm{t}}\mathrm{dt}\Rightarrow {\mathrm{\Gamma }}^{\left(1\right)}\left(\mathrm{a}\right)={\int }_0^{\mathrm{\infty }}{\mathrm{t}}^{\mathrm{a}-1}\mathrm{lnt}{\mathrm{e}}^{-\mathrm{t}}\mathrm{dt}\Rightarrow$$$${\mathrm{\Gamma }}^{{}^{\prime }}\left(\frac{1}{4}\right)={\int }_0^{\mathrm{\infty }}{\mathrm{t}}^{\frac{1}{4}-1}{\mathrm{e}}^{-\mathrm{t}}\ln \left(\mathrm{t}\right)\mathrm{dt}=\psi \left(\frac{1}{4}\right)\Rightarrow$$$${\int }_0^{\mathrm{\infty }}\frac{{\mathrm{e}}^{-{\mathrm{x}}^2}\mathrm{lnx}}{\sqrt{\mathrm{x}}}\mathrm{dx}=\frac{1}{4}\psi \left(\frac{1}{4}\right)$$

Commented by mathmax by abdo last updated on 03/Jan/22

$$\mathrm{sorry}\frac{{\mathrm{\Gamma }}^{{}^{\prime }}\left(\frac{1}{4}\right)}{\mathrm{\Gamma }\left(\frac{1}{4}\right)}=\psi \left(\frac{1}{4}\right)\Rightarrow {\mathrm{\Gamma }}^{{}^{\prime }}\left(\frac{1}{4}\right)=\psi \left(\frac{1}{4}\right).\mathrm{\Gamma }\left(\frac{1}{4}\right)$$

Terms of Service

Privacy Policy

Contact: info@tinkutara.com