Question Number 163280 by mr W last updated on 05/Jan/22
Commented by mr W last updated on 05/Jan/22
$${maybe}\:{impossible}\:{to}\:{solve}: \\ $$$${find}\:{the}\:{radius}\:{of}\:{the}\:{n}^{{th}} \:{circle}. \\ $$
Commented by aleks041103 last updated on 05/Jan/22
$${I}'{ll}\:{try}... \\ $$
Commented by behi834171 last updated on 07/Jan/22
$$\:\:\:\:\:\:\:\boldsymbol{{r}}_{\boldsymbol{{n}}} =\frac{\mathrm{1}}{\mathrm{2}^{\left(\boldsymbol{{n}}−\mathrm{1}\right)} }\left(\mathrm{2}−\sqrt{\mathrm{2}}\right)^{\boldsymbol{{n}}} \left(\mathrm{2}−\sqrt{\mathrm{2}−\sqrt{\mathrm{2}}}\right)^{\left(\boldsymbol{{n}}−\mathrm{1}\right)} \:\:. \\ $$$${please}\:{see}\:{q}#\mathrm{10455} \\ $$
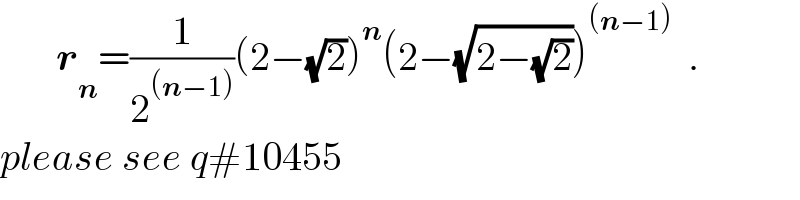
Commented by mr W last updated on 07/Jan/22
$${thanks}\:{sir}! \\ $$$${yes}.\:{i}\:{remember}\:{this}\:{question}\:{was} \\ $$$${once}\:{discussed}. \\ $$$${but}\:{the}\:{formula}\:{you}\:{mentioned}\:{is} \\ $$$${not}\:{correct}.\:{only}\:{r}_{\mathrm{1}} \:{is}\:{correct}.\:{upon} \\ $$$${n}=\mathrm{2},\:{it}\:{is}\:{not}\:{correct},\:{because}\:{the} \\ $$$${circles}\:{don}'{t}\:{tangent}\:{with}\:{the}\:{curve} \\ $$$${y}=\frac{\mathrm{1}}{{x}},\:{see}\:{diagram}. \\ $$$${i}\:{think}\:{it}'{s}\:{impossible}\:{to}\:{find}\:{a} \\ $$$${formula}\:{for}\:{the}\:{radius}\:{of}\:{n}^{{th}} \:{circle} \\ $$$${in}\:{terms}\:{of}\:{n}. \\ $$
Commented by mr W last updated on 08/Jan/22
Answered by ajfour last updated on 06/Jan/22
Commented by ajfour last updated on 08/Jan/22
![y^2 =x^2 +2 y=(√(x^2 +2)) (dy/dx)=(x/( (√(x^2 +2))))= a(√2)+a=(√2) ⇒ a=2−(√2) A[0,2(√2)−2] let B[(a+b)sin θ, a(√2)+(a+b)cos θ] b=((a(√2)+(a+b)(cos θ−sin θ))/( (√2))) let Circle B touches y=(√(x^2 +2)) at T_2 [p,(√(p^2 +2))] a(√2)+(a+b)cos θ+bsin φ=(√(p^2 +2)) (a+b)sin θ−bcos φ=p subtracting ⇒ a(√2)+(√2)(b−a)+b(sin φ+cos φ) =(√(p^2 +2))−p .....(i) tan φ=((sin φ)/(cos φ))=(p/( (√(p^2 +2)))) ⇒ p^2 =(2/(cot^2 φ−1)) ...(ii) and (p+bcos φ)^2 +((√(p^2 +2))−a(√2)−bsin φ)^2 =(a+b)^2 ⇒ p^2 +b^2 +2bpcos φ +((√(p^2 +2))−a(√2))^2 −2b((√(p^2 +2))−a(√2))sin φ=(a+b)^2 ......(iii) three eqns. unknowns: b,p,tan φ](Q163602.png)
$${y}^{\mathrm{2}} ={x}^{\mathrm{2}} +\mathrm{2} \\ $$$${y}=\sqrt{{x}^{\mathrm{2}} +\mathrm{2}} \\ $$$$\frac{{dy}}{{dx}}=\frac{{x}}{\:\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}}= \\ $$$${a}\sqrt{\mathrm{2}}+{a}=\sqrt{\mathrm{2}}\:\:\Rightarrow\:\:{a}=\mathrm{2}−\sqrt{\mathrm{2}} \\ $$$${A}\left[\mathrm{0},\mathrm{2}\sqrt{\mathrm{2}}−\mathrm{2}\right] \\ $$$${let}\:\:\:{B}\left[\left({a}+{b}\right)\mathrm{sin}\:\theta,\:{a}\sqrt{\mathrm{2}}+\left({a}+{b}\right)\mathrm{cos}\:\theta\right] \\ $$$${b}=\frac{{a}\sqrt{\mathrm{2}}+\left({a}+{b}\right)\left(\mathrm{cos}\:\theta−\mathrm{sin}\:\theta\right)}{\:\sqrt{\mathrm{2}}} \\ $$$${let}\:{Circle}\:{B}\:{touches}\:{y}=\sqrt{{x}^{\mathrm{2}} +\mathrm{2}}\:\:\:{at} \\ $$$${T}_{\mathrm{2}} \left[{p},\sqrt{{p}^{\mathrm{2}} +\mathrm{2}}\right] \\ $$$${a}\sqrt{\mathrm{2}}+\left({a}+{b}\right)\mathrm{cos}\:\theta+{b}\mathrm{sin}\:\phi=\sqrt{{p}^{\mathrm{2}} +\mathrm{2}} \\ $$$$\left({a}+{b}\right)\mathrm{sin}\:\theta−{b}\mathrm{cos}\:\phi={p} \\ $$$${subtracting} \\ $$$$\Rightarrow\:\:{a}\sqrt{\mathrm{2}}+\sqrt{\mathrm{2}}\left({b}−{a}\right)+{b}\left(\mathrm{sin}\:\phi+\mathrm{cos}\:\phi\right) \\ $$$$\:\:\:\:\:\:\:\:=\sqrt{{p}^{\mathrm{2}} +\mathrm{2}}−{p}\:\:\:\:\:.....\left({i}\right) \\ $$$$\mathrm{tan}\:\phi=\frac{\mathrm{sin}\:\phi}{\mathrm{cos}\:\phi}=\frac{{p}}{\:\sqrt{{p}^{\mathrm{2}} +\mathrm{2}}} \\ $$$$\Rightarrow\:\:{p}^{\mathrm{2}} =\frac{\mathrm{2}}{\mathrm{cot}\:^{\mathrm{2}} \phi−\mathrm{1}}\:\:\:...\left({ii}\right) \\ $$$${and}\:\:\:\left({p}+{b}\mathrm{cos}\:\phi\right)^{\mathrm{2}} +\left(\sqrt{{p}^{\mathrm{2}} +\mathrm{2}}−{a}\sqrt{\mathrm{2}}−{b}\mathrm{sin}\:\phi\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:=\left({a}+{b}\right)^{\mathrm{2}} \\ $$$$ \\ $$$$\Rightarrow\:\:\:{p}^{\mathrm{2}} +{b}^{\mathrm{2}} +\mathrm{2}{bp}\mathrm{cos}\:\phi \\ $$$$+\left(\sqrt{{p}^{\mathrm{2}} +\mathrm{2}}−{a}\sqrt{\mathrm{2}}\right)^{\mathrm{2}} \\ $$$$−\mathrm{2}{b}\left(\sqrt{{p}^{\mathrm{2}} +\mathrm{2}}−{a}\sqrt{\mathrm{2}}\right)\mathrm{sin}\:\phi=\left({a}+{b}\right)^{\mathrm{2}} \\ $$$$\:\:\:\:\:\:......\left({iii}\right) \\ $$$${three}\:{eqns}.\:\:\:{unknowns}:\:\:{b},{p},\mathrm{tan}\:\phi \\ $$